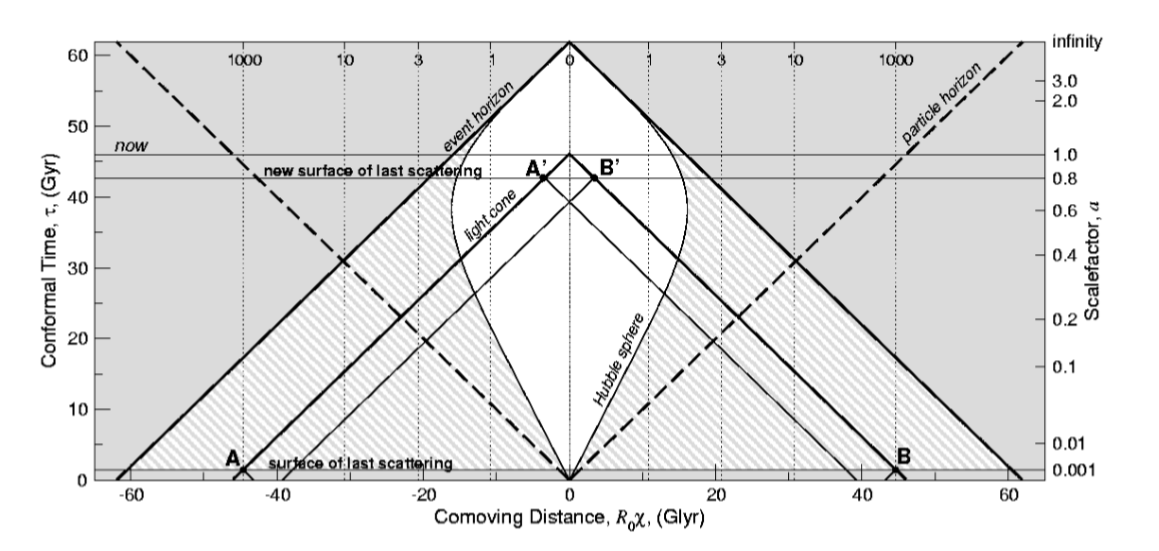
Imagine two points, A and B, that are presently at opposite locations on the last scattering sphere. Light emitted from A and from B has only just now reached Earth, and since the proper distance between A and B is twice the last scattering distance, they have never exchanged light signals. In other words, their light cones when projected from the last scattering surface to $\tau = 0$, do not intersect, as shown in the figure. And, yet, all such points on the last scattering sphere are strikingly uniform, suggesting that they were in equilibrium by the time of last scattering. As there is not causal mechanism which could have brought about this equilibrium, this is considered a problem -- the horizon problem.
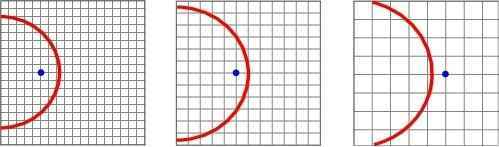
Inflation addresses this problem by providing such a mechanism. That the proper distance to the event horizon is shrinking in comoving coordinates is just another way of saying that space is expanding at a greater rate than the horizon is. Consider again points A and B, but now suppose that they have had time to exchange light signals before inflation begins, i.e. they are within each other's particle horizon's at the start of inflation. During inflation, since space expands at a greater rate than the horizon, points A and B will be pulled outside of each other's event horizons (as well as Earth's horizon). See the following sequence illustrating how a single point can be pulled outside an observer's horizon on account of the inflating space.
Now, when inflation ends, points A and B will appear to subtend an acausal distance on the last scattering sphere, but in reality they were once in causal contact. This has the effect of moving the last scattering surface ahead in time such that their lightcones indeed intersect at $\tau = 0$, i.e. that they have time to exchange light signals before last scattering.