It is a solid volume, but we can't see most of it.
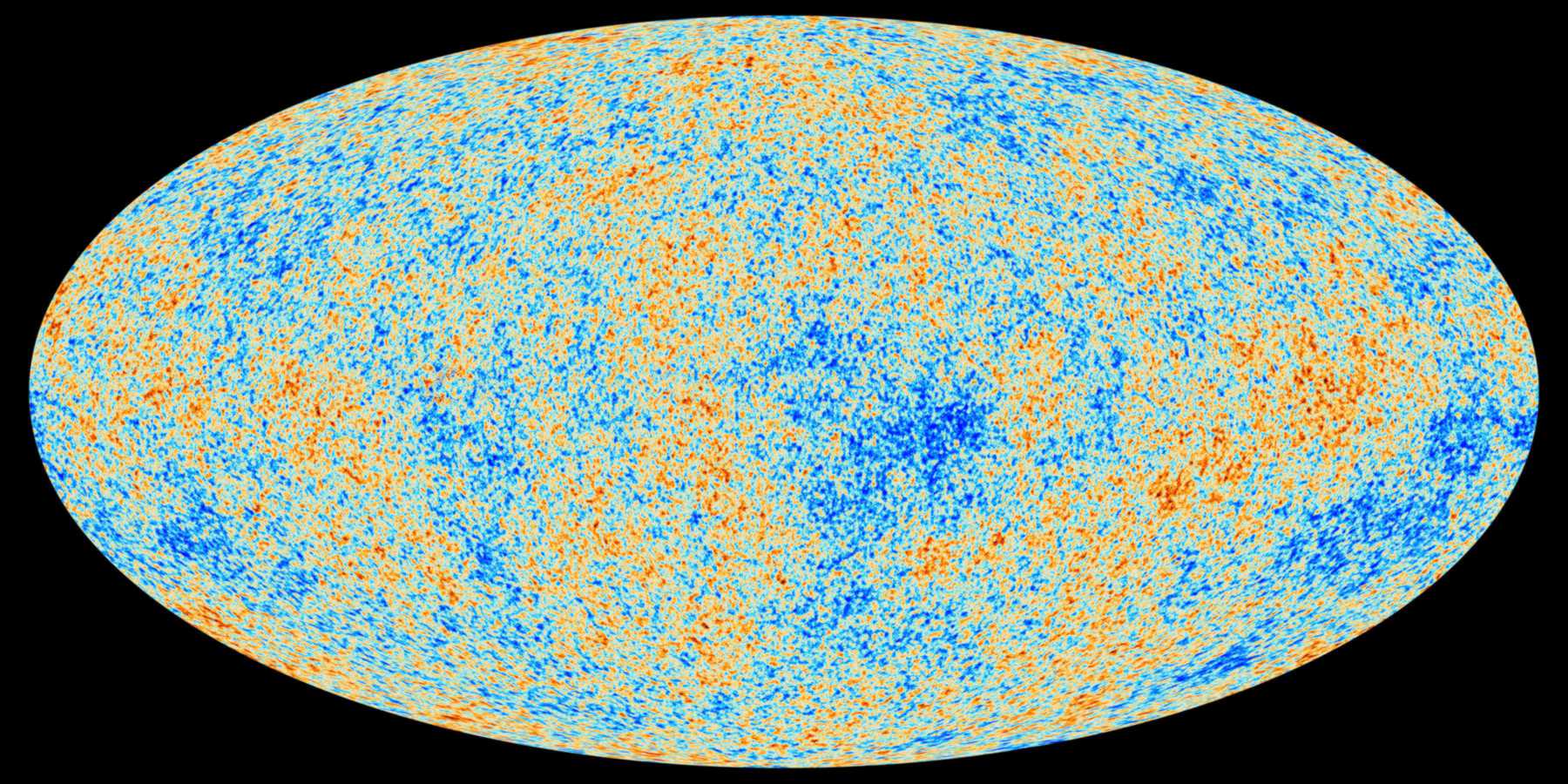
The plasma that emitted the CMBR is/was located on a spacelike 3D surface. Everything that we can see lies on the boundary of our past light cone, which is a 3D null surface. The only part of the CMBR that we can see is the intersection of those two surfaces, which is a 2-sphere.
(Actually, the CMBR surface has a nonzero thickness because the plasma has an optical depth, and the light cone has a nonzero thickness because the universe isn't a perfect vacuum, so the intersection is a "thick sphere". I don't know the thickness, but this paper, if I'm interpreting it correctly, estimates it at ~19 Mpc in present-day comoving distance, or ~0.1% of the 14 Gpc radius of the sphere. This is comparable to the angular resolution of Planck, so Planck's CMBR map may be the best we'll get for the next ~19 Mpc/c.)
A civilization at a different location in spacetime will see a different spherical slice of the CMBR. If they're close enough to us, the slices will have a circle in common. This is the intuition behind Cornish et al's search for correlated circles.