Create a square well potential for an electron in practice

Question

If my particle is an electron, how do I create a square well potential (in one dimension) in practice? I would like to know how to actually achieve it. Is it like at both the ends, I need to put some bunch of electrons to repel the electron in the middle or something like that?

Answer 1

Square well potentials are analytically convenient in educational demonstrations of quantum mechanics. In practice one zero dimensional realisation is the so called quantum dot, which is applied in QLED screens. Another is the Penning trap. A realisation of a 1D quantum well is called a quantum wire. An example is the carbon nanotube.

Answer 2

I have recently answered a question about this topic (here: Specific experiments that affirm particle-in-a-box QM theory), but I will reiterate here that even though the “particle in a box” infinite well potential is not physically real, it can be used to crudely model the electrons in the $\pi$ system of conjugated polyenes like $\beta$ carotene. This is because the electrons in the $\pi$ system are essentially confined to a “linear” region along the spine of the molecule. To improve the model, we could make the potential well finite to add a dissociation limit, and we could further add a softer potential edge, among other options. This is not the only application of the PIAB potential, of course, but it is one of particular interest to physical chemists and chemical physicists.

Answer 3

Square wells are fundamentally discontinuous and un-physical potentials. They're meant as toys to visualize behavior, so there's no good way to physically construct them. However, some reasonably similar situations can be made, though I'm not sure why you'd want to do that.

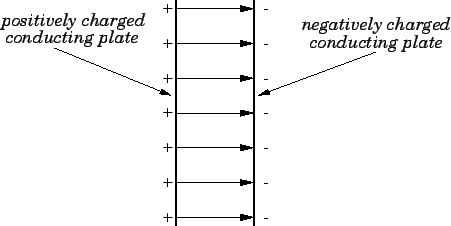
For an electron, you could, for instance, take 2 capacitor plates, such that the field is zero outside and some constant value inside., like this:

But if you look closer, you'll see that there need to be some charges deposited at finite distances from each other on each of those surfaces, and it'll be something like this:

_(source)

And around each of those charges there's a nice, continuous field, and only once you add all those individual fields will you get those equipotential surfaces parallel to the walls of the well.