Is cosmic inflation slow rather than fast?

Question

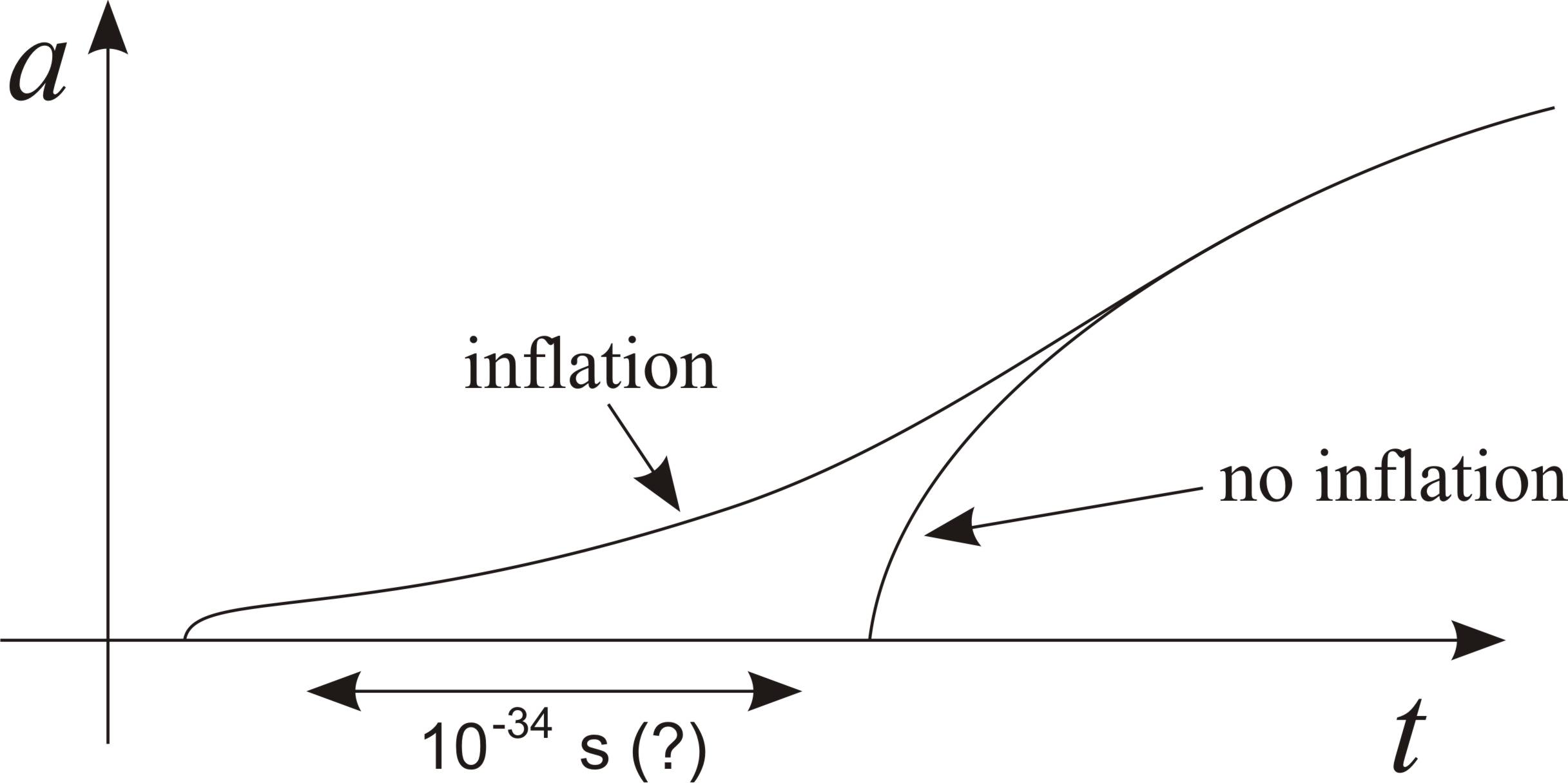
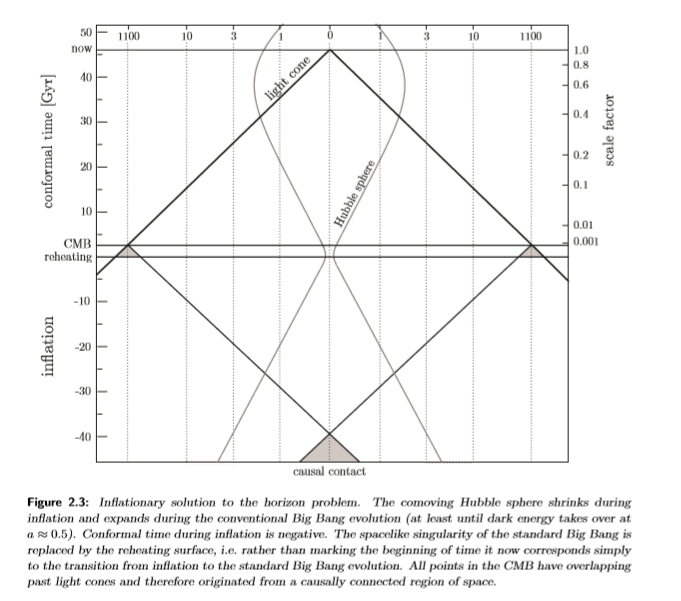
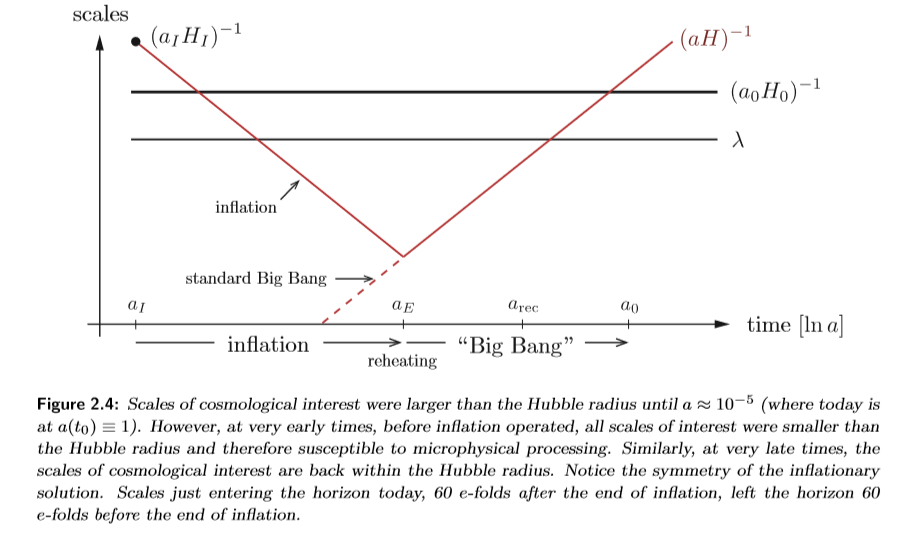
According to cosmic inflation models, there is an early period where $\Lambda$ dominates and the scale factor grows exponentially. Among other things, this helps to give a reason for large-scale homogeneity (i.e. 'solves' horizon problem), because the parts of the universe that are causally connected become much larger. So far so good.

It seems to me that the reason the inflation picture achieves this causal connection is because it asserts that the growth of the scale factor during this very early period is very much slower than it would be on another model such as Friedman model without $\Lambda$.

(Vertical scale on this diagram is off of course!) Because the growth is slow, there is time for light-speed-limited communication around larger parts of the universe, because they are not being carried away from each other. But when you read presentations of inflation, you very commonly see a phrase such as "inflation solves X because the universe went through a period of extremely fast exponential growth", with the emphasis on fast. Now I don't deny that these early processes were fast, but surely the whole point about inflation is that it makes them slower not faster?

I understand that this is a process that is very fast compared to everyday timescales, but as far as I can see, the reason it solves the horizon problem, if it does, is because it makes the early expansion of the universe extremely slow compared to what one might otherwise expect, and what was in fact thought.

My question is: is that right or am I misunderstanding something?

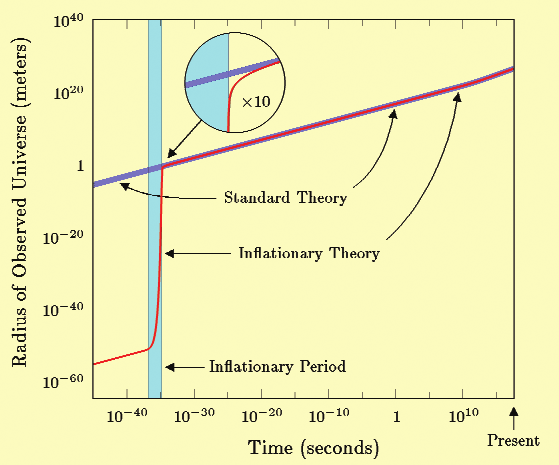
(On the widely-used illustration of cosmic history from NASA/WMAP Science Team (e.g. at https://en.wikipedia.org/wiki/Chronology_of_the_universe) there appears to have been an effort to show a sharper growth in the early part, marked inflation, whereas I think a correct graph would have a point of inflection and look more like the one I drew above.)

This is similar to an earlier (unanswered) question, but perhaps I may have asked it more clearly.

Answer 1

Introduction

As always in GR, there are different ways you can slice and dice a metric, and loosely using ordinary words like "fast" and "slow" will lead to confusion when different people inevitably use different coordinates or ways of describing the spacetime without being explicit. This is even more so when comparing two different spacetimes, where the choice of how to match the spacetimes affects the words used to describe the situation.

In support of "slow" inflation

Your figure implicitly points out two related facts about inflation.

• Relative to a radiation dominated universe, if we fix the late time expansion to be the same, a Universe with inflation can be much older.
• The proper distance between two points (evolving backward in time) will shrink to zero much more slowly in a Universe with inflation than a radiation-dominated Universe.

These are correct statements, and from this point of view it certainly makes sense to describe inflation as "slow." Indeed there are other facts that support this point of view, for example

• de Sitter spacetime is maximally symmetric, so in some sense during inflation, when the Universe is (almost) a de Sitter spacetime, the Universe is (almost) not evolving.
• The inflaton field drives inflation, and serves as the "clock" in the sense that the evolution of the field is a natural time coordinate, and eventually the field hits the end of the inflating part of the potential and tells inflation when to stop. The condition for inflation to occur is that the field obeys slow-roll conditions.

In support of "fast" inflation

Having said all of that, there is a definite sense in which inflation can be considered rapid expansion. The intuitive picture is that two nearby points in the early Universe, were driven by inflation to be a huge distance apart, compared to the horizon size of the Universe. This effect does not happen during a radiation dominated era.

As implied by the words, in this picture we are actually comparing two different length scales that can be defined within one expanding Universe, rather than comparing an "absolute" scale like proper distance or age between two different Universes. In particular, we are comparing (at some finite time $t$ after the big bang)

1. the proper distance between two points, and
2. the horizon size of the Universe (also called the particle horizon or cosmological horizon or comoving horizon), or the maximum distance from which light could have reached us.

The key idea is that during inflation, the proper distance grows much more rapidly than the horizon size, while the proper distance grows more slowly than the horizon size in a radiation dominated Universe. In "ordinary" FLRW coordinates:

• During inflation, the horizon size is constant while the proper distance grows exponentially.
• During radiation domination, the horizon size grows as $t$, while the proper distance grows as $\sqrt{t}$.

From this point of view, the key of inflation is that the separation between particles grew exponentially, relative to the natural length scale set by the Universe's expansion rate.

Summary

Of course, these different pictures are just different words that are draped around actual calculations using GR. There is not a unique "right" way to look at it$^\star$, and the different pictures are useful to emphasize different points in different situations. The "fast inflation" point of view makes it clear why inflation solves the horizon problem, which is perhaps why inflation is commonly described in these terms, despite the perfectly valid "slow inflation" point of view.

---

$^\star$ Originally I wrote "no right or wrong way to look at it", but actually there are definitely wrong ways to look at it.

Answer 2

Yes, inflation is slower. During inflation, $a$ increases by a factor of $e^{60}$ in around $10^{-33}\text{ s}$, but in the traditional radiation-dominated big bang (the "no inflation" curve in your image), $a$ increases by a factor of $\infty$ in around $10^{-35}\text{ s}$.

It's necessary for inflation to last long enough for there to be significant causal contact between opposite ends of the CMB sphere, but it's not sufficient: you need an expanding, critical-density hot big bang at the end, not just a homogeneous region. So I don't think it's correct to say that the "whole point" of inflation is that it's slow, but it does need to be slow (relatively speaking).

The belief that inflation is faster than the traditional big bang is probably reinforced by diagrams like the one below, which is from Inflation and the New Era of High-Precision Cosmology by Guth, and is also found in his popular book "The Inflationary Universe".

You can find many similar diagrams on the web, perhaps inspired by Guth's, and I suspect that it also inspired the shape of the universe in the NASA/WMAP image that you mentioned in the question, if you were talking about this one:

I can't just dismiss the diagram as wrong, since it was endorsed by Guth and probably made by him, so what's going on? I think the answer is that the diagram uses different origins of time for the two models, i.e., the cosmological time coordinates don't quite match in the post-inflationary epoch where the models are identical. The discrepancy is only about $10^{-35}\text{ s}$, but it makes a big difference when $t$ is equally small. If you shift the blue curve to align it with the red curve, you get this:

The red curve is made of square root, exponential, and square root curves grafted together as in the image in the question, but plotted on a log-log scale. It matches Guth's curve almost perfectly. The blue curve looks like two hand-drawn straight lines, but it's actually an accurate plot of a square-root curve on a log-log chart whose origin is slightly misaligned with it.

If you shift the red curve to align with the blue curve instead, you get this:

Most of the inflationary epoch is invisible on this graph because it happens at $t<0$.

I think the moral is that you shouldn't make log plots of cosmological time, because it has no natural zero point. I've never seen $\log t$ used as a coordinate in technical introductions to inflation, only in popularizations.

Answer 3

I understand that this is a process that is very fast compared to everyday timescales, but as far as I can see, the reason it solves the horizon problem, if it does, is because it makes the early expansion of the universe extremely slow compared to what one might otherwise expect, and what was in fact thought.

Well yes. In simple terms, The inflation model creates more conformal time due to the huge increase in the scale factor. So with the help of inflation, there is "enough time" to create a heat equilibrium between two antipodal points. Without inflation, there's "not enough time" to create this equilibrium.

But why inflation creates more time?

The reason is that fast change in the scale factor. Since at the beginning the scale factor was too small but then increases fast so when we take the integral to calculate the conformal time we get a large value.

$$\eta=\int d\tau /a(t)$$

or

$$d\eta/d\tau=1/a(t)$$

Source:http://www.damtp.cam.ac.uk/user/db275/Cosmology/Lectures.pdf

As I said before. The inflation creates more conformal time. You may refer is as "slow". But the proper time is in short period of time.

From the book of Andrew Riddle, Cosmological Inflation and Large Scale Structure

The point is theres difference between conformal time and proper time. Maybe this creates confusion.

Also you can read the article in the link (Chapter 2)