Green's Function Method for a Spring and mass system [closed]

Question

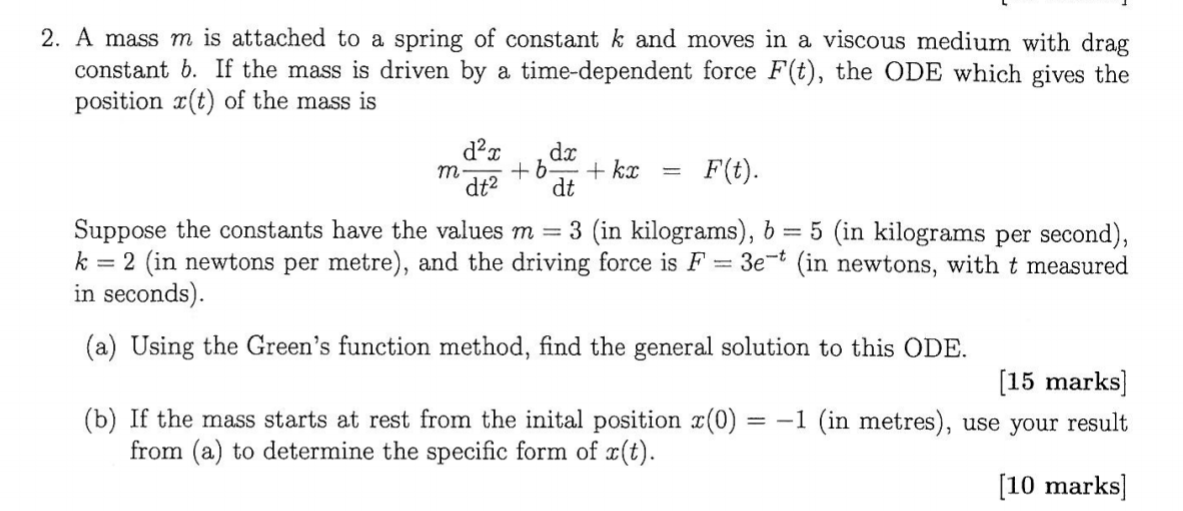
I think I've done part a) correctly and I have a general solution. However, I now have two unknown constants in my general solution and, as far as I can see, only one condition ($x(0)=-1$) with which to find these constants and provide the specific form required. Can anyone explain to me where a second condition is implied/found or have I done something wrong in answering part a)?

My answer for part a) was: \begin{align} x(t) &= 9(\exp(-t/3) - \exp(-2t/3)) \\ &- (3/2)(\exp(t) + \exp(-t) + A(\exp(-2t/3)) \\ &+ B\exp(-t) \end{align}

where $A$ and $B$ are constants, applying the initial condition $x(0)= -1$ I got $A+B=2$.

Answer 1

First, I think you don't quite have part a correct, but this just might be a typo. My particular solution from the usual retarded Green's function is \begin{equation} x_p(t) = 9e^{-2t/3}-(9+3t)e^{-t} \end{equation} and adding the homogeneous solution \begin{equation} x_0(t) = Ae^{-2t/3}-Be^{-t} \end{equation} as you have, gives part a.

In part b the boundary conditions are $x(0)=-1$ and the particle is at rest, so $\frac{dx(t)}{dt}$ is zero at $t=0$, and you have the two conditions needed to solve for $A$ and $B$.