3
$\begingroup$

Sum-frequency generation sum-frequency generation 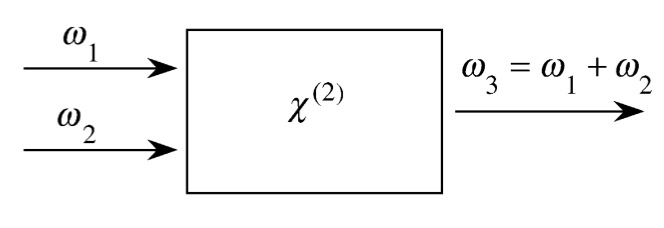is easy for my to understand. Two photons combine their energies in a nonlinear medium and out comes one photon with the sum of both energies.

But Difference-frequency generation difference-frequency generation 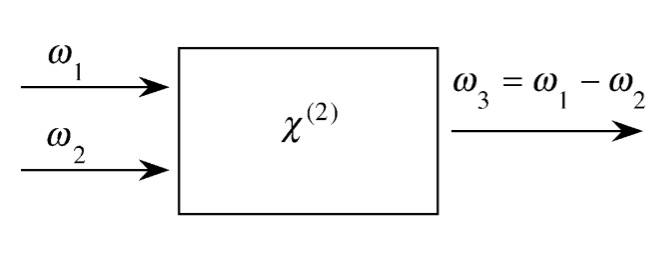is harder for me. For one thing, I'm struck by where the extra energy went? Is it heating up the nonlinear medium?

Is there a way for me to visualize this process that is similar to my understanding of the case of Sum-frequency generation?

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
1
$\begingroup$

The DFG component in the wave equation is proportional to

$$E_{1}E_{2}^{\ast}e^{-i(\omega_{1}-\omega_{2})}$$

Interpretating the fields as quantum entities, one takes $E_{1}\propto a_{1}$ and $E_{2}^{\ast}\propto a_{2}^{\dagger}$. Thus the above term is

$$a_{1}a_{2}^{\dagger}e^{-i(\omega_{1}-\omega_{2})}$$

You can interpret this contribution as the destruction of one $\omega_{1}$ photon and creation of both $\omega_{2}$ and $\omega_{1}-\omega_{2}$ photons. The creation of the first one is stimulated by an input beam of the same frequency. You can clearly see that conservation of energy works.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I see. So to write a balanced equation, the diagram should show one omega3 and one omega2 photon coming out of the non-linear medium. $\endgroup$
    – psitae
    Commented Jan 20, 2019 at 6:19
  • $\begingroup$ @psitae Two $\omega_{2}$. The book just focuses on the interesting beam, discarding all the others. $\endgroup$
    – eranreches
    Commented Jan 20, 2019 at 22:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.