0
$\begingroup$

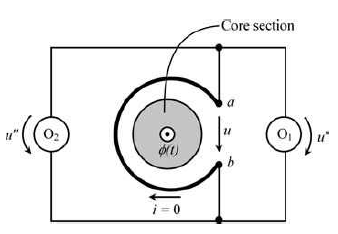
I'm currently studying induction law and circuits with inductors. I came however with the following circuit:

enter image description here

Now my text says the following:

"Unlike what your intuition might tell you, oscilloscopes 1 and 2 will measure different voltages. For a N-turn coil you will get

$$u' = N\frac{d\phi}{dt}$$ $$u'' = (N-1)\frac{d\phi}{dt}$$ "

How is that possible? I'm having trouble on understanding why this results are obtained. I understand (I think) that the oscilloscopes will measure different values because the induction E field is non conservative. But I don't understand their computation. How does the left side sees one coil less than the right side?

EDIT: Some additional information: At bold we have a ferromagnetic core that is excited by a sinusoidal current flowing in an inductor (not shown) which gives rise to a magnetic flux in the core. A N-turn coil is wound around the core. The coil is left open (i=0), but due to induction phenomena a voltage u(t) appears across its terminals a and b. In order to visualize the coil voltage, two oscilloscopes O1 and O2 are connected between a and b.

Improve this question
$\endgroup$
3
  • $\begingroup$ It's not quite clear to me, from the drawing, where the N-turn coil is. Is the bolded path from a to b (somehow) the coil (and the flux $\phi(t)$ generated somehow)? Or is the coil wrapped around the core section and the current through generated somehow? $\endgroup$
    – Alfred Centauri
    Commented Oct 30, 2018 at 0:44
  • 1
    $\begingroup$ @Alfred I'm going to add some information $\endgroup$
    – Granger Obliviate
    Commented Oct 30, 2018 at 0:46
  • $\begingroup$ @Alfred Just added some additional detail about the figure :) $\endgroup$
    – Granger Obliviate
    Commented Oct 30, 2018 at 0:48

2 Answers 2

Reset to default
0
$\begingroup$

But I don't understand their computation. How does the left side sees one coil less than the right side?

The emf is induced into wires connecting oscilloscopes with the coil. You have to figure why these contributions are different for the two oscilloscopes.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Exactly but that's exactly what I'm not figuring out. How is it just one coil that is not contributing to u''? $\endgroup$
    – Granger Obliviate
    Commented Oct 30, 2018 at 0:49
  • $\begingroup$ @GrangerObliviate What about the direction of the induced emf? $\endgroup$
    – V.F.
    Commented Oct 30, 2018 at 0:50
  • $\begingroup$ @GrangerObliviate The wires connected to the left oscilloscope go around the core, the wires connected to the right oscilloscope - don't. $\endgroup$
    – V.F.
    Commented Oct 30, 2018 at 0:53
  • $\begingroup$ It's clockwise following the path of the N coils right? Like I have no trouble understading that $$u' = N\frac{d\phi}{dt}$$. since u'=u and u is obtained from the induction law $$u = N\frac{d\phi}{dt}$$. u'' is what's giving me trouble. $\endgroup$
    – Granger Obliviate
    Commented Oct 30, 2018 at 0:54
  • $\begingroup$ I can see that but I don't see how that implies $$u'' = (N-1)\frac{d\phi}{dt}$$ " $\endgroup$
    – Granger Obliviate
    Commented Oct 30, 2018 at 0:55
0
$\begingroup$

How does the left side sees one coil less than the right side?

I think one way to approach this is to consider the case that the bolded wire from $a$ to $b$ is just that - a wire from $a$ to $b$ that doesn't wind around the core multiple times. Let's say that this is the $N = 1$ case.

Now, consider the path from the top of $O_2$ clockwise to $a$ then 'round the core to $b$ and then to the bottom of $O_2$. This loop is not threaded by the flux $\phi(t)$ (the closed path does not enclose the core section).

Now, consider the path from the top of $O_1$ counterclockwise to $a$ then 'round the core to $b$ and then to the bottom of $O_1$. This loop is threaded by the flux $\phi(t)$ (the closed path does enclose the core section).

It follows that $u' = 1\cdot\frac{d\phi}{dt}$ (flux threads one loop) and $u'' = 0\cdot\frac{d\phi}{dt}$ (flux threads zero loops).

From this, deduce that the general result for $N > 1$.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Hello Alfred! I completely understood this for the case N=1. Thank you. Now what it's difficult in my mind to accept is how if I have imagine 2 coils if I consider the path with O2 I traverse one of the coils (so $$u'' = 1 \frac{d\phi}{dt}$$) I just can't see it in my head? Why is it not zero again? $\endgroup$
    – Granger Obliviate
    Commented Oct 30, 2018 at 1:12
  • $\begingroup$ @GrangerObliviate, I'm afraid I don't understand why it isn't clear. If the wire from $a$ wraps around the core once before connecting to $b$, then the $O_2$ path encloses the flux once. Why would $u''$ be zero in this case? $\endgroup$
    – Alfred Centauri
    Commented Oct 30, 2018 at 2:31

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.