A colleague of mine (a physicist) recently claimed that it is possible to calculate the distance to a rainbow by applying the parallax method and that the result would be ~150 million kilometers, the earth-sun distance. To me this statement seems quite odd, since to my knowledge, you need some fixed, far distant background for calculating the parallax angle. Can anyone explain how this is possible or is it simply false?
-
3$\begingroup$ A rainbow has no 'shape' the arc is just the refraction of water droplets (that can be at different distances) at some angle. Therefore you cannot calculate the distance to a rainbow other than: the rainbow is where the rain/droplets are (atoptics.co.uk/fz439.htm). $\endgroup$– user190081Commented Oct 24, 2018 at 19:59
-
1$\begingroup$ @user190081, I can define the shape of a rainbow. It is a volume bounded by two cones. The vertices of both cones are at your eye, and the axis of both cones points from your eye in the direction opposite the direction of the Sun. $\endgroup$– Solomon SlowCommented Oct 24, 2018 at 21:04
-
1$\begingroup$ @SolomonSlow No objections. $\endgroup$– user190081Commented Oct 24, 2018 at 21:21
-
$\begingroup$ Rainbows occur at a very particular angle for sunlight reflecting off the back surface of rain drops. As you move towards a rainbow, it moves away from you to preserve this angle. This means that in a sense, there is no "distance" to a rainbow. $\endgroup$– David WhiteCommented Jul 11, 2020 at 22:37
3 Answers
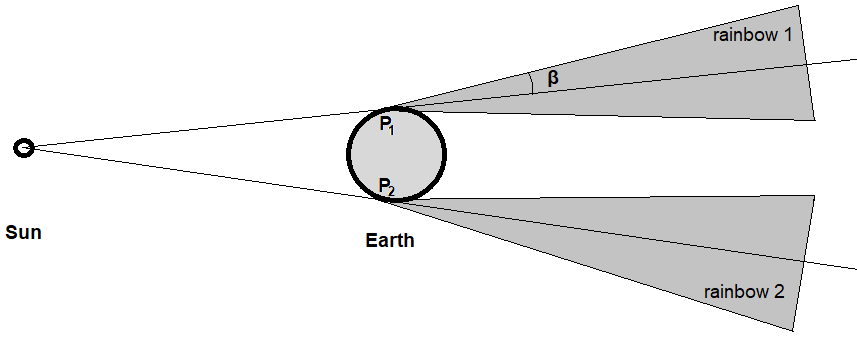
Your colleague is right. The rainbow is a virtual image (not a physical object) similar to a mirror image. The rain droplets are similar to small mirrors or scatterers. The virtual origin of the light rays, where the extrapolated rays intersect, is approximately at infinity. In geometrical optics, the exact distance of a virtual image is defined by its parallax. If the rainbow is observed from different points on Earth, as in the diagram below, the center of the rainbow (the antisolar point) has a small negative parallax, corresponding to the location of the Sun, at a distance of 1 AU (astronomical unit).
The reference background for parallax measurement is the celestial sky. For example, in mid january the rainbow is approximately positioned over the stars Aldebaran, Rigel and Sirius. The stars are not visible in the sky during daylight, but a planetarium app on a smartphone would reveal their location. From day to day the center of the rainbow is moving slowly across the celestial sphere, at a speed of 1° per day along the ecliptic.
Generally, when discussing rainbows, it is more useful to think of that distance of 1 AU as approximately infinite, because for visual observations by eye or camera the difference is negligable. Then:
The rainbow is a 42° circle around the antisolar point. The antisolar point is opposite to the sun, located at infinity.
When using visual observations (eye or camera, possibly at different locations), the rainbow is indistinguishable from a 42° circle at infinity.
Imagine the Sun was replaced by an ideal monochromatic point source. The rainbow would then be a 42° sharp monochromatic circle instead of a blurry multicolor circular band. A camera lens would have to focus at infinity to obtain a sharp photo, otherwise the recording would be blurred.
About three days before or after full moon it is possible, occasionally, to see the moon in the rainbow (example). With some luck it should be possible to make simultaneous photos from different places on earth. The parallax difference would show that the rainbow is further away than the moon.
Some people think the rainbow is a cone. However, the cone is the location of the light rays, whereas the rainbow is the apparent origin of those rays: a 42° circle around the antisolar point, at infinity.
-
2
-
1$\begingroup$ You appear to be saying, on the basis of definitions of terms in optics, that the location of the rainbow is at the Sun. I see that it is relevant and correct to note the parallax indicated by the diagram, but all the same, definitions of terms or not, it is simply not a helpful use of language to say that when looking in front of me I am looking at a virtual image that is behind me. I think it more helpful to agree that a rainbow is a set of colours arriving from different directions; it does not need to have a distance or a location, and has neither. What do you think? $\endgroup$ Commented Nov 14, 2018 at 21:10
-
$\begingroup$ My first paragraph, about the negative parallax due to a Sun at 1 AU distance, is merely a response to the claim quoted by the questioner. I admit the effect is unnoticably small. The rest of my reply is more straightforward, because it replaces the Sun by a point source at infinity. The rain scatters the light in such a way that the apparent origin of the rays is a 42° circle at infinity. All observers see the circle at the same location among the stars in the celestial sky. It is the plain language of geometrical optics that results in this well defined rainbow location at infinity. $\endgroup$– jkienCommented Nov 17, 2018 at 23:41
A rainbow is formed when sunlight beams its way through a thin mist of water droplets in a large cloud at the right angle for the light to be refracted into its constituent colors and reflected back out of those droplets towards your eye. All of the droplets at that magic angle, all the way through the entire depth of the cloud, contribute to the rainbow image that you see: that image is not an arc residing at a measurable distance but an extended cone cutting through the cloud with its apex at your eye and as such it has no single location. This means your colleague is wrong.
-
2$\begingroup$ niels, I completely agree. And since everyone is in a different location when they view a rainbow, everyone sees their own "special" rainbow, due to the fact that all observers are viewing the "rain field" from a different angle. $\endgroup$ Commented Oct 25, 2018 at 0:08
-
3$\begingroup$ -1 You're not thinking extensively enough. Could you (in principle) measure the distance to the sun using parallax? What if you did it by way of a mirror basically orthogonal to the line between the sun and observer? $\endgroup$– MikeCommented Oct 25, 2018 at 0:54
-
$\begingroup$ Using parallax, you would have to conclude that a rainbow is behind you, at the position of the sun. $\endgroup$ Commented Oct 25, 2018 at 4:19
Just for you, the rainbow you see yourself, If you can identify an object “behind the colors” and another object “in front of the colors” could you not approximate the distance from you to a rough point between these objects, and that would be about how far the rainbow is from you?