Gravitational attraction between a particle and a bar

Question

I'm trying to solve this problem from the book "Fundamentals of Applied Dynamics" by R.A.Tenenbaum.

I start it by trying to find the resultant gravitational force by the means of integration.

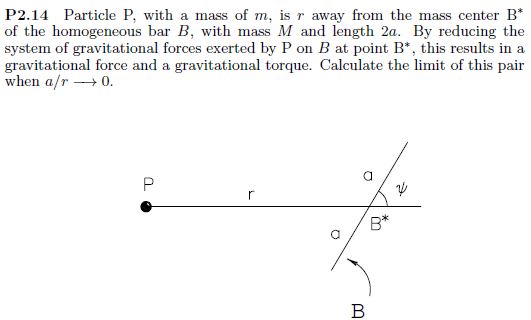
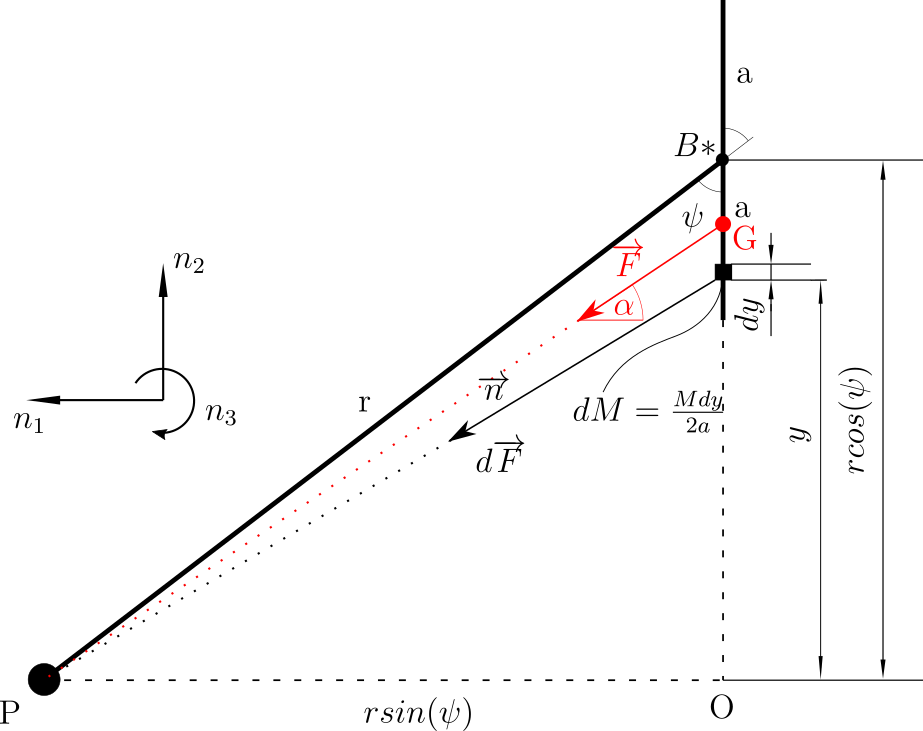
$$d\overrightarrow{F}=\frac{GmdM}{d^2}\overrightarrow{n}=\frac{GmMdy}{2a\sqrt{(r^2sin^2(\psi)+y^2)^2}}*\overrightarrow{n}= \frac{GmMdy}{2a(r^2sin^2(\psi)+y^2)^{3/2}}*(rsin(\psi)\overrightarrow{n_1}-y\overrightarrow{n_2})$$

$$\overrightarrow{F}=\int_{rcos(\psi)-a}^{rcos(\psi)+a}\frac{GmMdy}{2a(r^2sin^2(\psi)+y^2)^{3/2}}(rsin(\psi)\overrightarrow{n_1}-y\overrightarrow{n_2})$$

$$ \overrightarrow{F}=(\frac{GmM(rcos(\psi)+a)}{2arsin(\psi)\sqrt{r^2sin^2(\psi)+(rcos(\psi)+a)^2}}-\frac{GmM(rcos(\psi)-a)}{2arsin(\psi)\sqrt{r^2sin^2(\psi)+(rcos(\psi)-a)^2}})\overrightarrow{n_1}+(\frac{GmM}{2a\sqrt{r^2sin^2(\psi)+(rcos(\psi)+a)^2}}-\frac{GmM}{2a\sqrt{r^2sin^2(\psi)+(rcos(\psi)-a)^2}})\overrightarrow{n_2}$$

When I let a/r go to zero, I get $$F=GmM/r^2*\overrightarrow{n_1}$$ (I'm not sure that this step is correct, it probably isn't). Now the common sense tells me, that if a/r goes to zero, the bar is reduced to a point mass and I would expect that the resultant should be of the form: $$GmM/r^2 (sin(\psi)\overrightarrow{n_1} - cos(\psi)\overrightarrow{n_2})$$ without any gravitational torque. However the answer according to the author is: $GmM/r^2$ and $GmMa^2/2r^3*sin(2\psi)$ (the force and the torque). I would appreciate some guidance on this.

Answer 1

"When I let a/r go to zero, I get $$F=GmM/r^2*\overrightarrow{n_1}$$ (I'm not sure that this step is correct, it probably isn't)."

You're right: it isn't. Your term in $\vec {n_2}$ needs more careful handling...

Hope you agree that it can be written $$\frac{GmM}{2ar}\left[\frac{1}{\sqrt{1 + 2(a/r)cos(\psi)+(a/r)^2}}-\frac{1}{\sqrt{1 - 2(a/r)cos(\psi)+(a/r)^2}})\right]\overrightarrow{n_2}$$

Using the binomial series to first order on the reciprocal square roots, we get

$$\frac{GmM}{2ar}\left[(1 - (a/r)cos(\psi)-\tfrac12 (a/r)^2)-(1 + (a/r)cos(\psi)-\tfrac12 (a/r)^2)\right]\overrightarrow{n_2}$$

And that boils down to

$$-\frac{GmMcos(\psi)}{r^2}\overrightarrow{n_2}$$

As for $\vec n_1$, the reciprocal square roots are the same as for $\vec n_2$, so treating them in the same way, but neglecting $(a/r)^2$ terms right from the beginning, we get

$$\frac{GmM}{2ar^2 \sin \psi}\left[(r \cos \psi +a)(1 - (a/r)cos(\psi))-(r \cos \psi - a)(1 + (a/r)cos(\psi))\right]\overrightarrow{n_2}$$

Multiplying out, tidying and neglecting terms in $(a/r)^2$ we get

$$\frac{GmM}{2ar^2 \sin \psi}\left[2a-2a \cos^2 \psi \right]\overrightarrow{n_2}\ =\ \frac{GmM}{r^2 }\left[\sin \psi \right]\overrightarrow{n_2}$$

So the magnitude of the force when $r\gg a$ is

$$GmM/r^2 \sqrt{\left[(sin(\psi)\overrightarrow{n_1} - cos(\psi)\overrightarrow{n_2})\right].\left[(sin(\psi)\overrightarrow{n_1} - cos(\psi)\overrightarrow{n_2})\right]}=GmM/r^2$$

Answer 2

Here is my attempt to find an expression for the gravitational torque with respect to B*. Firstly, I define some quantities: $$f=\sqrt{r^2sin^2(\psi)+(rcos(\psi)+a)^2}$$ $$e=\sqrt{r^2sin^2(\psi)+(rcos(\psi)-a)^2}$$ $$F_1=\overrightarrow{F}\cdot \overrightarrow{n_1}=\frac{GmM(e(rcos(\psi)+a)-f(rcos(\psi)-a))}{2arsin(\psi)(f\cdot e)}=\frac{GmM((e-f)rcos(\psi)+(e+f) a)}{2arsin(\psi)(f\cdot e)}$$ $$F_2=\overrightarrow{F}\cdot \overrightarrow{n_2}=\frac{GmM(e-f)}{2a(f\cdot e)}$$ The resultant gravitational force intercepts the bar at some point “G”. The distance of this point to the point “O” is: $r\cdot sin(\psi) \cdot tan(\alpha)$. $$tan(\alpha)=F_2/F_1=\frac{(e-f)rsin(\psi)}{rcos(\psi)(e-f)+a(e+f)}$$ The distance of point G to B* is $$ ^{G}\overrightarrow{r}^{B*}=[rcos(\psi)-rsin(\psi)tan(\alpha)]\overrightarrow{n2}=[rcos(\psi)-\frac{(e-f)r^2sin(\psi)^2}{rcos(\psi)(e-f)+a(e+f)}]\overrightarrow{n_2}=[\frac{(e-f)(r^2cos(\psi)^2-r^2sin(\psi)^2)+(e+f)r\cdot a\cdot cos(\psi)}{rcos(\psi)(e-f)+a(e+f)}]\overrightarrow{n_2}$$ The gravitational torque with respect to B* is $$\overrightarrow{T}=^G\overrightarrow{r}^{B*} \times \overrightarrow{F} = [(rcos(\psi)-rsin(\psi)tan(\alpha))\cdot F_1]\overrightarrow{n_3}= [\frac{(e-f)(r^2cos(\psi)^2-r^2sin(\psi)^2)+(e+f)r\cdot a\cdot cos(\psi)}{rcos(\psi)(e-f)+a(e+f)}\cdot \frac{GmM(rcos(\psi)(e-f)+a(e+f))}{2a\cdot r\cdot sin(\psi)(f\cdot e)}]\overrightarrow{n_3}$$ Using binomial series: $$f=r(\sqrt{1+2a/rcos(\psi)+(a/r)^2})=r+acos(\psi)$$ $$e=r(\sqrt{1-2a/rcos(\psi)+(a/r)^2})=r-acos(\psi)$$ $$e-f=-2acos(\psi)$$ $$e+f=2r$$ $$e \cdot f = r^2-a^2cos(\psi)^2 = r^2(1-(a/r)^2cos(\psi)^2)=r^2$$ Putting everythin into the torque equation: $$[\frac{-2a\cdot r^2\cdot cos(\psi)(cos(\psi)^2-sin(\psi)^2)+2a\cdot r^2cos(\psi)}{2a\cdot r^3sin(\psi)}GmM]\overrightarrow{n_3}= [\frac{2a\cdot r^2\cdot cos(\psi)(1-cos(\psi)^2+sin(\psi)^2)}{2a\cdot r^3sin(\psi)}GmM]\overrightarrow{n_3}= [\frac{2a\cdot r^2\cdot cos(\psi)(2sin(\psi)^2)}{2a\cdot r^3sin(\psi)}GmM]\overrightarrow{n_3}= [\frac{GmM}{r}sin(2\psi)]\overrightarrow{n_3}$$ According to author the gravitational torque as a/r tends to zero is $$\frac{GmMa^2}{2r^3}sin(2\psi)$$ and my solution differs from it by a factor of $(1/2)(a/r)^2$. It looks like some of the higher order coefficients that I neglected using the binomial series but I cannot figure it out yet. Is the author's expression even correct? If we want to find the limit as (a/r) tends to zero, then the limit of his expression for the torque should be zero.