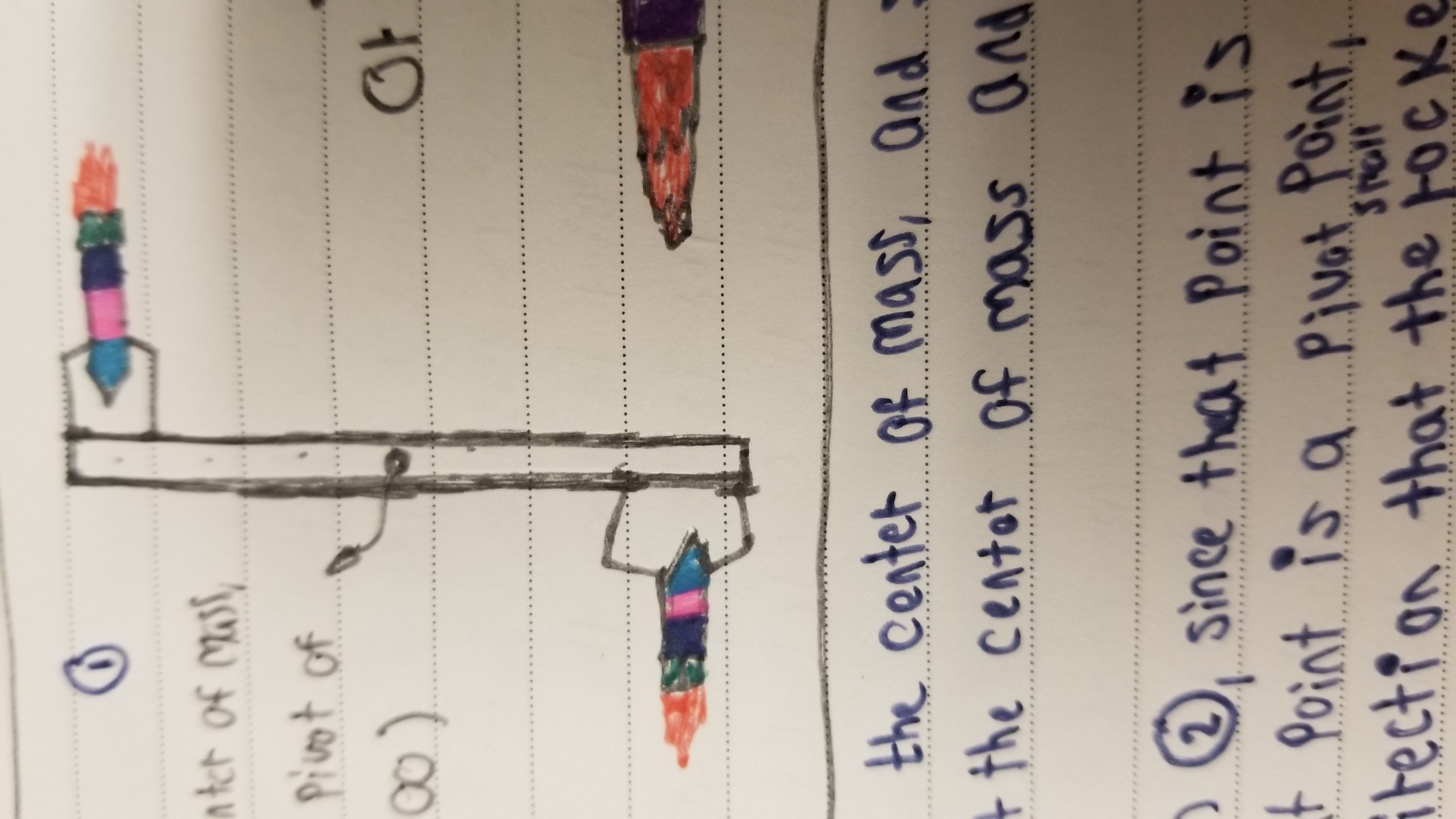
Say we have a solid bar in space. It is on a pivot, the pivot being right at the bar's center of mass
There is a massless rocket pushing on one end of it, making it spin, faster and faster.
Suddenly, the pivot disappears, and at the same time the rocket disappears, and thus the mass does not have a force on it anymore and is also free to move about.
What would happen? Would the mass just spin in place, or would it go off in the last direction the rocket pushed it?
I have a theory for each, but they are contradictory:
1. The mass stays in place spinning around its center of mass:
Momentum from the top spinning part balances out with momentum from the bottom spinning part, meaning the bar only has angular momentum but no translational momentum overall.
This makes sense, and if there was a rocket in the top part providing momentum in the opposite direction, I would accept it without further question.
However, the rocket is only on one side of the rod, so that leads me to think...
2. The mass keeps on spinning, but now has translational momentum as well
The reason for this is because the part with the rocket attached got the last "piece of momentum" before the rocket disintegrated, and thus will move the mass forwards...?
Also, I have a sub-questions to add:
1. What if the pivot was further up on the rod and then the same thing happened? Or, equivalently center of mass wise, what if the top part without the rocket was more massive than the bottom part?
Thank you!