1
$\begingroup$

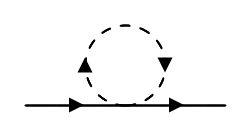
Given the Lagrangian $$\mathscr{L}=\bar{\psi}\left(i\partial\!\!\!/-m\right)\psi +\frac{1}{2}\left(\partial\phi\right)^2- \frac{1}{2}M^2\phi^2 - g\bar{\psi}\psi\phi^2,$$ calculate the propagator correction for the $\psi$ field at the first non-vanishing order in $g$.

I can't understand if this

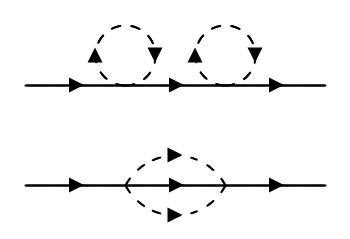
really is a correction to the propagator: it's first order in $g$, but the vertex has one $\psi$ and one $\bar\psi$. If this is not a correction, do the first non-vanishing terms are

(second order in $g$)?

Improve this question
$\endgroup$
6
  • $\begingroup$ If you're worrying whether the vertices are legal: as long as you can get the fermion arrows to match up, it's fine. Your first diagram is allowed. $\endgroup$
    – knzhou
    Commented Oct 6, 2018 at 10:21
  • $\begingroup$ @knzhou yes, this is exactly the point. Could you please briefly explain me why this is the case? Really thank you. $\endgroup$
    – Stig
    Commented Oct 6, 2018 at 11:06
  • 1
    $\begingroup$ Related: [Diagrams involved in 1-loop electron self energy in QED][1] Your interaction is $-g\bar{\psi} \psi\phi^2$. Practically speaking it means that every vertex must be touched by 2 fermionic lines and 2 bosonic lines. This is indeed the case for your first diagram. [1]: physics.stackexchange.com/questions/431609/… $\endgroup$
    – DavideL
    Commented Oct 6, 2018 at 12:14
  • 2
    $\begingroup$ To understand why, think in terms of Wick's theorem. Your notation may differ, but what you're looking for is $$\langle \psi(x) \bar{\psi}(y)e^{iS_{\text{interaction}}} \rangle = \langle \psi(x) \bar{\psi}(y)e^{-ig \int d^4z \bar{\psi}(z) \psi(z) \phi^2(z)} \rangle = \langle \, \psi(x) \bar{\psi}(y) \rangle + (-ig) \int d^4z \, \langle \, \psi(x) \bar{\psi}(y) \, \bar{\psi}(z) \psi(z) \phi^2(z) \, \rangle + O(g^2)$$ $\endgroup$
    – DavideL
    Commented Oct 6, 2018 at 12:15
  • 2
    $\begingroup$ To first order in the coupling you are dealing with the full contraction of 6 fields: 2 external fermions, 2 internal fermions and 2 inter. bosons. The two bosons must be contracted between each other. Considering that each $\psi$ can in principle be contracted with each $\bar{\psi}$ this leaves two possibilities: $\psi(x)-\bar{\psi}(y) \quad \psi(z)-\bar{\psi}(z)$, which is disconnected, and $\psi(x)-\bar{\psi}(z) \quad \psi(z)-\bar{\psi}(y)$, which is your first diagram. As for the non-vanishingness of the diagram, I got my own doubts (see related question, so I'm just commenting. $\endgroup$
    – DavideL
    Commented Oct 6, 2018 at 12:16

1 Answer 1

Reset to default
1
$\begingroup$

Expanding from the comments: your first diagram has the correct vertex structure for the reasons explained in the comments, and it is actually non-zero. The vertex does not introduce any spinorial structure, and the propagator is the simple bosonic one. E.g. in dimensional regularization, after a Wick rotation:

\begin{equation} \begin{split} (-ig) \int \frac{d^dk}{2\pi^d} \, \frac{i}{k^2-m^2} &= -ig \int \frac{d^4k_E}{2\pi^4} \, \frac{1}{k_E^2+m^2} \\ &=\frac{-ig}{(4\pi)^{d/2}} \left(\frac{1}{m^2}\right)^{1-d/2}\Gamma(1-d/2) \end{split} \end{equation}

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.