Let's take two inductors: one has its peak current (say, Io) flowing through itself and the other has no current through itself. Now if these two inductors are joined in series in a closed circuit, the first inductor will try to keep the current at Io while the second one will try to keep it at zero.
To solve this, I created an analogy between inductor and mass. When two masses with different velocities collide, the inertia of each mass tries to keep its velocity unchanged. Thereafter we use principle of conservation of linear momentum and value of coefficient of restitution to find the final velocities of the two masses. But here, in case of inductors, I do not know which physical quantity is analogous to linear momentum and hence I can not continue any further.
My Physics teacher told me that we need to equate the total magnetic flux in the inductors before and after joining them but he did not give any reason behind doing so.
So my questions are:
- Why is the total magnetic flux, before and after joining the inductors, equal?
- Since I tried creating analogy between collision of two masses and joining two inductors, will there be energy loss in the latter case? And is there any quantity analogous to the coefficient of restitution on which the magnitude of loss of energy depends?
PS:
- By loss of energy, I mean reduction in energy stored in the inductors.
- The actual question that I was solving is this:
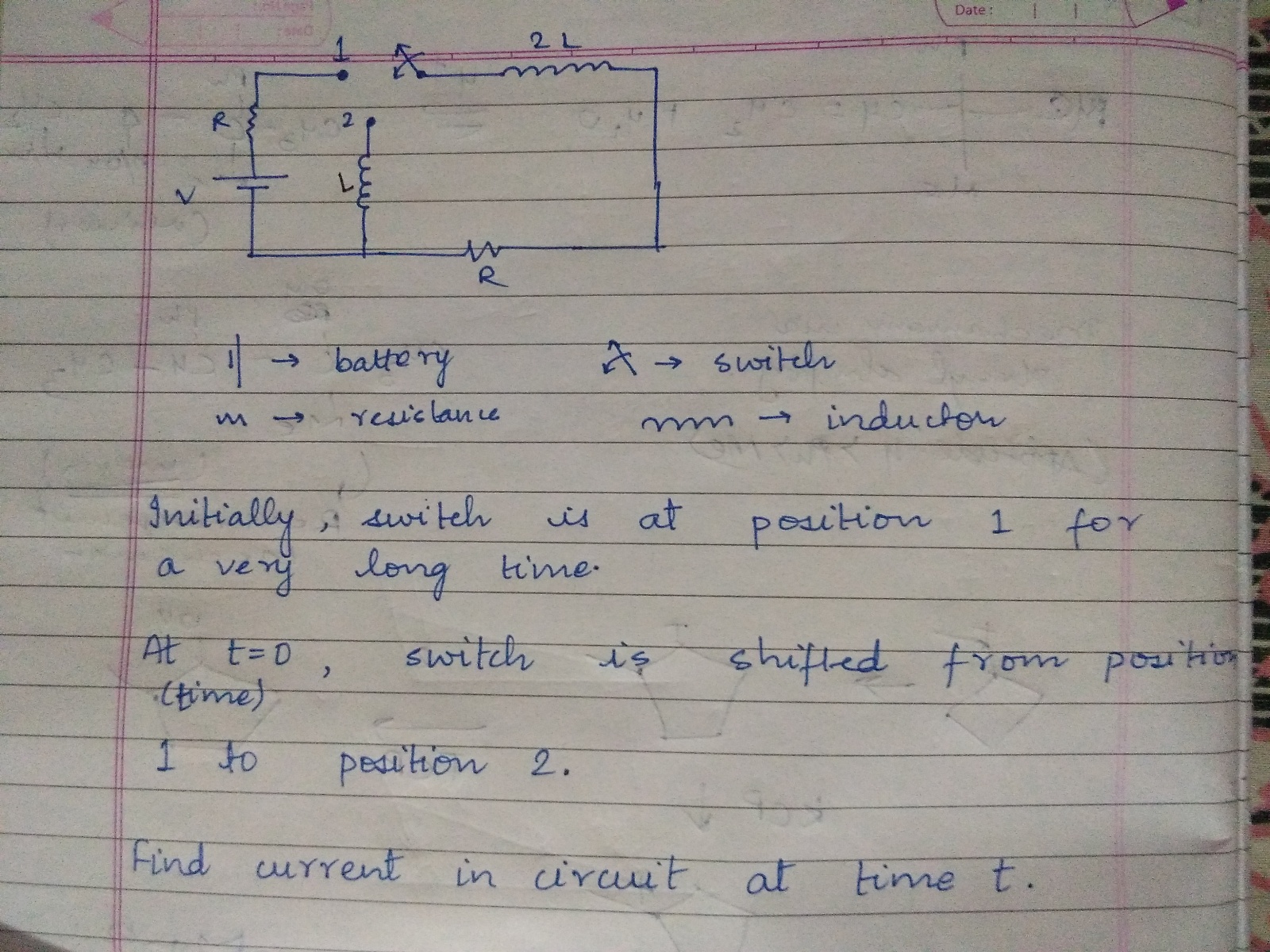 I do not need a solution of this question. This question is just for reference.
I do not need a solution of this question. This question is just for reference.
Edit: There are two other questions similar to my question (I tried searching for related questions before asking my own question but could not find one):
As for the first one, I have not been yet taught about terms such as Inverse Laplace transformation and parasitic interwinding capacitance. Hence I can't grasp what is being discussed there.
The second question has a greater similarity to my question (as I have also asked about reason behind conservation of magnetic flux) but there is only one answer to that question which does not tell why flux is conserved. Hence my question doesn't have an answer there too.
Moreover, I have also asked about analogy between mechanical system and electrical system which neither of the above two questions have talked about. And I have already got an answer here which has solved the question using that analogy.
