0
$\begingroup$

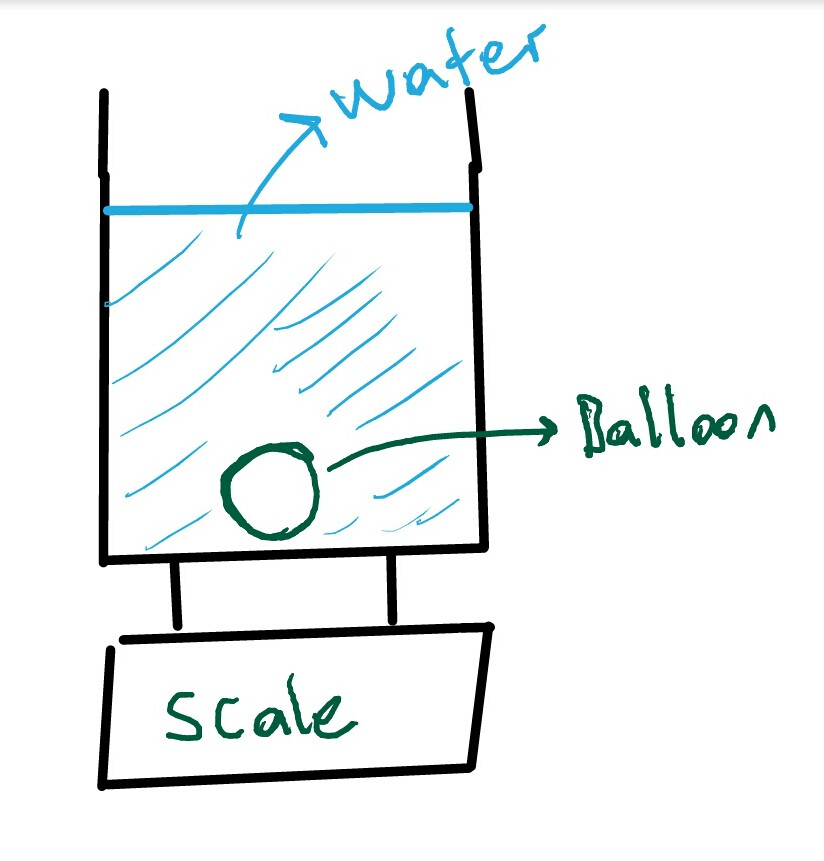
The balloon rises beause of buoyant force being greater than its weight, I'm interested in the change in scale reading while the balloon is still completely underwater. And let's ignore any viscous forces.

Here is the way I think.

While the balloon goes up, it starts to expand because of weaker pressure on it. Because it is going up, water takes its place and the center of mass of the water-balloon-container system accelerates downward. That means that the net force on the system must point downward. So the weight of the system gets more than the reaction force exerted by the scale and the scale reading gets smaller.

I'm not sure about the center of mass of the system accelerating downward though. When I think of this problem in terms of energy conservation; balloon gets potential and kinetic energy, so water has to lose potential energy due to the conservation law. That means the center of mass should be moving downward.

First of all this is not a homework question. I'm just curious about it. I'm looking forward to seeing your comments..

system

Improve this question
$\endgroup$
8
  • $\begingroup$ Newton's 3rd law applies here. There is a net upward buoyant force on the balloon pushing it up. There is a force of equal magnitude and opposite direction pushing down into the liquid. As a result, the scale reading shouldn't change as the balloon rises. $\endgroup$
    – David White
    Commented Sep 1, 2018 at 16:22
  • $\begingroup$ is the buoyant force constant during motion? I think not. $\endgroup$
    – physicsguy19
    Commented Sep 1, 2018 at 16:31
  • $\begingroup$ No, the buoyant force is not constant. But Newton's 3rd law still applies. $\endgroup$
    – David White
    Commented Sep 1, 2018 at 16:40
  • $\begingroup$ physicsguy19, here is an easy experiment for you to try. Put a beaker full of water on a triple beam balance. Don't fill the beaker completely full. Move the weights around on the beam until the beam is balanced and you can read the weight of the beaker and water. Now, carefully put your finger into the water, but make sure that you don't touch the beaker or spill any water. What happens to the pan of the triple beam balance? Does the pan go up, down, or stay in the same position? $\endgroup$
    – David White
    Commented Sep 1, 2018 at 16:44
  • $\begingroup$ so you are saying that center of mass of the system has no acceleration? $\endgroup$
    – physicsguy19
    Commented Sep 1, 2018 at 17:38

1 Answer 1

Reset to default
3
$\begingroup$

You are correct that if the center of mass of the system accelerates downwards then the force will be less than $mg$. When the balloon is initially released there will be a brief period of acceleration, but soon the balloon will reach “terminal velocity”. At that point the center of mass is still moving downwards, but not accelerating. The force will then be equal to $mg$ again

Improve this answer
$\endgroup$
4
  • $\begingroup$ what if we ignore viscous forces? $\endgroup$
    – physicsguy19
    Commented Sep 1, 2018 at 16:55
  • $\begingroup$ Then there is no terminal velocity. $\endgroup$
    – Dale
    Commented Sep 1, 2018 at 17:03
  • $\begingroup$ is it ok if I edit my post then? I have to mention this. $\endgroup$
    – physicsguy19
    Commented Sep 1, 2018 at 17:05
  • $\begingroup$ That is ok by me. $\endgroup$
    – Dale
    Commented Sep 1, 2018 at 17:14

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.