I am looking at a question from University Physics
The given answer
Whats the intuition behind using the below diagram
of finding $v_{tip}$? I was looking the the $v_{tan}$ not knowing how to continue. Also $v_{tip}$ will rotate and turn positive and negative
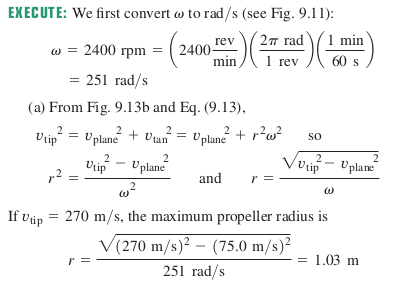
Firstly, we are just talking about the magnitude of $v_{tip}$. This will not change with rotation of the velocity. In the question we do not care about the direction of the velocity. It just says it may never move faster than $270 m s^{-1}$. That is why the solution only uses $v_{tip}^2$.
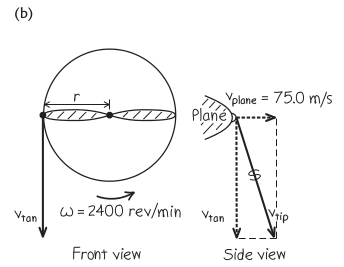
Now to find $v_{tip}^2$ they decide to use a configuration (position) of the propeller which is easy to handle. When the propeller is horizontal, the radial (tangential) velocity of the tip $v_{tan}$ is pointing downward. Additionally the plane is move forward with $v_{plane}$, so the propeller is moving forward at the same speed, too. Since these velocities are perpendicular to each other, we can apply a simple superposition to add them up (as the "Side View" diagram shows). Then to get the total $v_{tip}$ we use Pythagoras:
$|v_{tip}| = \sqrt{v_{plane}^2+v_{tan}^2}$
or
$v_{tip}^2 = v_{plane}^2+v_{tan}^2$
Now of course, the propeller will rotate, so the direction of $v_{tan}$ will change. But it will always be of the same magnitude, and always perpendicular to $v_{plane}$. Hence, the same equation will hold for all positions of the propeller.
