Deriving the boundary condition for this flow

Question

The Question:

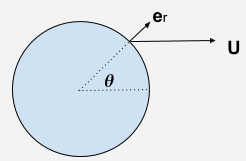
Suppose a circular cylinder of radius $a$ moves with constant velocity $U$ in the $x$-direction in a two-dimensional irrotational, incompressible flow whose velocity decays to zero at infinity.

The circulation around the cylinder is zero.

Let $\phi$ be the velocity potential of the flow. Show that

$$\frac{\partial \phi}{\partial r} = U\cos \theta \qquad \text{ on } \;\;r=a$$

where $(r,\theta)$ are the plane polar coordinates.

---

So basically, I don't really know how to put all the given information together.

How do I deduce anything about the velocity potential at $r=a$ if the cylinder moves with constant velocity?

Any hints would be much appreciated.

Answer 1

When thinking about boundary conditions, you need to think about a few things. First, how many are required and what type might they be? You correctly noted (in your comment) that the governing equation you are solving is:

$$ \nabla^2 \phi = 0 $$

which is Laplace's equation. How many boundary conditions does it need? What are the types of boundary conditions that could be applied?

Once you have your list of number and possible types of boundary conditions, you have to decide which ones to use. To do that, you need to make a list of assumptions and decide how to enforce physical boundary conditions consistent with those assumptions. You noted correctly (again in your comment) that the flow is irrotational and incompressible. But you missed an assumption:

Is the flow viscous or inviscid?

Once you add that assumption in, what does it tell you about the boundary condition needed? Does the flow slip past the wall or is it zero on the wall? How does a slip or no-slip condition relate to the types and number of conditions you listed in the earlier step?

Answer 2

Since the flow is irrotational, velocity vector may be written as $\mathbf{u}=\nabla\phi$, where $\phi$ is a scalar potential. The cylinder moves with constant velocity $\mathbf{U}$ (vectors are denoted by bold-face font). At the cylinder's surface, normal velocity of both cylinder and fluid must be the same. This means that if $\mathbf{n}$ is the normal vector at the cylinder's surface then $\mathbf{n}\cdot\mathbf{u}=\mathbf{n}\cdot\nabla\phi=\mathbf{n}\cdot\mathbf{U}$.

Consider a cylindrical coordinate system whose origin is instantaneously coincident with the center of the cylinder. Now the normal to the surface of the cylinder is the unit radial vector $\mathbf{e}_r$. Therefore, at the cylinder's surface, we have: $$\mathbf{e}_r\cdot\nabla\phi=\mathbf{e}_r\cdot\mathbf{U}\\\Rightarrow\quad\frac{\partial\phi}{\partial r}=U\cos\theta$$ in which $U$ is the magnitude of cylinder's velocity.