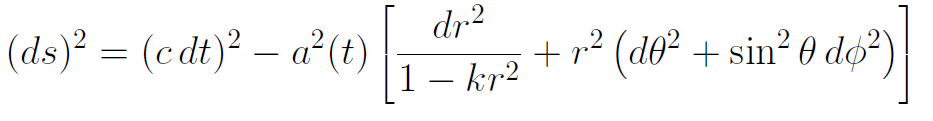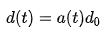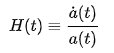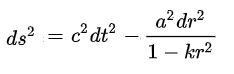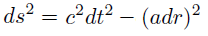A distant galaxy appears to move relative to the earth because of two different effects. The first is the comoving velocity which is an apparent speed due solely to the stretching or expansion of space. This comoving velocity is what appears as the galactic red shift, and because there is no actual relative motion there is no time dilation nor length contraction experienced. How could it not be so, since such a universal time dilation would break the symmetry that is necessary to derive the Friedman solution? The other component is called the peculiar velocity, and that is motion toward or away from us due to the motion of an object relative to the comoving frame. In this case, length contraction and time dilation do apply, but this is a very small component of the red shift from distant galaxies.
To show this we start with the Friedmann–Lemaître–Robertson–Walker metric:
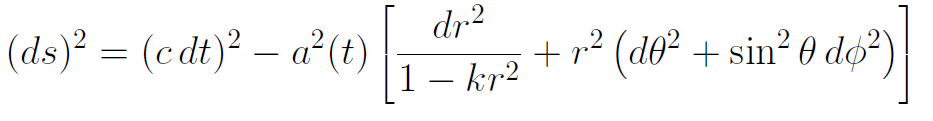
Where
- ds is the spacetime interval
- dt is the time interval
- dr is the spatial interval
- c is the speed of light
- a is the time-dependent cosmic scale factor
- k is the curvature per unit area
The important thing to note here is the cosmic scale factor a, which relates the comoving distance r (which does not change with the cosmic expansion) to the proper distance ar. The proper distance is the apparent distance in any epoch, as in the equation below, where here do is arbitrary and usually chosen as the distance at the current time:
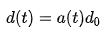
Following this, the Hubble parameter is:
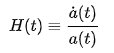
where the dot represents a time derivative. The Hubble parameter varies with time, not with space, with the Hubble constant Ho being its current value.
Going back to the FLRW metric, and the reduced circumference polar co-ordinates above, here we are interested only in the radial direction (the direction of the cosmic expansion) so the two angle terms can be chosen to be zero, simplifying the equation as follows:
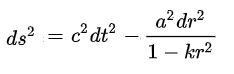
Now remember that in our chosen comoving co-ordinates, the spatial distance r is invariant with the cosmic expansion, and so for a galaxy that is comoving (that is it is moving with the "Hubble flow"), dr is equal to zero and substituting for ds we obtain
(c$\tau)^2$ = $\ (ct)^2$ or $\tau$ = t,
where $\tau$ is the time within the frame of the distant object. Substituting back we can see that the distance measures are preserved in the comoving frames of reference.
It is interesting at this point to consider the case of a relative motion within the comoving frame, that is the peculiar motion of the distant galaxy against the Hubble flow (which for distant galaxies is much smaller than the apparent or proper velocity). In order to simplify the example, we will take a "flat" universe, which is described by k (curvature per unit area) equal to zero. This would be the case in any local area, or on cosmic scales if the universe is flat (which current cosmology indicates that it is). With this simplification, and with dr representing the peculiar motion of an object within the frame, the radial version of the FLRW metric reduces to:
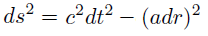
Since a*dr is just the radial motion of an object relative to the comoving co-ordinates in the current epoch, this reduces to the familiar Minkowski metric of special relativity. Thus, time dilation and length contraction do apply to the peculiar motion of the object, but time and space are invariant within any flat comoving frame.
The paper referenced by D Halsey below is discussing the observed time between events in distant galaxies as opposed to the perceived time. The observed time between events is larger because the wavelength of the light is stretched as it travels through space that is expanding, not because the time in the comoving frame of reference is dilated. The confusing thing here is that the observed time dilation is superficially similar, but not mathematically similar, to the time dilation in special relativity, however if you consider a contracting universe the observed time in the moving galaxy would be accelerated, which clearly has no analog in special relativity. A better analogy is a tune played by an orchestra, which would appear to last longer if the orchestra were moving away from you.