Finite size effect

Question

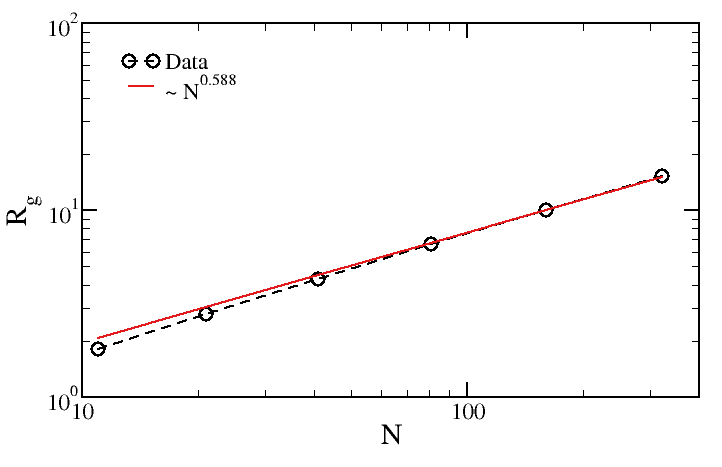
What does finite size effect mean in physics? I googled it and that is what I got:

"When studying any macroscopic system with a very large number of degrees of freedom, invariably make an approximation and simulate a smaller and/or discretized model system. This introduces systematic errors called finite size effects. Have to understand these, and be able to extrapolate to an infinite system, usually by doing a number of simulations at different system sizes."

But I am still a bit in the dark side. How for example, If I have a set of data from a system of for example 5 degrees of freedom, if I analyse and plot the data, how would I know that I should look at a larger system? How could the plot tell me that I probably have a finite size effect problem?

Answer 1

This is a very generic question. The only possible answer is: you simulate larger and larger systems till the quantities of interest become size-independent or their size dependence assumes a (known a priori) asymptotic behaviour.

Since you have tagged your question with "polymers", we will use the behaviour of a self-avoiding chain as an example. According to polymer theory, its radius of gyration in 3D should scale with the number of beads as $R_g(N) = b N^\nu$, where $b$ is a non-universal prefactor and $\nu \approx 0.588$. However, this holds true only in the so-called scaling regime where, by definition, finite-size effects are not present any more. The figure below shows the radius of gyration of self-avoiding polymers of different length. As you can see, it is only for polymers of size $\approx 100$ and beyond that simulation data takes on the theoretical behaviour.