This is a very generic question. The only possible answer is: you simulate larger and larger systems till the quantities of interest become size-independent or their size dependence assumes a (known a priori) asymptotic behaviour.
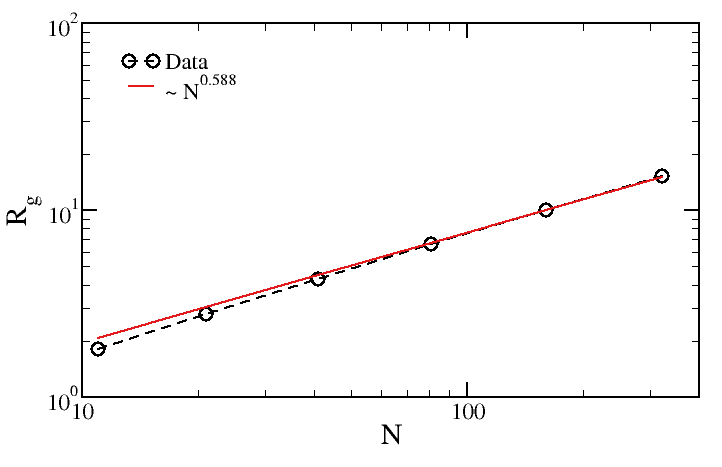
Since you have tagged your question with "polymers", we will use the behiaviourbehaviour of a self-avoiding chain as an example. According to polymer theory, its radius of gyration in 3D should scale with the number of beads as $R_g(N) = b N^\nu$, where $b$ is a non-universal prefactor and $\nu \approx 0.588$. However, this holds true only in the so-called scaling regime where, by definition, finite-size effects are not present any more. The figure below shows the radius of gyration of self-avoiding polymers of different length. As you can see, it is only for polymers of size $\approx 100$ and beyond that simulation data takes on the theoretical behaviour.