2
$\begingroup$

As far as I know a charged particle emits a photon when it undergoes acceleration.

When it comes to collisions it seems quite straightforward that a single photon is emitted with an energy related to the energy lost by the charged particle.

However, in case of an oscillating charge, or more generally, in case of a charge experiencing acceleration that is changing with time, it is unclear to me what is triggering the photon emission. Is it the change of acceleration? Is it something else?

Improve this question
$\endgroup$
1
  • $\begingroup$ Nothing "triggers the emission of a photon" since this is not a classical but a quantum effect, the system does not skip to another state, but the quantum state continuously changes while the particle is accelerated (and the probability to actually measure a photon in the state increases with time). $\endgroup$
    – Sebastian Riese
    Commented Apr 16, 2018 at 17:19

1 Answer 1

Reset to default
1
$\begingroup$

Classical electromagnetism describes this radiation well and is used for calculating the radiation.

Photons though are in the realm of quantum mechanics. Quantum mechanical calculations introduce some corrections in synchrotron and bremsstrahlung radiation.

For example bremsstrahlung , braking/decelerating radiation can be calculated using the tools of quantum electrodynamics. Here is a Feynman diagram for bremsstrahlung

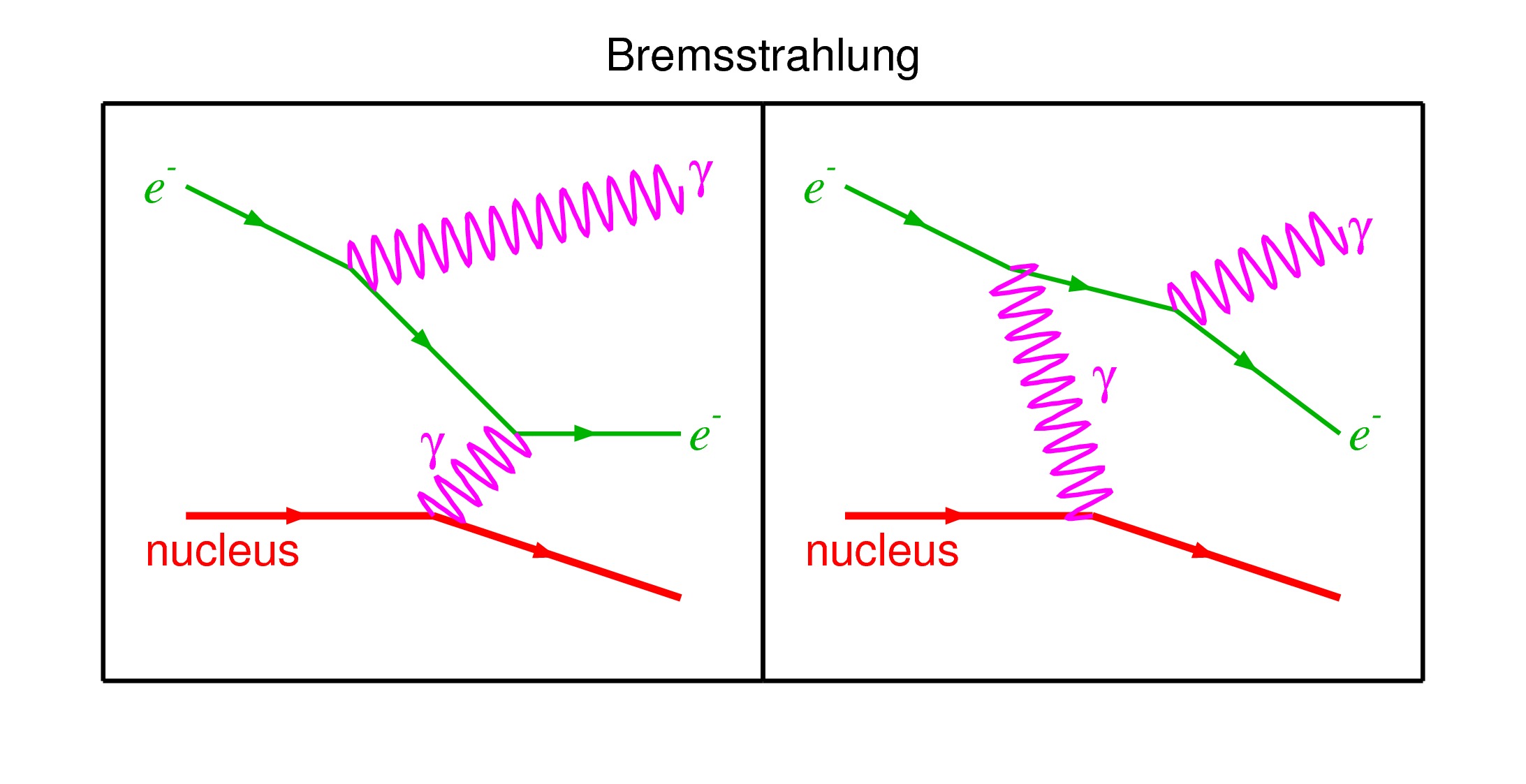
brehms

If one calculates it one will get the probability distribution for a photon to be emitted, and this probability distribution describes the spectrum . Individual photon emissions follow this probability distribution, as with all quantum mechanical interactions, individual photon measurements will look random, but they add up to the probability distribution predicted.

Corresponding diagrams can be written for acceleration, where the virtual photon does not come from the nucleus but from the field in an accelerator, creating synchrotron radiation. Again individual photons will look random, but the cumulative distribution is predicted by the quantum mechanical solutions.

There is no "trigger" for the photon emission other than the exchange of a virtual photon that will generate the probability functions for a real photon to appear.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Well, is it to say that the Larmor formula is a good approximation of the probability peak in certain cases? Is it to say that when a radio is emitting RF EM radiation there must be some basic "noise" arising from the very low probability photons that come at a frequency different from the tank circuit itself (which is the most probable frequency)? $\endgroup$
    – Lorand Nagy
    Commented Apr 17, 2018 at 6:54

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.