Are the electromagnetic waves transverse?

Question

The em waves are said to be the oscillations o electric and magnetic field perpendicular to each other and to the direction of propagation of wave and hence transverse.

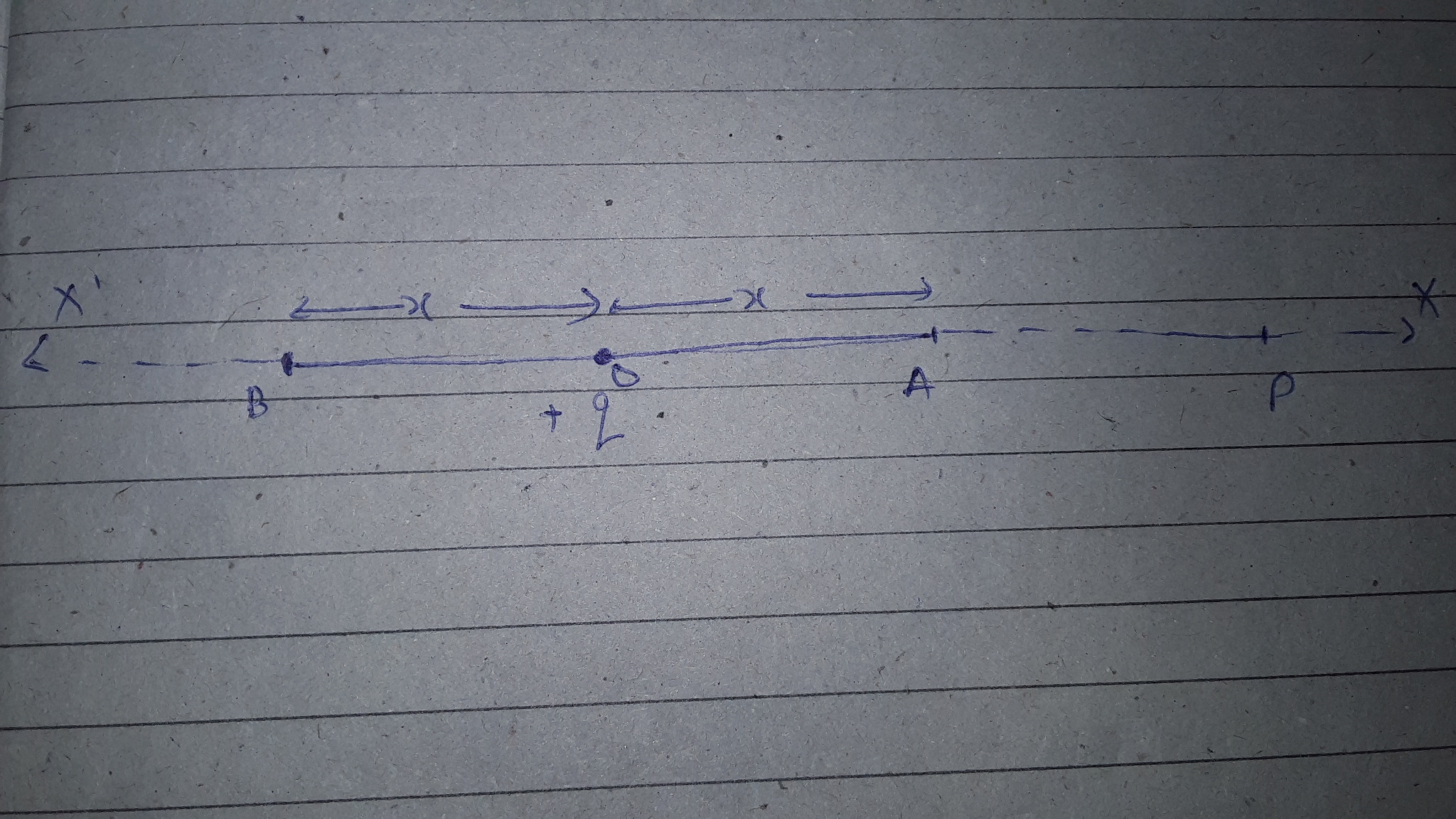
However consider a charged particle oscillating along x axis with no motion along y and z axis. Let it be at O. Consider a point P where we are considering the electric field due to charge. When the particle moves from O to A, the electric field at P increases. And when it goes from O to B, the electric field at P decreases by an equal amount. Since the charged particle is in SHM, the electric field at P will vary sinusoidally. However the varying electric field is also in direction of X axis. And the wave also propagates in this direction. So, it comes out to be longitudinal. However since magnetic field variation will be in perpendicular direction to electric field, it will also be perpendicular to direction of propagation of wave. So the wave should be partially longitudinal and partially transverse.

Answer 1

You are right in your observation of the electric and magnetic fields at a point P. This is, however, a consideration of the so-called near-field of an oscillating charge. The near field doesn't constitute a freely propagating electromagnetic field. To get the freely propagating (far field) electromagnetic field, you have to consider distances much larger than the wavelength corresponding to the oscillation ferquency. Then you will see that the propagating field are transverse EM waves.

Note added later: Irrespective of the distance, the near field (electric and magnetic) decays as $1/r^2$ and the far field decays as $1/r$, corresponding to propagating electromagnetic fields. Thus far enough from the source, the far field is dominating.

If you look at the electric field derived from the Lienard-Wiechert potential (See Wikipedia, https://en.wikipedia.org/wiki/Liénard–Wiechert_potential) of a point charge moving (accelerating) in x-direction, there is only a near-field component in x-direction at any distance and no far-field component. In particular, there is no transverse electric or magnetic field.

Answer 2

If I’ve understood your geometry properly, you may have a confusion around language.

The system you’re describing doesn’t emit any “electromagnetic radiation” along the axis of its motion. That’s what’s generally meant when people talk about “electromagnetic waves”: the “waves” invoke something that can travel far from its source.

Yes, there are changing E (along the axis) and B (around the axis) fields there, but they’re not what people mean when they refer to “electromagnetic radiation”: they’re not radio, light, etc.

Formally, this is because the fields at the far point fall off like $1/r^2$, which in turn happens because they’re only due to the source particle.

When fields are created in the right EM-wave configuration, they self-reinforce: the fields at a distance are sourced, hence strengthened, by fields at a distance, so they only drop off as $1/r$, hence travel through free space. That’s what we call radiation, making up the usual idea of electromagnetic waves.