3
$\begingroup$

I was imagining how an oscillating charge would produce an electromagnetic wave and I got stuck at a point. We know that the direction of propagation of em waves is perpendicular to both the constituent fields. But in my view of that wave generation process (which I suspect to be wrong) , I am getting a field along the wave propagation. Here's how I got to it :-

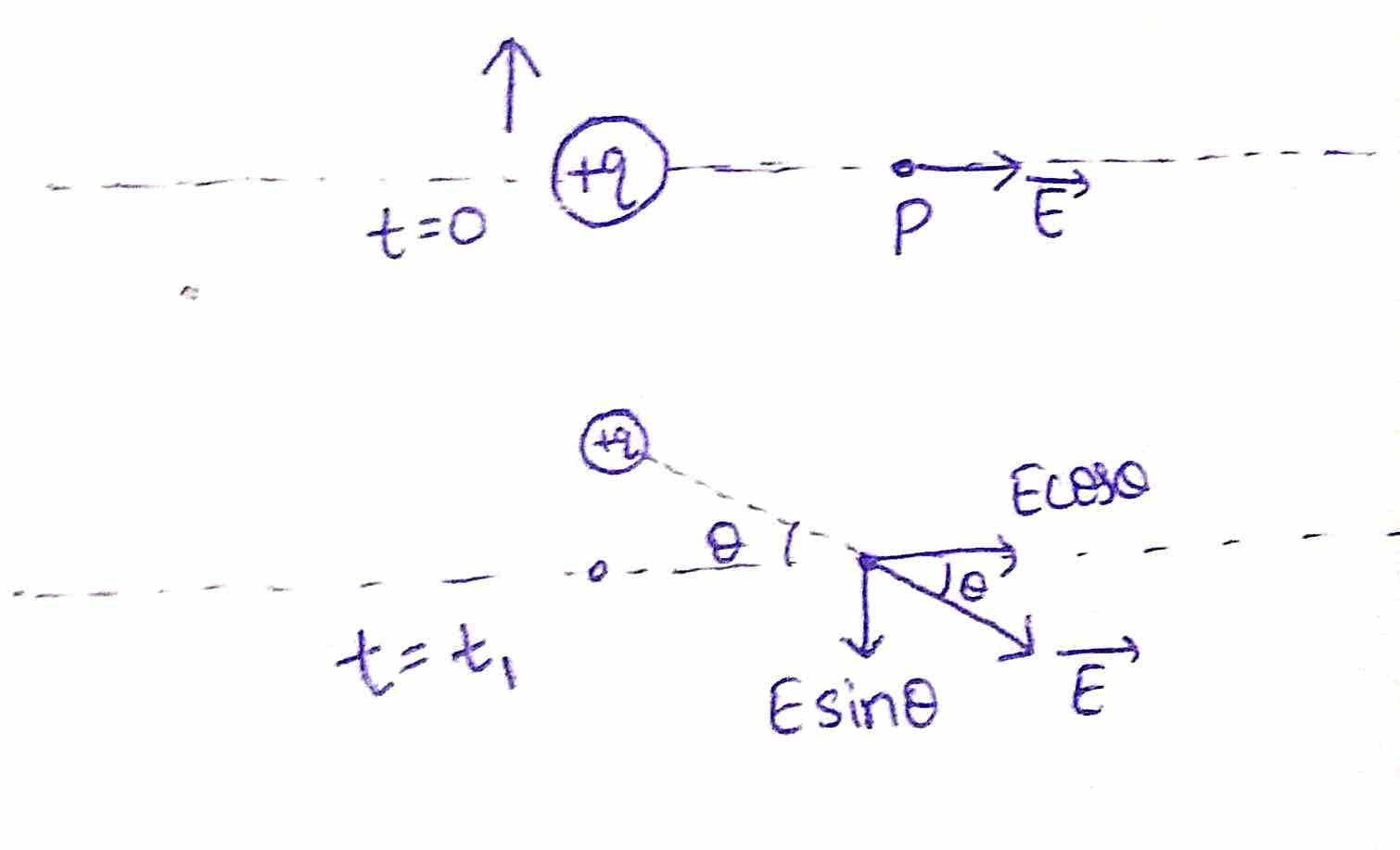
Suppose I have an oscillating positive charge oscillating with a constant angular frequency.

enter image description here

If at $t=0$ the charge is at its mean , the electric field at the point "P" would be along the dashed line (which shows the direction of propagation of the em wave) .

Now after some time the particle is somewhere above the mean point and so if I indicate the direction of electric field at the point "P" , I do get some component along the dashed line and this component is non zero for any position of the charge along its line of motion.

But isn't it contradictory to the theories of em waves !! I know I am making a blunder somewhere but I don't know exactly about it. Please someone point it out.

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ See Purcell E&M requires SR to resolve this properly $\endgroup$
    – Brian
    Commented Jun 29, 2022 at 21:37

1 Answer 1

Reset to default
8
$\begingroup$

You are correct, there will in general be a component of the electric field parallel to the direction of propagation but it will rapidly drop off in amplitude as the distance increases from the source.

This is part of the distinction between what is known and the "near field" and "far field". (See Wikipedia: Near and far field)

In the far field, the electric field falls off with an amplitude dependance of $1/r$ where $r$ is the distance from the source, therefore the power of the propagating wave drops as $1/r^2$. These fields, that drop off in this manner, have the electric and magnetic fields oriented perpendicular to the direction or propagation.

In the near field there can be all sorts of field orientations such that none of the electric field, magnetic field, and radial direction are perpendicular. All of the field components that contribute to this effect will fall off faster than $1/r$ and will eventually become negligible relative to the terms that are perpendicular to the direction of propagation.

Improve this answer
$\endgroup$
1
  • 8
    $\begingroup$ In short, the perpendicularity of $\vec B$ and $\vec E$ and $\vec P$ is only required for a plane wave (which is the limiting form of the far field). Other field configurations can easily be non-perpendicular. $\endgroup$
    – Ruslan
    Commented Jun 25, 2022 at 23:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.