Does special relativity imply that time dilation is affected by an orientation of clocks?

Question

Many texts about STR time dilation use as an example thought experiment with 2 mirror photon clock. The conclusion of this experiment is: In a frame moving relative to the clock, they will appear to be running more slowly. As I understand it, this is just a visual effect, it doesn't mean that processes in the system with clocks are affected by someone observing it from moving frame. I can't imagine any other interpretation of this, cause this would result in all sorts of paradoxes, like what if there are 3 clocks oriented: parallel, perpendicular and at 45 degree relative to direction of moving frame. If you visualise light path from moving frame perspective like it is done in wiki link above, and do analogical interpretation, this would imply that some of the 3 clocks in same frame are running slower and some are faster, depending on orientation.

According to the same wiki page this time dilations are not just visual effect and do change behavior of objects, here is a quote from 2nd paragraph:

Such time dilation has been repeatedly demonstrated, for instance by small disparities in a pair of atomic clocks after one of them is sent on a space trip, or by clocks on the Space Shuttle running slightly slower than reference clocks on Earth, or clocks on GPS and Galileo satellites running slightly faster.

So if we continue our analogy, we can take 4 pairs of atomic clocks, and send 3 of them on a space trip oriented differently, we would get different time results on them.

We can even continue this absurd, and remind "twins paradox", and conclude that the one that was perpendicular to moving frame would become older....

Answer 1

Answer to the title question: absolutely not.

---

Experimental Touchstone

Before we explain in detail, let's begin by noticing that the Michelson-Morley interferometric experiment explicitly tests if orientation affects the clocking behavior of a there-and-back light path. And quite famously the answer is "no". This must be true for any inertial observer.¹

So why do all the introductory materials use a transverse clock?

It's actually a good question and the answer (at least beyond "Well, that's what Einstein did!"), requires taking a close look at the way the explanation would work with a longitudinal clock.

What is Going on, Then?

The short version is easy: because the longitudinal light clock is affected by length contractions as well as time dilation.² And then it follows that from a didactic point of view you want to develop one of the rules (time dilation or length contraction) first, and address the second one separately rather than trying to deal with them at the same time. That makes the transverse clock preferable for intorducing relativity.

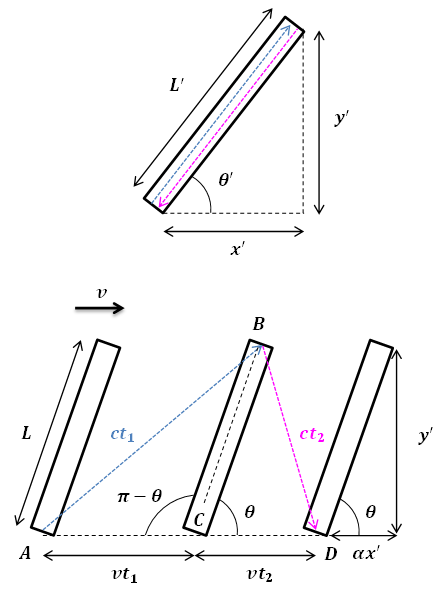
To show this the long way we'll imagine two basically identical light-reflection clocks $\mathbb{c}$ and $\mathbb{C}$, where $\mathbb{c}$ is the traditional transverse clock and $\mathbb{C}$ is aligned longitudinally.³ In their rest frame $S$, each clock has length $l = L$, and consequently identical periods $p = 2l/c$ and $P = 2L/c$. We then consider the behavior of the clocks as observed in frame $S'$ moving at speed $-v$ along the length of $\mathbb{C}$ with respect to $S$.

Transverse case

The analysis of the period of $p'$ of the transverse clock is the traditional one: the time required to complete the trip (out and back) is \begin{align} p' &= \frac{\sqrt{(2l)^2 + (vp')^2}}{c}\\ &= \sqrt{\left(\frac{2l}{c}\right)^2 + \left(\beta p'\right)^2} \\ &= p \sqrt{1 + \left( \beta \frac{p'}{p}\right)^2} \;, \end{align} so that \begin{align} \left(\frac{p'}{p} \right)^2 &= 1 + \left(\beta \frac{p'}{p}\right)^2\\ \frac{p'}{p} &= \left(1 - \beta^2 \right)^{-1/2} \\ &= \gamma\;. \end{align}

Longitudinal case

To find the period $P'$ of the longitudinal clock we have to do a bit more figuring. The elapsed time $T_f$ for the forward going half of the journey is $$ T_f' = \frac{L' + v T_f'}{c} \;,$$ and for the backward going half of the journey the time $T_b$ required is $$ T_b' = \frac{L' - v T_b'}{c} \;.$$ After a little figuring we get the period as \begin{align} P' &= \frac{L'}{c(1 - \beta)} + \frac{L'}{c(1 + \beta)}\\ &= \frac{2L'}{c(1 - \beta^2)} . \end{align} Now, if $L' = L$ this would lead to $$ \frac{P'}{P} = \left( 1 - \beta^2 \right)^{-1} = \gamma^2 \;, \tag{wrong!}$$ meaning the clocks would not agree, but as we said before Michelson-Morley style of experiments rule that out, so $L'$ must not be the same as $L$. To get the agreement we must have it is required that $$ \frac{L'}{L} = \left(1 - \beta^2 \right)^{1/2} \;,$$ the usual expression for length contraction.

Better Way

All that work is, quite frankly, nasty, and I would recommend a geometry first approach as a better alternative to Einstein's version. Get Takeuchi's book, it's worth the money.

---

¹ Because it tells us that two clocks set with their emit/receive ends coincident that beat in time with one another will still beat in time with one another when you swing them around. It doesn't mean that all observer will agree on the frequency of the clocks, just that the two clocks agree.

² That's what Lorentz-Fitzgerald contraction is all about after-all: fixing up the classical theory to match the Michelson-Morley results.

³ We'll continue to use lower case for quantities related to the transverse clock and capitals for quantities related to the longitudinal clock throughout.

Answer 2

Consider 4 identically constructed light-clocks of various orientations that have the same mirror separation when they are at rest. For convenience, let's arrange them in a circle, each with one mirror at a common point. In the rest frame, if the initial signals are emitted by the center at the same event, the reflections off the mirrors are received by the center at the same event.

When this circular light clock is set in uniform motion, the variously-oriented light clocks experience length-contractions... [the circular light clock looks like an ellipse in the lab frame].. in such a way that the reflections are still received by the center at the same event... in accord with the principle of relativity.

Here is a frame from my video https://www.youtube.com/watch?v=AXx3CB80rAk to motivate Length Contraction.

Here is a frame from my video ( https://www.youtube.com/watch?v=tIZeqRn7cmI ) of an animated spacetime diagram of a ticking circular light-clock.

In this video ( https://www.youtube.com/watch?v=NqjAOyGR82s ), I apply this to demonstrate the Clock Effect (related to the Twin Paradox).

Some further details are available in my paper:
"Visualizing proper-time in Special Relativity"
Physics Teacher (Indian Physical Society), v46 (4), pp. 132-143 (October-December 2004) https://arxiv.org/abs/physics/0505134

Answer 3

I would resolve this problem for any arbitrary angle of the light clock inclination to show that the time dilation is independent of the light clock's orientation. If the clock is inclined at an angle $\theta^\prime$ in its rest frame, this angle changes into $\theta$ from the viewpoint of the lab observer WRT whom the light clock moves at $v$ so that we have: [See the attached Figure.]

$$\cos\theta'=\frac{x'}{L'}\space and \space \cos\theta=\frac{\alpha x'}{L} \tag{1&2}$$

Recall that $\alpha$ is the reciprocal of the Lorentz factor. Moreover, we have:

$$\tan\theta'=\frac{y'}{x'}\space and \space \tan\theta=\frac{y'}{\alpha x'} \tag{3&4}$$

Eqs. (1&2) and Eqs. (3&4) repectively imply:

$$\frac{\cos\theta'}{\cos\theta}=\frac{L}{\alpha L'} \space and \space \frac{\tan\theta'}{\tan\theta}=\alpha \tag{5&6}$$

Eqs. (5&6) yield:

$$\frac{L}{L'}=\frac{\alpha/\cos\theta}{\sqrt{1+\alpha^2\tan^2\theta}}=\frac{c\alpha}{\sqrt{c^2-v^2\sin^2\theta}} \tag{7}$$

Now, using the cosines law for $\Delta ABC$, we get:

$$c^2t_1^2=v^2t_1^2+L^2-2vt_1L\cos(\pi-\theta) \rightarrow $$

$$t_1=\frac{v\cos\theta+\sqrt{c^2-v^2\sin^2\theta}}{c^2-v^2}L \tag{8}$$

using the cosines law for $\Delta BCD$, we finally get:

$$c^2t_2^2=v^2t_2^2+L^2-2vt_2L\cos\theta \rightarrow $$

$$t_2=\frac{-v\cos\theta+\sqrt{c^2-v^2\sin^2\theta}}{c^2-v^2}L \tag{9}$$

For $t=t_1+t_2$, we have:

$$t=\frac{2L\sqrt{c^2-v^2\sin^2\theta}}{c^2-v^2}\tag{10}$$

As we know the time measured by the observer in the light clock's rest frame is $t^\prime=2L'/c$, thus we can write:

$$\frac{t}{t'}=\frac{c\sqrt{c^2-v^2\sin^2\theta}}{c^2-v^2}\frac{L}{L'} \tag{11}$$

Substituting Eq. (7) into Eq. (11), we get:

$$\frac{t}{t'}=\frac{1}{\alpha} \tag{12}$$

Therefore, the time dilation is independent of the light clock's orientation.