Let's say I wire the negative pole of a battery to the positive pole. Obviously, the battery will short circuit as the electron pool in the negative side will become attracted to the positive side and cause a huge flow.
However, if I add a lamp to the circuit, the electrons again flow because they are attracted to the positive pole, but for some reason only enough of them to power the lamp. Why don't they keep flowing past the lamp and drain the battery just like a short circuit? Why does the resistance dictate the current?
$\begingroup$
$\endgroup$
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
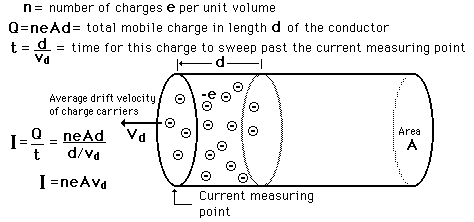
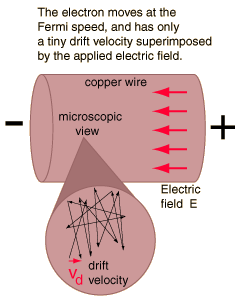
This is a microscopic view of the current:
For many conductors of electricity, the electric current which will flow through them is directly proportional to the voltage applied to them.
When a microscopic view of Ohm's law is taken, it is found to depend upon the fact that the drift velocity of charges through the material is proportional to the electric field in the conductor. The ratio of voltage to current is called the resistance,
A microscopic view of Ohm's law
Why don't they keep flowing past the lamp and drain the battery, just like a short circuit? Why does the resistance dictate the current?
They will eventually drain the battery, but after some time much longer than the short circuit time because the current depends on the resistance which depends on the collisions the electrons have in the material, the larger the number of random collision the larger the resistance, as seen in the links given.
Electrons in conductors are like students running through an empty corridor when the class is let out, or running into a crowd of students partially blocking the way and slowing the flow.
$\begingroup$
$\endgroup$
Why don't they keep flowing past the lamp and drain the battery just like a short circuit?
They do keep flowing past the lamp. Current flows in complete circuits. From one terminal of the battery, through the lamp, and back to the other terminal of the battery.
They don't drain the battery as fast as a short circuit because the quantity of carriers flowing (per second) is less, which we normally just call "the current is smaller".
Why does the resistance dictate the current?
The current is less because the battery has a (roughly) fixed potential difference between its terminals. The lamp has higher resistance than the simple wire and
$$ I = \frac{V}{R},$$
which is just Ohm's law.
If you want to understand why Ohm's law is what it is, read Ohm's Law: Drude Model
answered Dec 26, 2017 at 18:42
$\begingroup$
$\endgroup$
The shortest answer would be Ohms law -> U = R * I
Following your example, let's assume your battery has a voltage of 12V.
If you now simply connect both poles with a piece of wire, you create a short-circuit because the wires resistance is very low - lets say 0.01 Ohm.
Applying Ohms law -> U/R = I -> 12V / 0.01 Ohm = 1200A
1200 Ampere is quite a high current, so the stored energy in the battery will be consumed very fast. (In reality your piece of wire would just burst into flames)
Now if you add a lightbulb to this circuit, things look different.
Lets assume your lightbulb has a resistance(impedance) of 200 Ohm, which would be a legit number in real life.
Going back to Ohms law you get -> 12V / 200Ohm = 0.06 Ampere
Comparing both cases, you get a very big difference in your current:
1200 Ampere to 0.06 Ampere
Now that your current is much smaller when introducing a lightbulb to your circuit, your battery will discharge only slowly .
Also one little correction: The current doesn't actually slow down or speed up, only the amplitude changes.
For the speed in which electrons travel through conductive materials you will have to check out "drift velocity".
