2
$\begingroup$

I am fairly new to plasma physics and am making a project on the Ion Acoustic Wave. We have discussed Landau-damping in a plasma where we took the electrons to have a Maxwellian distribution and considered ions as immobile. The idea of the project is to generalise this to the case where we also take ions into account.

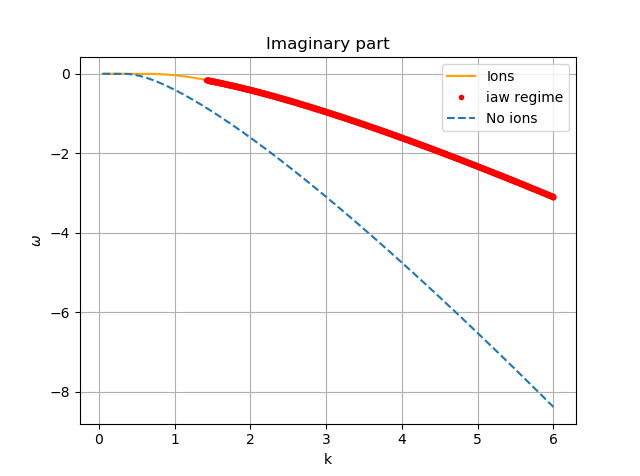
In my attempt to do so, I have used Python to solve the dispersion relation numerically. I find that for a temperature ratio $T_{el}/T_{ion}= 10^4$ and mass ratio $m_{el}/m_{ion}= 1/2$ the damping effect is weaker for the IAW than when the ions were considered immobile. How could I understand this result more intuitively? I was expecting the wave to damp faster as there are now two species absorbing energy from the wave. I also found that for heavier ions, the IAW gives almost identical results as the initial plasma model.

enter image description here

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ The original idea behind IAWs was that $T_{e}/T_{i}$ needed to be greater than 3 otherwise the instability that radiates the waves would be suppressed. In the 1970s and 1980s, several authors (e.g., Christian Dum) did a lot of work on this topic and found that the instability dependence on $T_{e}/T_{i}$ could be reduced or even ignored in the presence of electron heat fluxes, temperature gradients, beams, etc. Note that the original concept is horribly flawed in that we never observe single, isotropic Maxwellians for either ions or electrons in space plasmas. $\endgroup$
    – honeste_vivere
    Commented Dec 14, 2017 at 13:29
  • 1
    $\begingroup$ Thanks! This explains why the influence seen in my figures is almost negligible! $\endgroup$
    – Plasmaths
    Commented Dec 14, 2017 at 18:53
  • 1
    $\begingroup$ I missed something the first time I read this. How do you get an IAW with immobile ions? IAWs are like Langmuir waves but where the ions oscillate in a longitudinal fashion. The electrons serve to counter the ion oscillations to prevent charge accumulation. If just the electrons oscillate, then the real part of the frequency would be near fpe not fpi. These would damp differently than an IAW too. $\endgroup$
    – honeste_vivere
    Commented Nov 24, 2020 at 15:11

1 Answer 1

Reset to default
1
$\begingroup$

This all depends on where you are in the the dispersion relation. The condition you have given is for cold ions, and thus our dispersion relation looks something like the following for ion-acoustic waves:

enter image description here

(Sorry for the crude drawing). There are two key observations here:

  • The wave $\omega/k$ is in the tail of the dispersion relation of the ions (since $v_{thi}\lt\lt \omega/k$ for ions in ion-acoustic waves).
  • The wave is near the maximum of the electron distribution (since $v_{the}\gt\gt \omega/k$ for electrons in ion-acoustic waves).

This means that the gradient of both dispersion relations is small at $v=\omega/k$ which means that the number of electrons (ions) with velocities moving slightly faster then the wave is approximately equal to the number of electrons (ions) moving slightly slower. The wave does work on these slower electrons and has work done on it by the faster electrons. Since they are approximately equal in number the net transfer of energy from the wave to the resonant particles is small and there is consequently little damping.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Nice explanation, thank you. But assuming that the temperature of the electrons is kept identical in the IAW model as in the model where the ions are considered immobile, why does the damping effect seem to decrease? I would expect that, since the ions can now also contribute to the damping, albeit not very much, the opposite effect would make more sense? $\endgroup$
    – Plasmaths
    Commented Dec 14, 2017 at 18:08
  • $\begingroup$ @Plasmaths What frequencies and wavenumbers are you looking at in both models? Do they actually satisfy the assumptions for IAW? $\endgroup$
    – Quantum spaghettification
    Commented Dec 14, 2017 at 18:22
  • $\begingroup$ I have added a figure - $k$ in units Debye length $\endgroup$
    – Plasmaths
    Commented Dec 14, 2017 at 18:51

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.