This all depends on where you are in the the dispersion relation. The condition you have given is for cold ions, and thus our dispersion relation looks something like the following for ion-acoustic waves:
[![enter image description here][1]][1]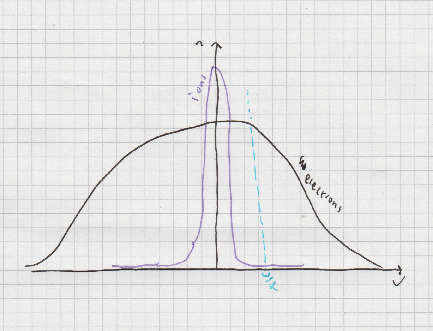
(Sorry for the crude drawing). There are two key observations here:
- The wave $\omega/k$ is in the tail of the dispersion relation of the ions (since $v_{thi}\lt\lt \omega/k$ for ions in ion-acoustic waves).
- The wave is near the maximum of the electron distribution (since $v_{the}\gt\gt \omega/k$ for electrons in ion-acoustic waves).
This means that the gradient of both dispersion relations is small at $v=\omega/k$ which means that the number of electrons (ions) with velocities moving slightly faster then the wave is approximately equal to the number of electrons (ions) moving slightly slower. The wave does work on these slower electrons and has work done on it by the faster electrons. Since they are approximately equal in number the net transfer of energy from the wave to the resonant particles is small and there is consequently little damping. [1]: https://i.sstatic.net/wp5hu.png
