Acoustic wave incident to pipe wall

Question

I would like to consider the sound incident to a water filled pipe wall. I think the pipe wall is typically considered as a rigid wall boundary, it means all the incident wave is reflected. Is this assumption is correct in real world? I think if the sound inside the pipe is large, we can hear the sound from outside the pipe. In practical, what kind of boundary condition should be assumed for the pipe wall?

Answer 1

Introduction

At every interface (change of medium, or better density) there is some reflection. This has to do with the change in the impedance “seen” by the impinging wave.

It is safe to assume that this will be the behaviour of real systems but you have to keep in mind that the models used in such cases are approximations with many parameters either assumed to be known (with unknown error compared to their true value) and many simplifications.

Direction towards a possible solution

As you already stated in a comment, the impedance discontinuity at the interface can be used to estimate the reflected and transmitted energy/wave. Without going into the derivation (you can find more information in various sources online or in textbooks about acoustics), the reflection coefficient is given by

$$ R_{e} = \left( \frac{Z_{2} - Z_{1}}{Z_{2} + Z_{1}} \right)^{2} \tag{1} \label{1} $$

where $Z_{1}$ is the acoustic impedance of the medium where impinging (to the interface) sound wave is travelling and $Z_{2}$ is the acoustic impedance of the medium in which the transmitted wave is travelling.

$R_{e}$ is an energy-based metric. In this link, it is stated that the amplitude reflection coefficient is given by

$$ \begin{align} R_{a} & = \frac{Z_{2} - Z_{1}}{Z_{2} + Z_{1}} \tag{2.a} \label{2.a}\\ R_{a} & = \frac{Z_{1} - Z_{2}}{Z_{2} + Z_{1}} \tag{2.b} \label{2.b} \end{align} $$

Which coefficient to use depends on the quantity of interest and whether this quantity is on the numerator or the denominator of the impedance formula. For quantities on the numerator, such as voltage or force you have to use equation \eqref{2.a} while for quantities such as velocity or current equation \eqref{2.b}.

Please note that you have to treat this index carefully. $R_{a}$ shows what the maximum amplitude of the reflected wave will be but you have to keep in mind that the amplitude can be discontinuous at the interface so treat it with caution.

You can calculate the transmitted energy from equation \eqref{1} as

$$ T_{e} = 1 - R_{e} = 1 - \left( \frac{Z_{2} - Z_{1}}{Z_{2} + Z_{1}} \right)^{2} = \frac{4 Z_{1} Z_{2}}{\left( Z_{1} + Z_{2} \right)^{2}}\tag{3} \label{3} $$

Similarly, you can calculate the transmitted amplitude from equations \eqref{2.a} and \eqref{2.b} as

$$ \begin{align} T_{a} & = \frac{2 Z_{2}}{Z_{1} + Z_{2}} \tag{4.a} \label{4.a}\\ T_{a} & = \frac{2 Z_{1}}{Z_{1} + Z_{2}} \tag{4.b} \label{4.b}\\ \end{align} $$

Remarks

It is important to note that these formulas are for normal incidence and do not provide good results for inclined incidence with the errors increasing with the angle of incidence. This means that they can be quite good approximations for plane waves travelling perpendicular to an interface. Nevertheless, it is a good starting point to get a first estimate of what would be expected and these formulas can, more often than not, provide some decent results for low frequencies where only plane waves are supported in confined places.

Multiple interfaces

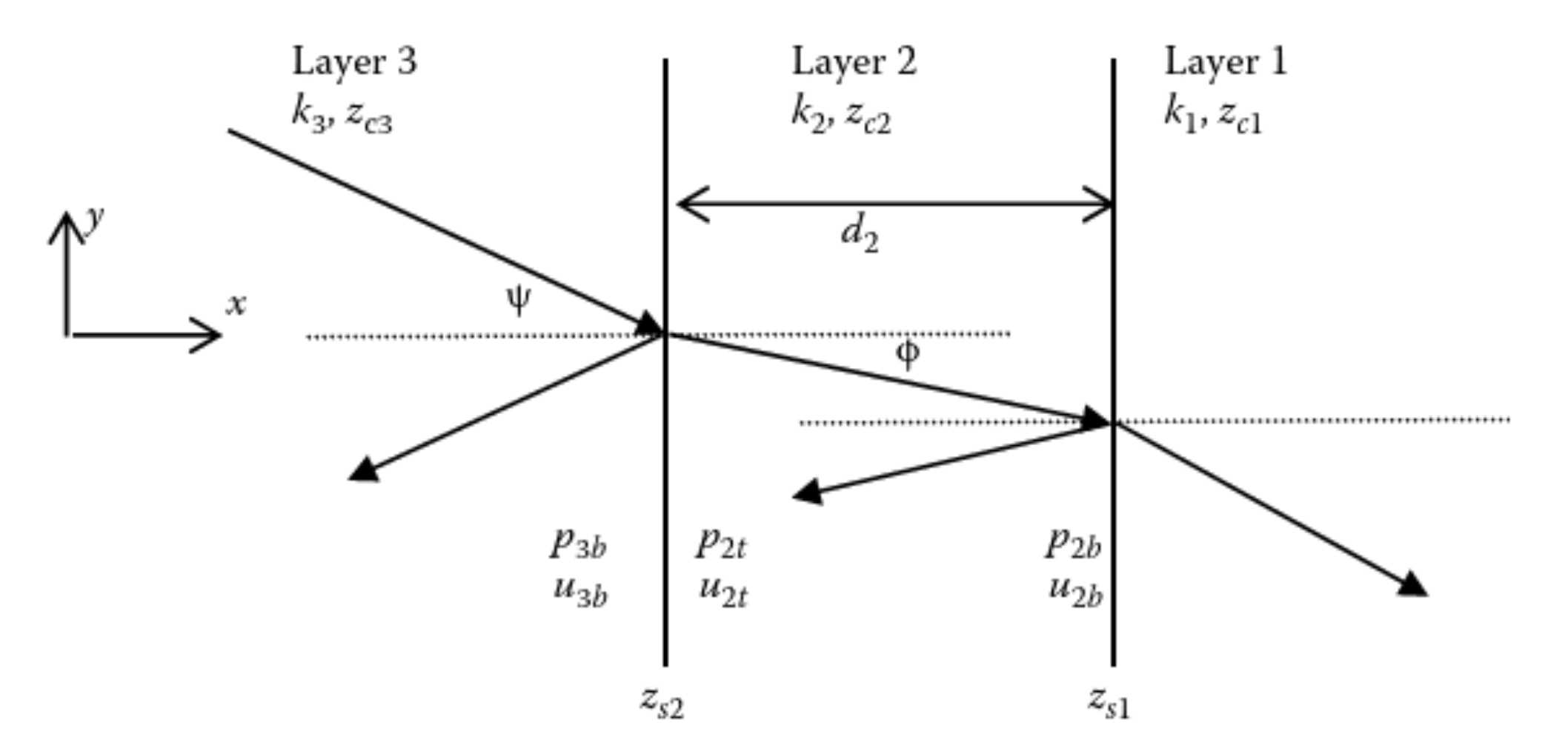
If you are dealing with multiple interfaces one after the other (like in a sandwich panel or from your water pipe, into the wall, then from the wall to the air outside) you have to take into account the reflections between the two interfaces too. The diagram below (from "Acoustic Absorbers and Diffusers: Theory, Design and Application" by Trevor Cox and Peter D’ Antonio) shows exactly this case.

Referring to the image, the interface that the sound wave impinges on is the leftmost and has a surface impedance $Z_{s_{2}}$. The wave number on the $x$ axis (the $x$ component of the wavevector) is $k_{x_{3}}$ and $Z_{c_{3}}$ denotes the characteristic impedance of the travelling medium (for example, for air, this is $\approx 425 \, \text{rayl}$, but for many media it is complex). From the same source (as the image), the impedance “felt” by the impinging wave can be expressed as

$$ Z_{s_{2}} = \frac{-\text{j} Z_{s_{1}} Z_{c_{2}} \frac{k_{2}}{k_{x_{2}}} \cot \left( k_{x_{2} d_{2}} \right) + \left( Z_{c_{2}} \frac{k_{2}}{k_{x_{2}}} \right)^{2}}{Z_{s_{1}} - \text{j} Z_{c_{2}} \frac{k_{2}}{k_{x_{2}}} \cot \left( k_{x_{2} d_{2}} \right)} \tag{5} \label{5} $$

where $\text{j}$ denotes the imaginary unit for which $\text{j}^{2} = -1$ is true and the rest of the quantities can be deduced directly from the image above.

Update - Clarification

As Michael M very well commented, the solutions provided in this answer apply to the case that the interface is flat. Furthermore, since in the formulation of the problem, an infinite interface is assumed, for practical applications the wavelength has to be shorter than the shortest dimension of the interface (this will be finite in dimension in reality).

Without any proof, based solely on intuition and the fact that it is widely accepted that most phenomena in acoustics have a direct or indirect dependence on the relation of the wavelength and the dimensions of the system, I believe that if the curvature of the interface is small the equations provided above could provide a rather good approximate solution (for engineering purposes mostly) to the actual phenomenon. Of course, if the curvature is considerable in the area of interaction (again, this is much like a problem of locally reacting interface, or equivalently, "how much” the assumption of an interface with infinite dimensions is violated) refinement of the solution is necessary.