0
$\begingroup$

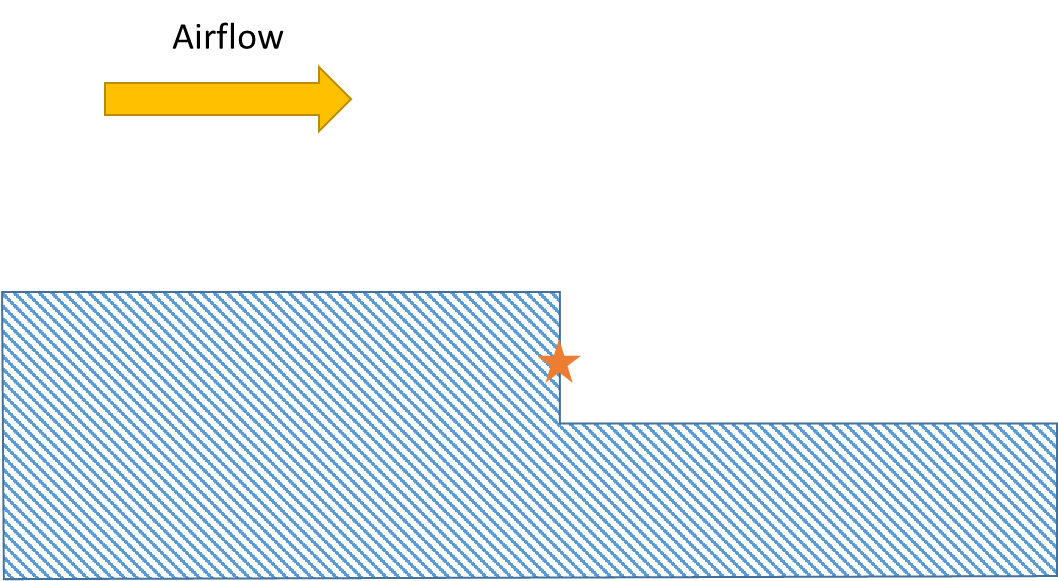
I've been having quite a heated debate with a friend of mine on a subject that seems so simple but we can't find a definitive agrement.

Illustration of the problem

In the case of a backwards facing step, a lower pressure zone is created behind the step. On the backward facing face, the pressure coefficient (P-Pinf)/Qinf (Pinf: farfield pressure; P: local pressure: Qinf: farfield dynamic pressure) is negative. So far we both agree.

In the figure describing the problem, the step is considered solid and infinite in all directions Where we disagree is here: What is the direction of the aerodynamic force on the step?

Two possibilities:

  • The load is a pressure load and therefore is applied on the face of the step resulting in a load towards the left of the figure (sure the pressure is less than the atmospheric pressure but it still "pushes" on the wall and therefore towards the load is towards the left).
  • The load is a function of (P-Pinf) in which case it is directed towards the right of the figure (in this case the depression tries to "suck" the step towards the right).

Any help would be quite appreciated. All the better if some sort of source material is available :)

Thanks a lot

Improve this question
$\endgroup$

4 Answers 4

Reset to default
0
$\begingroup$

In the figure describing the problem, the step is considered solid and infinite in all directions

I presume that the solid body extends infinitely in the leftward and rightward directions (in addition to the direction perpendicular to the plane of drawing). In that case the only vertical face in the entire solid body is that of the step. So the only horizontal force on the solid body is that due to pressure acting on that vertical face of the step. Since $p>0$ the solid body experiences a leftward force on it. The fact that $p-p_\infty<0$ is irrelevant; it only says that the leftward force acting on the solid body is less than would be the case if we assumed the pressure in the vicinity of the step to be equal to far-stream pressure $p_\infty$.

If however the solid body were finite in the leftward direction then a second step would form there and thus a second vertical face would be present for action of pressure. On this vertical face the pressure would be higher than $p_\infty$, and then the body experiences a net rightward force.

P.S. As far as I know, the technique of calculating force on the body due to a pressure field by first subtracting a uniform pressure everywhere on the surface of the body works only for finite closed bodies (or control volumes). This does not therefore apply to your case, i.e. you are not allowed to integrate $p-p_\infty$ over the body's surface to obtain the load on it.

Improve this answer
$\endgroup$
0
$\begingroup$

you are describing flow separation drag, which acts against the direction of travel. If you place a pressure sensor in the vicinity of the step during flow, it will read slightly below atmospheric pressure. that pressure difference times the area of the step is a first order approximation to the size of the drag force created by the flow separation.

Improve this answer
$\endgroup$
0
$\begingroup$

Even though the absolute pressure in the low pressure zone is less than atmospheric, it is still acting to the left. However, that being said, what you are not accounting for are the pressure forces acting on the remainder of the structure. If, over the remainder of the surface, the pressure were the far-field pressure, the net pressure force on the overall structure would be to the right. From the standpoint of gauge pressure, you would say that there are no other pressure forces on the remainder of the structure and only suction behind the step (and a net force to the right). But, from the standpoint of absolute pressure, you would say that there are pressure forces acting over the entire structure, but lower pressure behind the step, and, again, a net pressure force to the right. So both approaches give the same answer.

Improve this answer
$\endgroup$
0
$\begingroup$

My take on it is that

  • yes, there is flow separation as has been pointed out (the flow would reattach towards the right)
  • there is a vortex created next to the step. It is clockwise and time varying (probably oscillating a bit)
  • the vortex applies an upward shear force on the step face and a leftward shear force on the lower step
  • the pressure on the step face is approximately constant in time, acting towards the left but higher at the bottom than near the top.
Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.