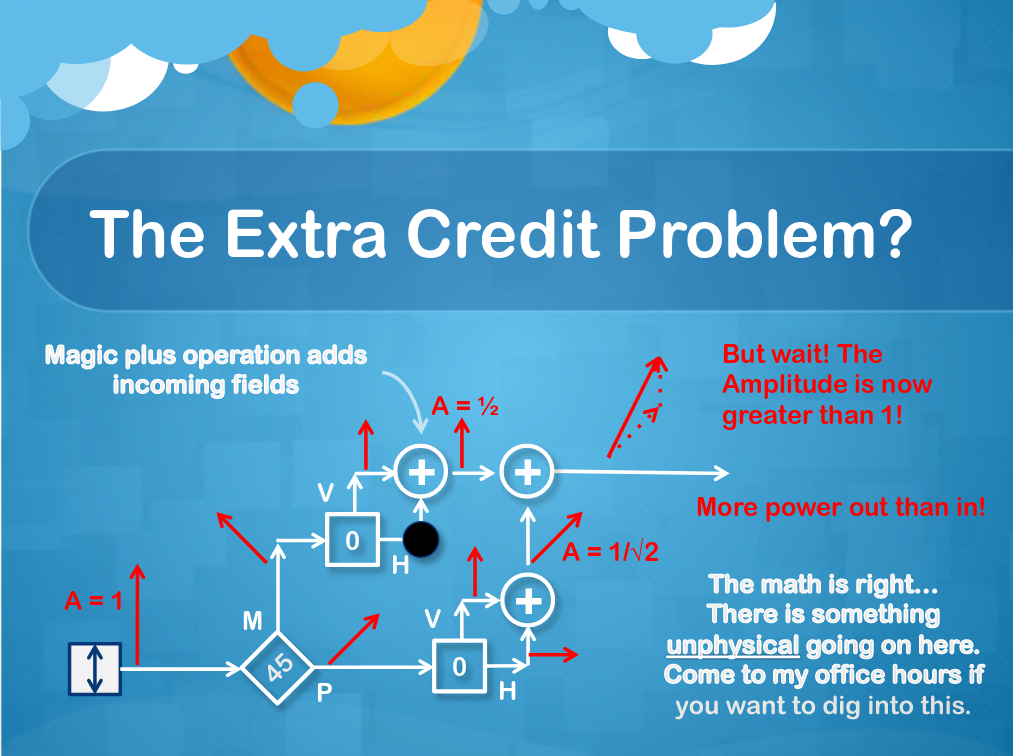
I am going through the MIT OCW course on Quantum Physics and while looking at the recitations slide on polarizers I got stuck on the problem given in the second last slide (the extra-credit problem):
The squares in the above image are polarizing beam splitters (splits the incoming beam into two beams with perpendicular polarization). The vectors in red indicate the direction of the E-field and not the direction of propagation of the wave.
I understand that they have used mathematical sleight of hand. Essentially by writing the zero-valued horizontal component as $+\frac{1}{2}$ and $-\frac{1}{2}$ then selectively filtering out one component they are able to get a non-zero horizontal component in the end. Hence, output power is more than input power.
But what is the unphysical thing that is going on? I can't for the life of me figure out that part.
EDIT: Added description about the diagram.