Suppose we have a pointed electric charge or a bipolar magnet. If we put a massive gravity source nearby, will the magnetic and electric fields be distorted? In what way?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ Please award your bounty to either Andrew Steane’s answer or my answer. $\endgroup$– G. SmithCommented Nov 12, 2018 at 17:25
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
5
Yes, electromagnetic fields are distorted by gravity.
In our current theory of gravity (Einstein’s General Relativity), gravity is explained as spacetime curvature. Maxwell’s equations can be reformulated in curved spacetime, so one can, for example, study the electric field of a static point charge outside a black hole. There is even an exact analytic solution to this electrostatics problem, at least in the simplest case of a Schwarzschild black hole.
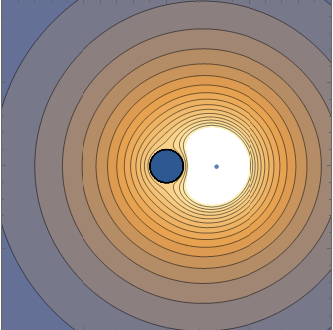
The following contour plot shows the electrostatic potential when the charge is at radius $6M$ outside a black hole of mass $M$. The horizon is at $2M$. (I'm using geometric units where $G=c=1$.) The blue circle is the black hole. (Sorry, I couldn't figure out how to change the color in Mathematica.) The blue dot is the charge. The contour lines represent equally-spaced values of the potential. The white area is where the contours get too close together to be plotted without overlapping.
The field of the charge looks quite different when a black hole is nearby, compared to the simple radial field that the charge would have with no black hole present.
One interesting feature of the solution is that the horizon of the black hole is a equipotential surface for the electrostatic potential. A surface of slightly greater potential (in the case of a positive charge) avoids the hole by wrapping around it on the side towards the charge. A surface of slightly less potential wraps around the hole on the side away from the charge.
Put differently, the black hole bends the electric field lines diverging from the charge so that at the horizon of the hole they are actually perpendicular to the horizon. Unfortunately, I don't have a plot of the field lines but you can envision them at right angles to the contour lines.
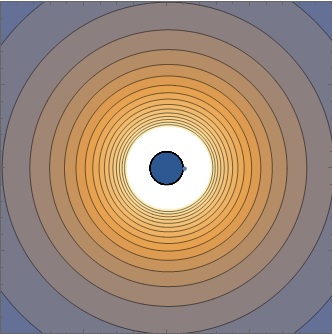
A second interesting feature is that as the position of the charge approaches the horizon, the field becomes centered on the hole, not on the charge! The following plot shows the potential when the charge is at $2.2M$.
The most interesting feature is that the gravitational bending of the field lines means that they don’t diverge from the charge symmetrically in all directions like they do in flat spacetime. Near the charge, the field is stronger in the direction away from the hole than in the direction toward the hole. This asymmetry results in the point charge experencing a gravitationally-induced electrostatic “self-force” away from the black hole.
I expect that similar effects occur with magnetostatic fields, although I have not seen these calculations.
Physicists have also theoretically studied the scattering and absorption of electromagnetic waves by black holes. If gravity did not distort the fields, there would be no electromagnetic scattering by the black hole.
-
$\begingroup$ "the field is stronger in the direction away from the hole than in the direction toward the hole" - are u sure? the image in the other onswer show the opposite (as the lines denser closer to the horizon). $\endgroup$– AnixxCommented Nov 8, 2018 at 13:12
-
1$\begingroup$ @Anixx I am sure that the self-force on the charge -- when measured in a local, freely-falling frame momentarily at rest relative to the charge -- is repulsive. See journals.aps.org/prd/abstract/10.1103/PhysRevD.22.1276. $\endgroup$– G. SmithCommented Nov 8, 2018 at 23:35
-
$\begingroup$ Thanks for the reference to Smith and Will. I note from the abstract the phrase "after mass renormalization". The largest part of the self-force is the inertial part, i.e. the part that can be accounted for by adjusting the mass. This part acts to oppose the acceleration of the charge relative to a local inertial frame, in other words towards the hole in this example (I assume the figure shows a charge at fixed distance from the hole). I expect the repulsive force you mention is the next term. (This is my quick reaction; I will check). $\endgroup$ Commented Nov 8, 2018 at 23:54
-
$\begingroup$ It's the next term in the sense that it's the finite part left over after an infinite term is absorbed by mass renormalization. I assume a similar calculation for your case of a uniform gravitational field would produce the Abraham-Lorentz force? Do you have any idea why the self-force in the uniform-field case is toward the "source" of the gravity and in the black hole case away from it? $\endgroup$– G. SmithCommented Nov 9, 2018 at 0:06
-
$\begingroup$ Yes the self-force would include the one named after Abraham and Lorentz, but it also includes the inertial term which diverges in the point limit. When you look at the field lines it is this inertial (infinite) term which stands out and is opposed to the acceleration. The remaining terms could be in either direction depending on the particular case. The complete series for my example is given in journals.aps.org/prd/abstract/10.1103/PhysRevD.91.065008 and the leading term after the diverging one is indeed of opposite sign, so AWAY from the horizon, just like black hole case. $\endgroup$ Commented Nov 14, 2018 at 11:38
$\begingroup$
$\endgroup$
5
Yes they are distorted. The simplest example is a static point charge in a modest gravitational field. Then we can calculate the electric field by using the fact that it is the same as the field observed in an inertial frame for a charge undergoing motion at constant proper acceleration relative to that frame. This can be calculated using Maxwell's equations in flat spacetime. Here it is:
This image shows the electric field for a charge undergoing motion at constant proper acceleration in the upward direction, in flat spacetime, as observed in the inertial frame where the charge is momentarily at rest. At this moment the B field is zero. The same image also shows the electric field for a charge held at a fixed position in a modest gravitational field directed in the downwards direction on the diagram.
Thus we can observe the easily-remembered fact that gravity causes the field lines to "droop".
answered Nov 5, 2018 at 16:31
-
$\begingroup$ What is the horizontal line at the bottom, which looks like a field line going both left and right? And what is the significance of the annotation $x=-ct$? $\endgroup$– G. SmithCommented Nov 5, 2018 at 17:23
-
$\begingroup$ Oh yes; I forgot to say. This is where, in the case of the uniformly accelerating charge, the field takes the form of an intense pulse, somewhat like a shock-wave. It is at the limit of the forward light-cone of points on the past worldline of the particle. When we adopt the uniformly accelerating reference frame in which the particle is at rest, this is the location of a horizon. So not such a modest gravitational field after all! $\endgroup$ Commented Nov 5, 2018 at 17:29
-
$\begingroup$ It’s interesting that, as in the black hole case, the field lines are perpendicular to the horizon. (Or at least they appear to be in the diagram.) Do you know if there is a simple explanation for why this happens in general? $\endgroup$– G. SmithCommented Nov 5, 2018 at 17:41
-
$\begingroup$ I agree that that is interesting, and my intuition is that it should be possible to give a simple explanation, for example by looking at the transformation of the em field tensor as one approaches a horizon. $\endgroup$ Commented Nov 5, 2018 at 19:23
-
$\begingroup$ Has this been measured by experimentation? $\endgroup$ Commented Aug 9, 2019 at 13:11