Yes, electromagnetic fields are distorted by gravity.
In our current theory of gravity (Einstein’s General Relativity), gravity is explained as spacetime curvature. Maxwell’s equations can be reformulated in curved spacetime, so one can, for example, study the electric field of a static point charge outside a black hole. There is even an exact analytic solution to this electrostatics problem, at least in the simplest case of a Schwarzschild black hole.
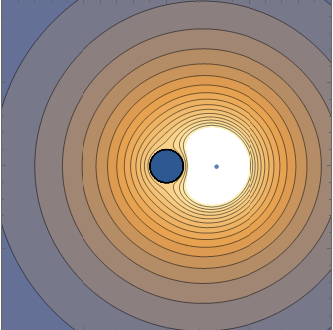
The following contour plot shows the electrostatic potential when the charge is at radius $6M$ outside a black hole of mass $M$. The horizon is at $2M$. (I'm using geometric units where $G=c=1$.) The blue circle is the black hole. (Sorry, I couldn't figure out how to change the color in Mathematica.) The blue dot is the charge. The contour lines represent equally-spaced values of the potential. The white area is where the contours get too close together to be plotted without overlapping.
The field of the charge looks quite different when a black hole is nearby, compared to the simple radial field that the charge would have with no black hole present.
One interesting feature of the solution is that the horizon of the black hole is a equipotential surface for the electrostatic potential. A surface of slightly greater potential (in the case of a positive charge) avoids the hole by wrapping around it on the side towards the charge. A surface of slightly less potential wraps around the hole on the side away from the charge.
Put differently, the black hole bends the electric field lines diverging from the charge so that at the horizon of the hole they are actually perpendicular to the horizon. Unfortunately, I don't have a plot of the field lines but you can envision them at right angles to the contour lines.
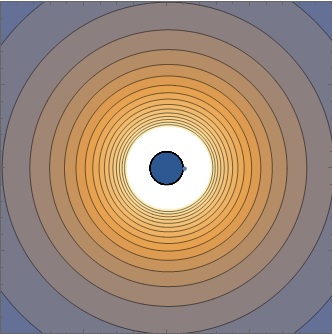
A second interesting feature is that as the position of the charge approaches the horizon, the field becomes centered on the hole, not on the charge! The following plot shows the potential when the charge is at $2.2M$.
The most interesting feature is that thisthe gravitational bending of the field lines means that they don’t diverge from the charge symmetrically in all directions like they do in flat spacetime. Near the charge, the field is stronger in the direction away from the hole than in the direction toward the hole. This asymmetry results in the point charge experencing a gravitationally-induced electrostatic “self-force” away from the black hole.
I expect that similar effects occur with magnetostatic fields, although I have not seen these calculations.
Physicists have also theoretically studied the scattering and absorption of electromagnetic waves by black holes. If gravity did not distort the fields, there would be no electromagnetic scattering by the black hole.