7
$\begingroup$

I've been taught that if a point-sized object is placed between two plane mirrors at an angle theta with each other, then the number of images formed is $360^{\circ}/\theta$ or $360^{\circ}/\theta - 1$, depending on whether $360^{\circ}/\theta$ is even or odd.

Moreover, if $360^{\circ}/\theta$ is non-integral, we simply floor the value. So if, say, the angle between the mirrors is $65^{\circ}$, we will get ${\rm floor}\,(360/65) = 5$ images.

However, on actually drawing the figure, I'm easily able to obtain 6 images, and probably more too.

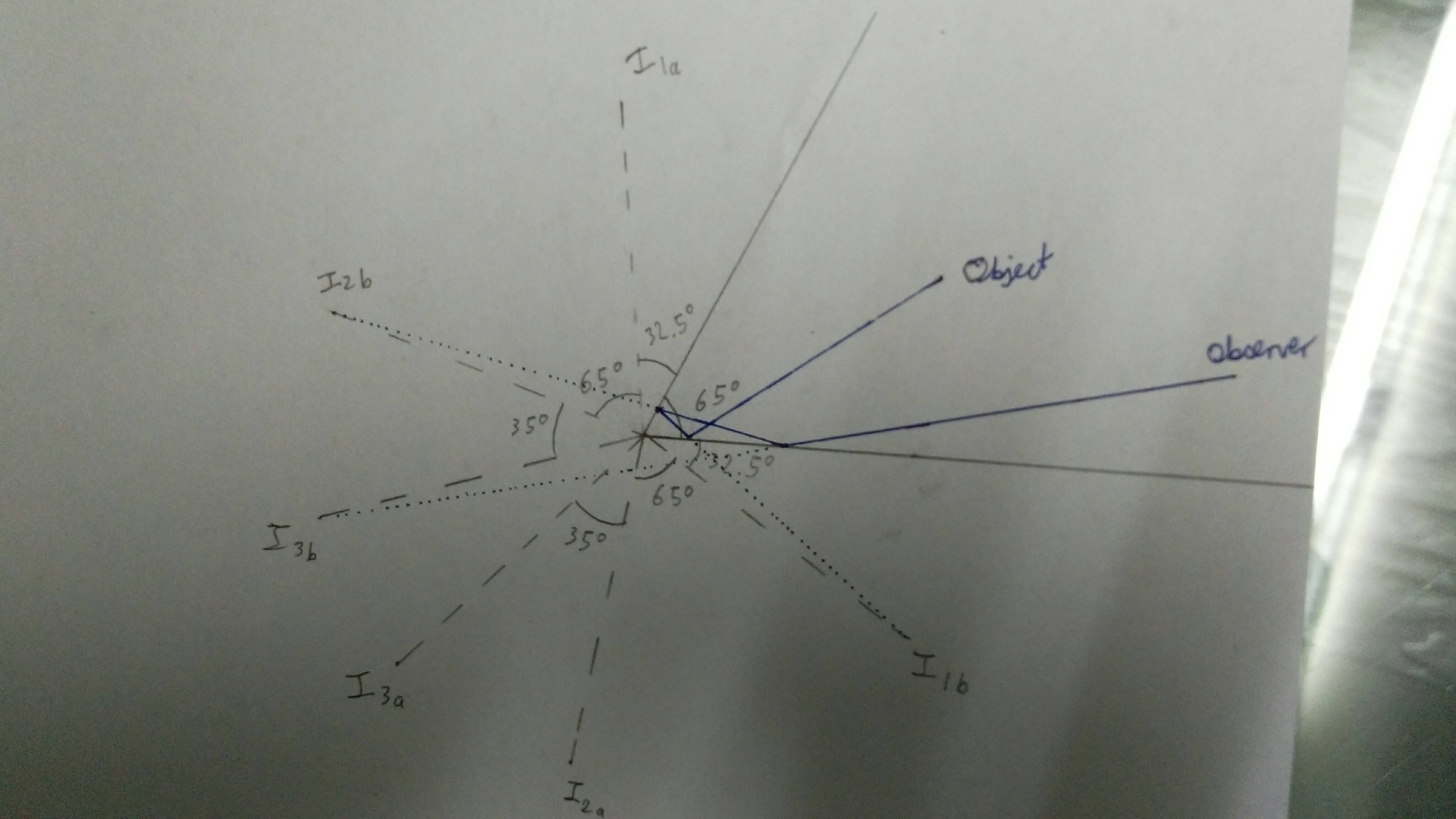Plane mirrors at <span class=$65^{\circ}$ with 6 images" />

If the formula is erroneous, what is the correct formula?

P.S. This is definitely not a homework question. Even some books I've seen have published the formula ${\rm floor}\,(360/65)$.

Improve this question
$\endgroup$
2
  • $\begingroup$ Try making the ray diagrams with the help of compass, it helped me get accurate ray diagrams. $\endgroup$
    – Amsterdam6483
    Commented Mar 26, 2021 at 9:45
  • 1
    $\begingroup$ That might work, but I'm looking for something more mathematical. $\endgroup$
    – harry
    Commented Mar 26, 2021 at 10:21

3 Answers 3

Reset to default
0
$\begingroup$

No. of images formed by 2 inclined mirrors: $n=\displaystyle{\frac{360^o}{\theta}-1}$

Exceptions:

when $~\displaystyle{\frac{360^o}{\theta}}$ is an odd integer and the object is placed asymmetrically, $~n=\displaystyle{\frac{360^o}{\theta}}$

when $~\displaystyle{\frac{360^o}{\theta}}~$ is not an integer, $~~n=\displaystyle{\Bigg[\frac{360^o}{\theta}\Bigg]}$

where [ ] denotes the greatest integer function.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Source: Understanding Physics - Optics and Modern physics by D.C.Pandey. ISBN:9788188222135 Page No. 86 $\endgroup$
    – Amsterdam6483
    Commented Mar 26, 2021 at 9:41
  • $\begingroup$ The pic in OP's question has 6 images resolved. $\endgroup$
    – harry
    Commented Mar 26, 2021 at 10:23
  • $\begingroup$ The greatest integer function doesn't always hold true even if $ \frac{360°}{\theta} $ is a real number with a nonzero decimal part. I will explain that in my answer. $\endgroup$
    – ThePhysicist
    Commented Jul 15, 2021 at 23:28
0
$\begingroup$

All the images formed by two inclined mirrors ($\theta$), of an object placed between them, are formed on a circle centred at the point of intersection of the two mirrors and with a radius equal to the distance of the object from the centre.

The first pair of images will be the direct pair and the angular separation between them will be $2\theta$.

Now there will be cross-forming of images from these direct images.

The separation between the second pair of the images will be $4\theta$ and so on.

For the $n^{th}$ pair, the separation will be $2n\theta$, only if $2n\theta\leq 360^\circ$.

If $2n\theta=360^\circ$, then out of the $2n$ images, the final pair will act as one image. Thus, the total number of images will be $\frac{360^\circ}{\theta}-1$.

When $2n\theta$ exceeds $360^\circ$, then the final pair won't form. Only the $(n-1)^{th}$ pair will form, thus $2n-2$ images in pair. Now, whatever space is remaining on the circle of images, can be utilized by one mirror or the other to form a single image.

The complete mathematical analysis can be found here. https://thephysicist.in/understanding-inclined-plane-mirrors/

Improve this answer
$\endgroup$
0
$\begingroup$

Actually only 5 images will be formed if more images are drawn they will overlap the existing ones if drawn with perfect geometry.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Would you care to provide some more information on the way you reached this conclusion and how the person who posted the question can follow your approach to do the same? $\endgroup$
    – ZaellixA
    Commented May 16, 2022 at 9:13

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.