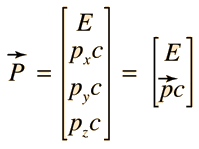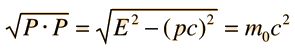Does mass of universe at the moment of big bang changes compared to present day? [duplicate]

Question

Empty space isn't empty as it can contain energy in terms of quantum fluctuation, so since inflation I like to know whether if the present day universe has put on weight?

Answer 1

We should start with the usual reminder that the vacuum doesn't fluctuate and it isn't full of pairs of virtual particles. See Are vacuum fluctuations really happening all the time? for more on this.

However there is an energy associated with the vacuum state of a quantum field, and this energy density, i.e. energy per cubic metre, doesn't change as the universe expands. That means the the expansion of space does produce energy out of nowhere.

Well ... sort of.

The problem is that if the universe is infinite, which it may well be, then it makes no sense to talk about total energy because you can't do arithmetic with infinity. So your question has the universe put on weight doesn't have any simple answer.

And there are other complicating factors. If we do a naive calculation of the vacuum energy we end up with such a large number that it would have blown up the universe in a tiny fraction of a second and we wouldn't be here now. That means the vacuum energy cannot contribute to gravity - either that or our calculation of the vacuum energy is totally wrong. But there does appear to be some vacuum energy that does contribute to gravity, and this is the dark energy that you've probably heard of.

So I think we'd have to say that right now we don't really understand what the situation is with vacuum energy and so we can't answer your question.

Answer 2

so since inflation I like to know whether if the present day universe has put on weight?

From the title you mean mass and not weight as in the question , which I suppose is a humorous one.

One has to define mass. Mass in special relativity is the length of the four vector

The length of the energy-momentum 4-vector is given by

The length of this 4-vector is the rest energy of the particle. The invariance is associated with the fact that the rest mass is the same in any inertial frame of reference.

Discussing a flat universe, where special relativity holds, the mass of the invariant mass of the universe is the length of the summed four vectors of all the constituents, particles and radiation. As the name implies this mass is invariant.

Now as discussed here, the question of whether the universe is flat or curved in some way is an open research question.

If curved anything goes as far as masses go, though people are still trying to get a mass estimate for the universe.

So if a Big Bang model is used, one cannot answer if at the beginning there was a possible times where the universe was curved. Only after reaching flatness, or approximate flatness one can talk of conserved invariant mass of the universe, which is consistent with our observations at the moment.

In reality, determining the value of the density parameter and thus the ultimate fate of the universe remains one of the major unsolved problems in modern cosmology. The recently (June 30, 2001) launched MAP mission will be able to measure the value definitively within the next 5 years.

See also the answer here. .

Edit after comment by MBN

This article in wikipedia on mass in general relativity shows that all of the above discussion is on shaky ground, as there are many possible mass definitions in GR, which are still under research.

So my generalizing the concept of invariant mass to the total four vector of the universe is on shaky ground, even for flat spaces, as time also enters the frame and is constrained only locally. So take the above with many grains of salt. You can keep that the mass density of the universe is a measurable goal.