Variation of angle of minimum deviation with prism angle

Question

The equation for $D_{min}$ is, $D_{min} = 2i - A$ ($D_{min}$is angle of minimum deviation, $i$ is angle of incidence, and $A$ is prism angle)

But as $i$ changes with $D_{min}$ and $A$, I'm not sure if it's possible to plot this on a graph. (Or at least i don't know how to plot this on a graph).

The equation,

$n = \frac{\sin\frac{A+D_{min}}{2}}{sin \frac{A}{2}}$ ; (n is refractive index)

has only 2 variables, so can this be plot on a graph, and if so what is the graph?

Edit: I think I wasn't clear enough. What i mean is Dmin depends on A and i is fixed for Dmin and A of a specific prism and i for Dmin changes when A changes. So what I'd like to know is the variation of Dmin with the angle of prism, like the curve that is obtained if Dmin is plotted on the y-axis and A is plotted on the x-axis.

Answer 1

In above Figure-01 we see the graphs of the function of the minimum deviation angle $\:\delta^*(\mathrm A)\:$ for various values of the relative refractive index $\:n=n_2/n_1\:$.

As shown also in Figure-01 this function is⁽¹⁾ \begin{equation} \delta^{*}\left(\mathrm A\right)=2\cdot\arcsin\left[\left(\dfrac{n_{2}}{n_{1}}\right)\sin\left(\dfrac{\mathrm A}{2}\right)\right]-\mathrm A \tag{01} \end{equation}

Note that to each $\:n$-graph there corresponds a maximum value of $\:\mathrm A\:$ and from this a maximum $\:\mathrm p(\mathrm A)\:$ (for the given $\:n\:$) of the minimum deviation angle $\:\delta^*\:$ where the graph stops. This must be expected since the argument $\:n=n_2/n_1\sin(\mathrm A/2)\:$ of the function $\:\arcsin\:$ in equation (01) as representing the $\:\sin\:$ of an angle must be less or equal to 1 \begin{equation} \left(\dfrac{n_{2}}{n_{1}}\right)\sin\left(\dfrac{\mathrm A}{2}\right)\le 1 \tag{02} \end{equation} If case that this argument is 1 we have \begin{equation} \mathrm p(\mathrm A)=2\cdot\arcsin\left(1\right)-\mathrm A=2\cdot 90^{o}-\mathrm A=180^{o}-\mathrm A \tag{03} \end{equation} with graph a straight line as shown in Figure-01.

---

^{(1) See equation (12) in my answer here : Analytic solution for angle of minimum deviation? In Figure-02 below we have the configuration of the minimum deviation angle.}

---

^{(2) Related also : Why does the graph of deviation angle in a prism doesn't get a symmetry?}

Answer 2

For a given prism, it's refractive index and prism angle are constant.

Minimum deviation for a prism is that case when the deviation is minimum. This case occurs when the incident angle is equal to the emergent angle. Hence, for a prism minimum angle of deviation is constant. Well, a prism cannot have two minimum deviation angles.

But as $i$ changes with $D_{min}$ and $A$, I'm not sure if it's possible to plot this on a graph. (Or at least i don't know how to plot this on a graph).

This statement is wrong. Its the angle of deviation that changes with incidence angle not the angle of minimum deviation.

For a given prism, minimum deviation is constant, it cannot wary with incidence angle. Its a property of the prism just like refractive index.

In its proper form, angle of deviation $(\delta)$ is given by,

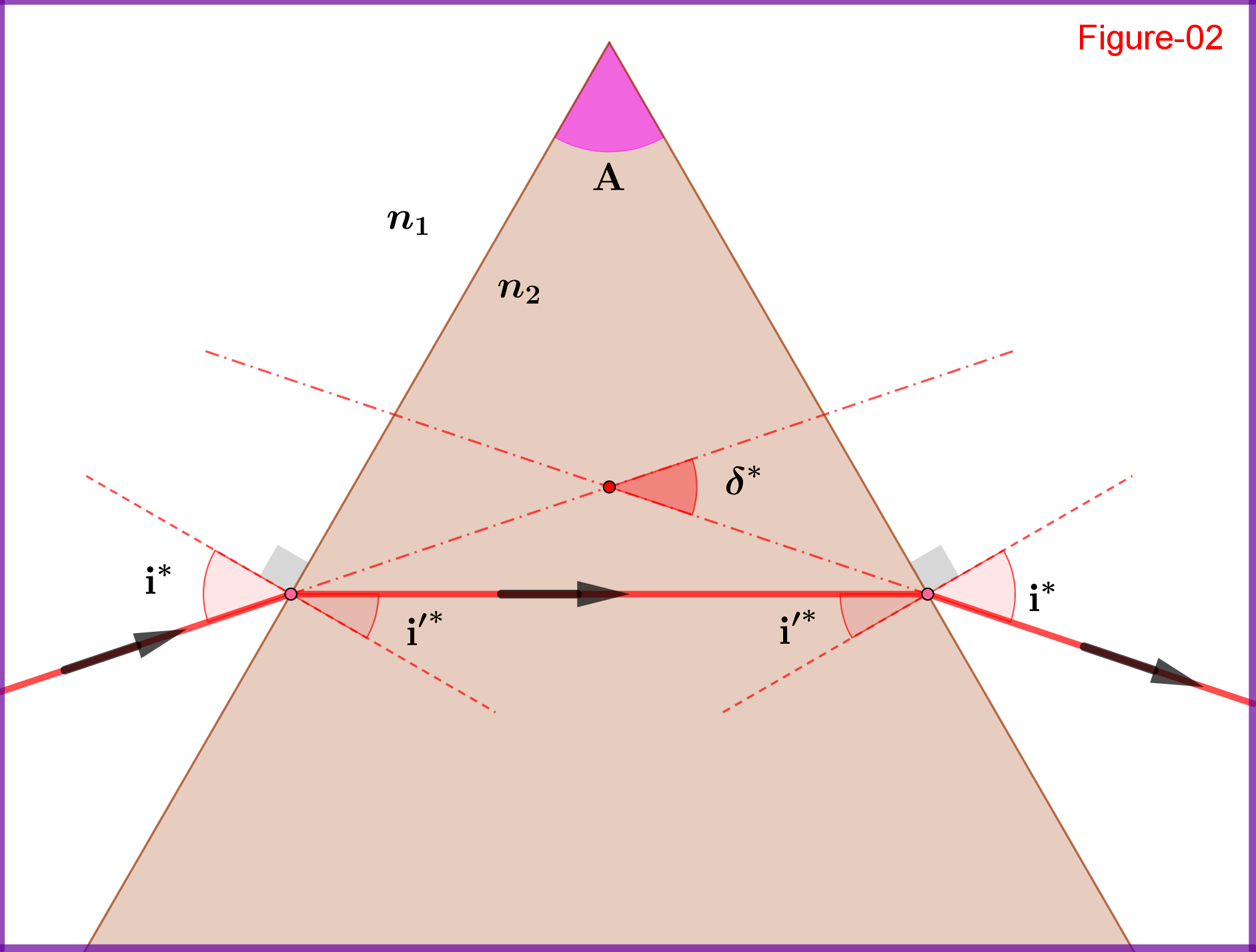
$\delta = (i+e)-A$ $\tag{1}$

$\delta _{min}=2i-A=2e-A$.

You can use equation $(1)$ to find out for which angle deviation is minimum when the angle of minimum deviation is given or vice versa.

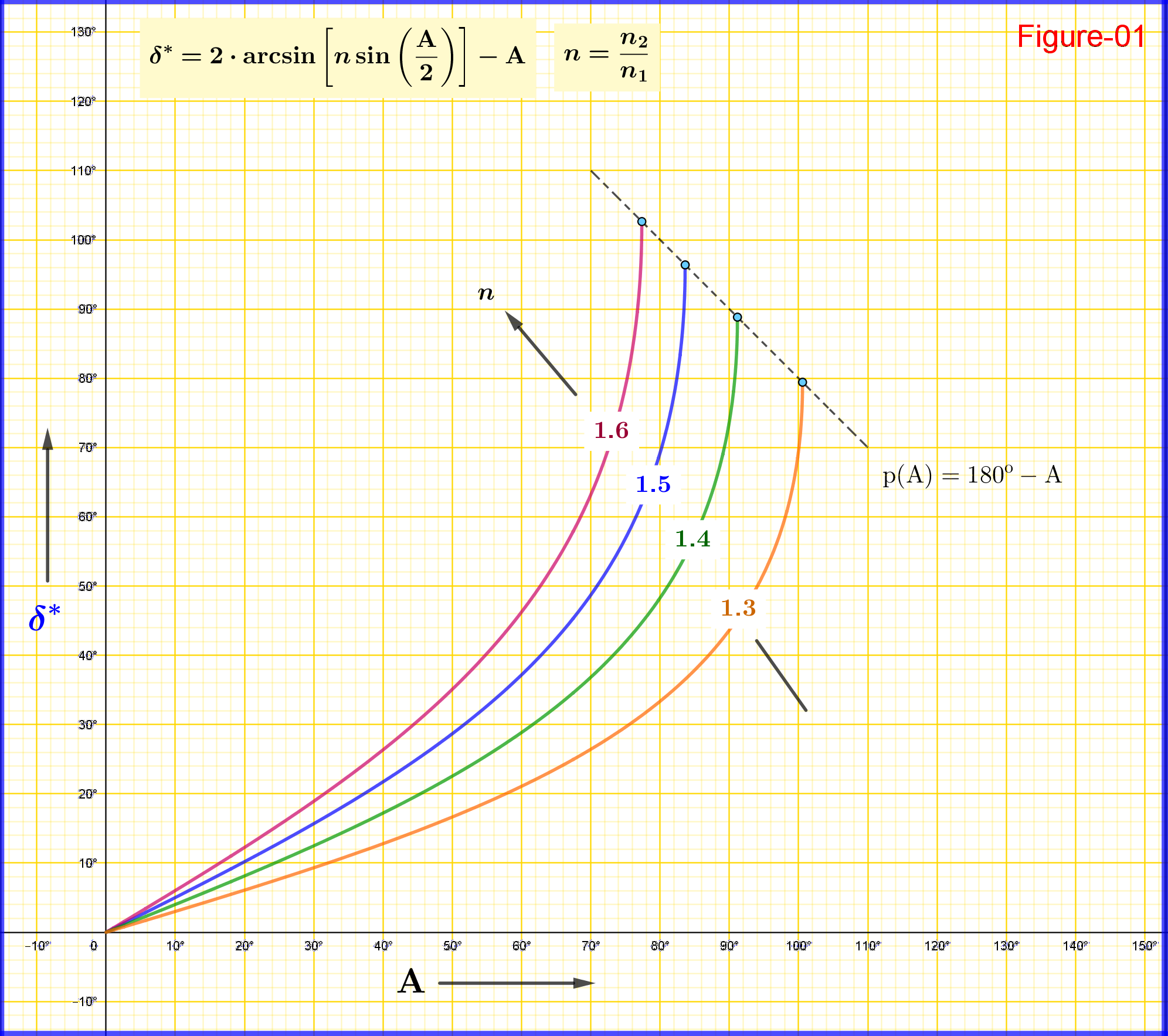
The second equation in your question, gives us the relation between the refractive index, the prism angle and the angle of minimum deviation. This equation applies to all the prisms in the universe!!

As you can see, for a constant angle of prism, angle of minimum deviation depends on the refractive index of the prism and for constant refractive index, angle of minimum deviation depends on the angle prism.

This means that prisms with different prism angles with same refractive index will give different angles of minimum deviation.

But if any one parameter is constant, all you have to do is to expand to sine formula to form up a relation between $\delta _{min}$ and the variable parameter.

Answer to the edit :

Angle of minimum deviation does not depend on the angle of incidence. Because you see, no matter what $i$ is ,for a given prism, $\delta _{min}$ will be the same. Its like saying, for $i=30^{\circ}$, $\delta _{min}$ is $d_1$ and for $i=60^{\circ}$, $\delta _{min}$ is $d_2$. This obviously is wrong. A prism can have only one minimum deviation angle.

If you want to plot a graph between $\delta _{min}$ and $A$, you will need the refractive index as explained above.

For $n=1.5$,

Input = 2 atan((sin(0.5x))/(1.5-cos(0.5x))

$A=2\tan^{-1}{[\frac{sin(0.5D)}{1.5-cos{0.5D}}]}$