Analytic solution for angle of minimum deviation? [closed]

Question

Consider a simple prism with a prism angle $A$, angle of incidence $\theta_1$, angle of emergence $\theta_4$ and the first and second angle of refraction as $\theta_2,\theta_3$. the refractive index for the prism (w.r.t the surroundings) is $n$. The angle of deviation is $\delta$.I wanted to derive an equation that could give the relation between $\theta_1$ and $\delta$, plot of which for a monochromatic light is as in the animation here. Below is my failed attempt (equations 2 and 3 are from the geometry of the figure):- $$\theta_4=\sin^{-1}n\sin(\theta_3)$$ $$A+\delta=\theta_1+\theta_4$$ $$A=\theta_2+\theta_3$$ $$\delta=\theta_1+\sin^{-1}n\sin(\theta_3)-A$$ $$\delta=\theta_1+\sin^{-1}n\sin(A-\theta_2)-A$$ $$\delta=\theta_1+\sin^{-1}n\sin(A-\sin^{-1}\frac{\sin(\theta_1)}{n})-A$$ Equation, when I plotted it on Wolframalpha for an equilateral prism with $n$=$1.5$ yielded the required plot in the limit $28.5^\circ<\theta_1<90^\circ$ (to avoid total internal reflection). But then, how do I use this equation, to analytically find the angle of minimum deviation, and the fact that at minimum deviation $\theta_1=\theta_4$. (I tried taking the derivative, but it turned out to be too complex).

Answer 1

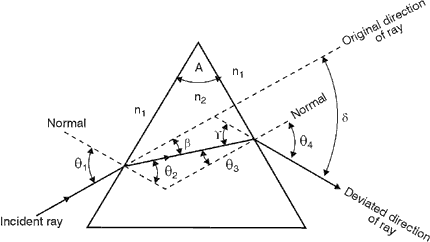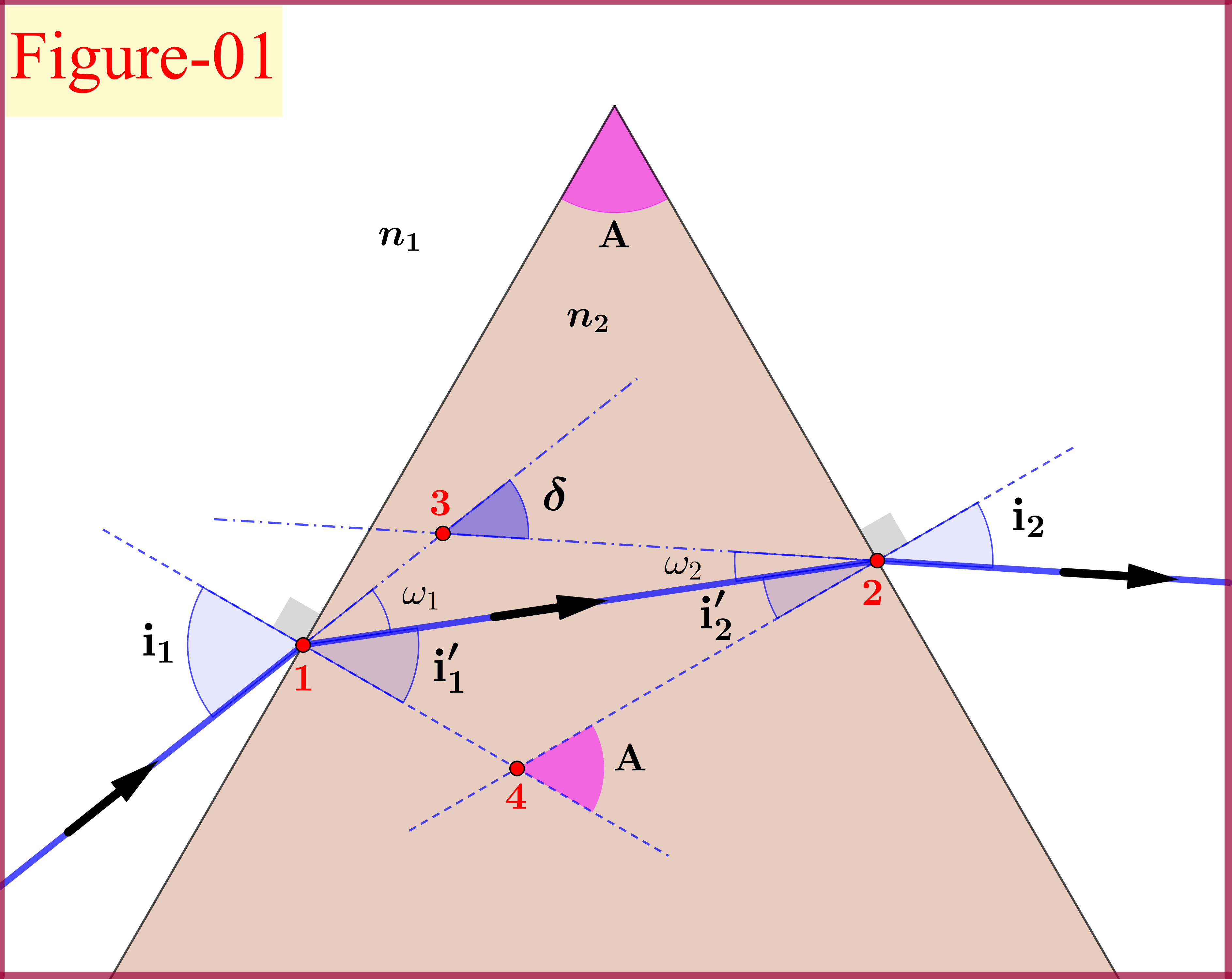
We'll express the emergent angle $\:\mathrm{i}_{2}\:$ and the deviation angle $\:\delta\:$ as functions of the incident angle $\:\mathrm{i}_{1}$ and after this we'll find the condition that minimizes the deviation angle $\:\delta$.

From Figure-01 we have \begin{align} \sin\mathrm{i}_{1} & = \dfrac{n_{2}}{n_{1}}\sin\mathrm{i}_{1}^{\prime}\qquad \text{Snell's Law at point }\color{red}{\boldsymbol{1}} \tag{01a}\\ \sin\mathrm{i}_{2}^{\prime} & = \dfrac{n_{1}}{n_{2}}\sin\mathrm{i}_{2}\qquad \text{Snell's Law at point }\color{red}{\boldsymbol{2}} \tag{01b}\\ \mathrm{i}_{2}^{\prime} & = \mathrm A - \mathrm{i}_{1}^{\prime}\qquad \quad \!\text{from triangle }\color{red}{\boldsymbol{124}} \tag{01c}\\ \delta & = \omega_{1}+\omega_{2}=\left(\mathrm{i}_{1}-\mathrm{i}_{1}^{\prime}\right)+\left(\mathrm{i}_{2}-\mathrm{i}_{2}^{\prime}\right)=\mathrm{i}_{1}+\mathrm{i}_{2}-\underbrace{\left(\mathrm{i}_{1}^{\prime}+\mathrm{i}_{2}^{\prime}\right)}_{\mathrm A} \nonumber\\ \Longrightarrow \quad \delta & = \mathrm{i}_{1}+\mathrm{i}_{2}-\mathrm A \qquad \!\text{from triangle }\color{red}{\boldsymbol{123}} \tag{01d} \end{align} so \begin{align} \mathrm{i}_{2} & \stackrel{(\rm 01b)}{=\!=\!=} \arcsin\left(\dfrac{n_{2}}{n_{1}}\sin\mathrm{i}_{2}^{\prime}\right)\stackrel{(\rm 01c)}{=\!=\!=} \arcsin\left[\dfrac{n_{2}}{n_{1}}\sin\left(\mathrm A - \mathrm{i}_{1}^{\prime}\right)\right] \nonumber\\ & \stackrel{(\rm 01a)}{=\!=\!=}\arcsin\left[\dfrac{n_{2}}{n_{1}}\sin\left(\mathrm A - \arcsin\left[ \dfrac{n_{1}}{n_{2}}\sin\mathrm{i}_{1}\right]\right)\right] \nonumber\\ & =\!=\!=\arcsin\left[\dfrac{n_{2}}{n_{1}}\left(\sin\mathrm A\sqrt{1- \left(\dfrac{n_{1}}{n_{2}}\right)^{2}\sin^{2}\mathrm{i}_{1}} -\cos\mathrm A \dfrac{n_{1}}{n_{2}}\sin\mathrm{i}_{1}\right)\right] \nonumber\\ & =\!=\!=\arcsin\left(\sin\mathrm A\cdot\sqrt{\left(\dfrac{n_{2}}{n_{1}}\right)^{2}- \sin^{2}\mathrm{i}_{1}} -\cos\mathrm A \sin\mathrm{i}_{1}\right) \nonumber \end{align} that is \begin{equation} \mathrm{i}_{2}\left(\mathrm{i}_{1}\right)=\arcsin\left(\sin\mathrm A\cdot\sqrt{\left(\dfrac{n_{2}}{n_{1}}\right)^{2}- \sin^{2}\mathrm{i}_{1}} -\cos\mathrm A \sin\mathrm{i}_{1}\right) \tag{02} \end{equation} and from (01d) \begin{equation} \delta\left(\mathrm{i}_{1}\right)=\mathrm{i}_{1} +\underbrace{\arcsin\left(\sin\mathrm A\cdot\sqrt{\left(\dfrac{n_{2}}{n_{1}}\right)^{2}- \sin^{2}\mathrm{i}_{1}} -\cos\mathrm A \sin\mathrm{i}_{1}\right)}_{\mathrm{i}_{2}}-\mathrm A \tag{03} \end{equation}

To find the minimum deviation angle we start from \begin{equation} \delta\left(\mathrm{i}_{1}\right)=\mathrm{i}_{1} +\underbrace{\arcsin\left[\dfrac{n_{2}}{n_{1}}\sin\mathrm{i}_{2}^{\prime}\right]}_{\mathrm{i}_{2}}-\mathrm A \tag{04} \end{equation} and so \begin{align} \dfrac{\mathrm d \delta \hphantom{_{1}}}{\mathrm d\mathrm{i}_{1}} = & \:1 +\dfrac{\dfrac{n_{2}}{n_{1}}\cos\mathrm{i}_{2}^{\prime}}{\sqrt{1-\left(\dfrac{n_{2}}{n_{1}}\right)^{2}\sin^{2}\mathrm{i}_{2}^{\prime}}}\dfrac{\mathrm d \mathrm{i}_{2}^{\prime}}{\mathrm d\mathrm{i}_{1}} \nonumber\\ \stackrel{(\rm 01a)}{=\!=\!=} & \:1 +\dfrac{\dfrac{n_{2}}{n_{1}}\cos \mathrm{i}_{2}^{\prime}}{\sqrt{1-\left(\dfrac{n_{2}}{n_{1}}\right)^{2}\sin^{2} \mathrm{i}_{2}^{\prime}}}\dfrac{\dfrac{n_{1}}{n_{2}}\cos\mathrm{i}_{1}}{\sqrt{1-\left(\dfrac{n_{1}}{n_{2}}\right)^{2}\sin^{2}\mathrm{i}_{1}}} \nonumber\\ =\!=\!=& \:1+\dfrac{\cos\mathrm{i}_{2}^{\prime}\cdot\cos\mathrm{i}_{1}}{\sqrt{1-\left(\dfrac{n_{2}}{n_{1}}\right)^{2}\sin^{2}\mathrm{i}_{2}^{\prime}}\:\sqrt{1-\left(\dfrac{n_{1}}{n_{2}}\right)^{2}\sin^{2}\mathrm{i}_{1}}} \nonumber \end{align} that is \begin{equation} \dfrac{\mathrm d \delta \hphantom{_{1}}}{\mathrm d\mathrm{i}_{1}}=1+\dfrac{\cos\mathrm{i}_{2}^{\prime}\cdot\cos\mathrm{i}_{1}\vphantom{\sqrt{1-\left(\dfrac{n_{2}}{n_{1}}\right)^{2}\sin^{2}\mathrm{i}_{2}^{\prime}}}}{\sqrt{1-\left(\dfrac{n_{2}}{n_{1}}\right)^{2}\sin^{2}\mathrm{i}_{2}^{\prime}}\:\sqrt{1-\left(\dfrac{n_{1}}{n_{2}}\right)^{2}\sin^{2}\mathrm{i}_{1}}} \tag{05} \end{equation} Now \begin{align} \dfrac{\mathrm d \delta \hphantom{_{1}}}{\mathrm d\mathrm{i}_{1}} & =0 \qquad \Longrightarrow \nonumber\\ \cos\mathrm{i}_{2}^{\prime}\cdot\cos\mathrm{i}_{1} & = -\sqrt{1-\left(\dfrac{n_{2}}{n_{1}}\right)^{2}\sin^{2}\mathrm{i}_{2}^{\prime}}\:\sqrt{1-\left(\dfrac{n_{1}}{n_{2}}\right)^{2}\sin^{2}\mathrm{i}_{1}}\qquad \Longrightarrow \nonumber\\ \cos^{2}\mathrm{i}_{2}^{\prime}\cdot\cos^{2}\mathrm{i}_{1} & = \left[1-\left(\dfrac{n_{2}}{n_{1}}\right)^{2}\sin^{2}\mathrm{i}_{2}^{\prime}\right]\:\left[1-\left(\dfrac{n_{1}}{n_{2}}\right)^{2}\sin^{2}\mathrm{i}_{1}\right]\qquad \Longrightarrow \nonumber\\ \left(1-\sin^{2}\mathrm{i}_{2}^{\prime}\right)\cdot\left(1-\sin^{2}\mathrm{i}_{1} \right)& = \left[1-\left(\dfrac{n_{2}}{n_{1}}\right)^{2}\sin^{2}\mathrm{i}_{2}^{\prime}\right]\:\left[1-\left(\dfrac{n_{1}}{n_{2}}\right)^{2}\sin^{2}\mathrm{i}_{1}\right]\qquad \Longrightarrow \nonumber\\ \sin^{2}\mathrm{i}_{1} & = \left(\dfrac{n_{2}}{n_{1}}\right)^{2}\sin^{2}\mathrm{i}_{2}^{\prime}\stackrel{(\rm 01b)}{=\!=\!=} \sin^{2}\mathrm{i}_{2} \tag{06} \end{align} so \begin{equation} \sin^{2}\mathrm{i}_{1}=\sin^{2}\mathrm{i}_{2} \tag{07} \end{equation} Since $\:\mathrm{i}_{1},\mathrm{i}_{2}\in \left[0,\pi/2\right]\:$ the condition for extreme deviation angle is \begin{equation} \mathrm{i}_{1}=\mathrm{i}^{*}=\mathrm{i}_{2} \tag{08} \end{equation} But then \begin{equation} \mathrm{i}_{1}^{\prime}=\mathrm{i}_{2}^{\prime} \tag{09} \end{equation} so from (01c) \begin{equation} \mathrm{i}_{1}^{\prime}=\dfrac{\mathrm A}{2}=\mathrm{i}_{2}^{\prime} \tag{10} \end{equation} From (01a) \begin{equation} \mathrm{i}^{*}=\arcsin\left[\left(\dfrac{n_{2}}{n_{1}}\right)\sin\left(\dfrac{\mathrm A}{2}\right)\right] \tag{11} \end{equation} Finally for the minimum deviation angle we have, see (01d), \begin{equation} \delta^{*}=2\cdot\mathrm{i}^{*}-\mathrm A =2\cdot\arcsin\left[\left(\dfrac{n_{2}}{n_{1}}\right)\sin\left(\dfrac{\mathrm A}{2}\right)\right]-\mathrm A \tag{12} \end{equation} From (12) the refraction index of the prism material relative to the surrounding material could be expressed as function of the prism angle $\:\mathrm A\:$ and the minimum deviation angle $\:\delta^{*}\:$ \begin{equation} \left(\dfrac{n_{2}}{n_{1}}\right)=\dfrac{\sin\left(\dfrac{\mathrm A + \delta^{*}}{2}\right)}{\sin\left(\dfrac{\mathrm A}{2}\right)} \tag{13} \end{equation}

---

Numerical Example

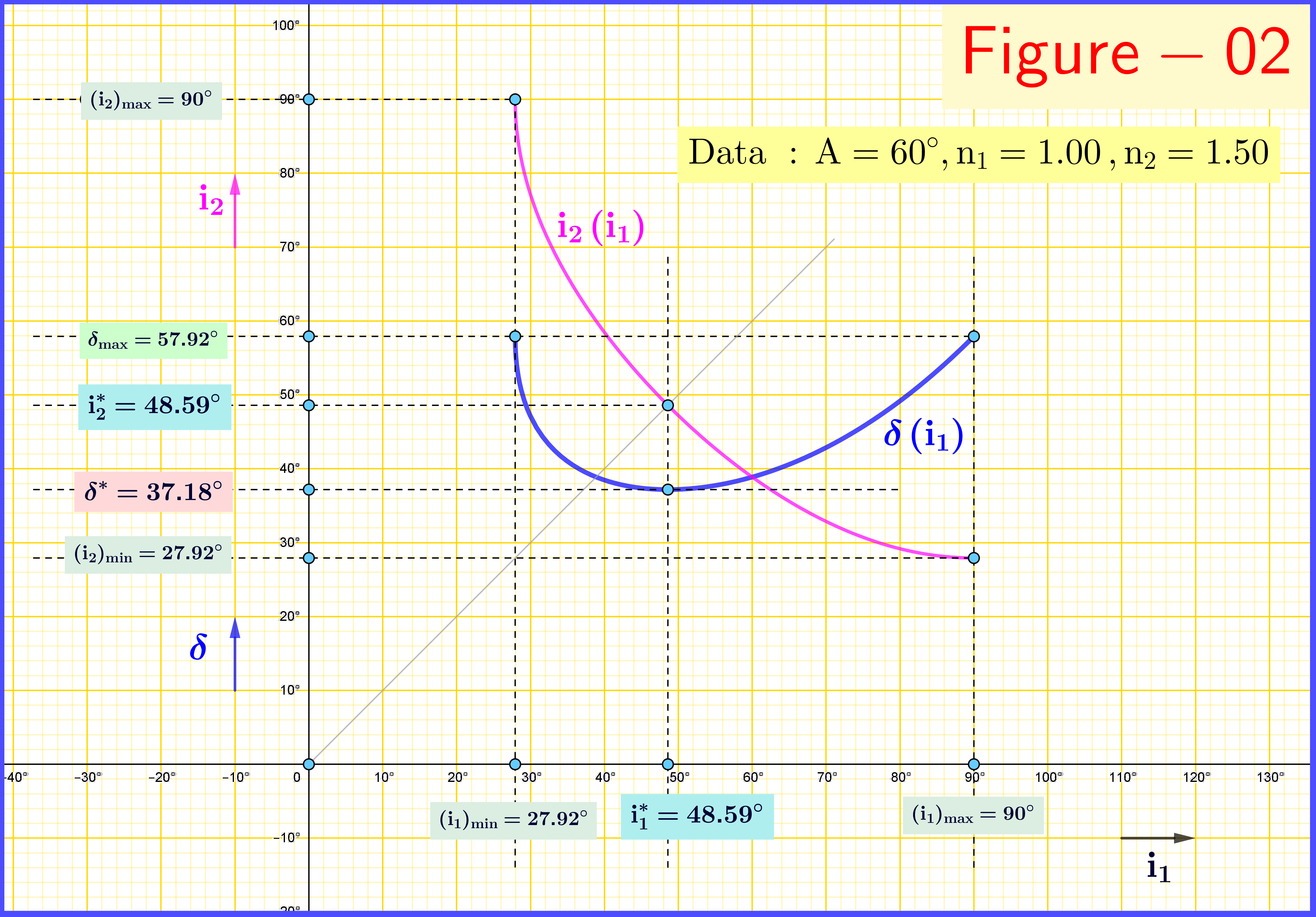
Let \begin{equation} \mathrm A =60^{\rm o}\,,\quad n_{1}=1.00\,,\quad n_{2}=1.50 \tag{14} \end{equation} From (11) \begin{equation} \mathrm{i}^{*}=\arcsin\left[\left(\dfrac{1.50}{1.00}\right)\sin\left(\dfrac{60^{\rm o}}{2}\right)\right]=\arcsin\left(0.75\right)=48.59^{\rm o} \tag{15} \end{equation} From (12) \begin{equation} \delta^{*}=2\cdot\mathrm{i}^{*}-\mathrm A =2\cdot48.59^{\rm o}-60^{\rm o}=37.18^{\rm o} \tag{16} \end{equation}

---

Related : Why does the graph of deviation angle in a prism doesn't get a symmetry?.

Answer 2

The answer is $$N = \frac{\sin \left((A+D)/2\right)}{\sin (A/2)}$$ at $\theta_1 = \theta$ for d = deviation, your $\delta$.

From symmetry, one can deduce that when angle 1 equals angle 4, the symmetrical case, then the deviation is either a maximum, or a minimum. The maximum possibility, is easily dismissed, so it must be a minimum.