Why must the single slit in a double slit experiment be narrow?

Question

I read that this causes contrast to become lost between dark and light fringes, but why?

Answer 1

The double slit experiment uses the fact that a single narrow slit diffracts light to create two coherent sources that will interfere. If the single slit wasn't narrow, there wouldn't be diffraction, and thus each of the two sources would only emit in a very narrow direction. In consequence, there wouldn't be any interference.

Answer 2

The single slit produces a diffraction pattern and if the single slit is narrow enough the central maximum of the diffraction pattern will illuminate both slots which then act as coherent sources.
The light from these coherent sources will overlap and produce an interference pattern.

With a light source like a laser the single slit is not necessary but the laser beam must be capable of illuminated both slits.

With a light source like a light bulb the single slit is necessary so that one can observe the interference pattern produced by the two slits.

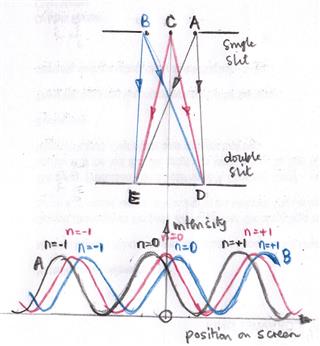
The diagram below shows a single slit $AB$ which illuminates two slits $D$ and $E$.

Light from the centre of the single slit $C$ travels equal distance to slits $D$ and $E$ and so the two slits act as sources which are in phase with one another and so produce an interference pattern , shown in red in the diagram, which is centre at position $0$ on the screen.

Light from edge $B$ of the slit travels different distances to slits $D$ and $E$ and so the two slits act as sources with the wave from each slit differing in phase with light from slit $E$ leading light from slit $D$.
This results in the interference pattern being displaced to the right of position $0$ on the screen which is shown in blue in the diagram.

There is a similar displacement pattern but in the opposite direction for the light coming from $A$ and is shown in grey in the diagram.

So from the whole of the single slit a series of displaced and overlapping interference patterns are produced.

This reduces the contrast (related to the difference between the maximum intensity and minimum intensity of the pattern) of the interference pattern and makes the interference fringes more difficult to observe.

If the difference in phase from different parts of the single slit can be kept below the equivalent of a fraction of a wavelength then the interference pattern should be visible.
This is achieved by having the central part of the central maximum of the diffraction pattern due to the single slit illuminating the two slits.

There are a number of related answers to your question which you may also care to read:
Answer 1
Answer 2
Answer 3

Answer 3

If you calculate the intensity $I$ at any point in space of a sum of two spherical waves: $$\Psi=\exp{(i\vec{k}\cdot\vec{x})}+\exp{(i\vec{k}\cdot(\vec{x}+\delta\vec{x}))}$$ coming from different points separated a distance $\delta \vec{x}$ noting that $I\propto |\Psi|^2$ you will obtain that $$I\propto\cos^2{\left(\frac{|\delta \vec{r}|\pi}{\lambda}\cos{\theta}\right)}$$ Where $\theta$ is the angle between the vectors $\delta{r}$ and $\vec{k}$.

For the interference pattern to appear clearly it is necessary that $|\vec{r}|\sim\lambda$ to avoid excessive oscillations in the pattern. This is the reason for which the sepparation must be of the order of the wavelength being therefore, small.