8
$\begingroup$

I have read that when bosons interfere they do so by adding the probability amplitudes, then I read that when fermions interfere they do so by subtracting the probability amplitudes.

The usual double slit interference pattern has a bright fringe in the middle and weaker fringes around it. If electrons (with same spin) are used and they interfere by subtracting the amplitudes, then we would get a negative interference pattern with a dark fringe in the middle and two fringes of equal strength around it don't we?

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ "I have read that when bosons interfere they do so by adding the probability amplitudes, then I read that when fermions interfere they do so by subtracting the probability amplitudes."...where have you read this? $\endgroup$
    – ACuriousMind ♦
    Commented Apr 12, 2016 at 10:30
  • $\begingroup$ @ACuriousMind Perhaps I misunderstood what I read in the Feynman Lectures vol3 /chapter 3. It says: "When a particle can reach a given state by two possible routes, the total amplitude for the process is the sum of the amplitudes for the two routes considered separately." Then later when they scatter two particles on each other: "In the case of α-particles with α-particles there are two alternatives that cannot be distinguished. Here, we must let the probability amplitudes interfere by addition". "In the case of electrons, the interfering amplitudes for exchange interfere with a negative sign" $\endgroup$
    – Calmarius
    Commented Apr 12, 2016 at 12:17
  • $\begingroup$ @ACuriousMind Probably the crux is that in the first case we have only 1 particles interfering with itself, while on later case we have at least 2... In that case I probably have the answer already that the two interference patterns will be identical... $\endgroup$
    – Calmarius
    Commented Apr 12, 2016 at 12:18
  • 2
    $\begingroup$ Yes, you misunderstood. The crucial word in "In the case of electrons, the interfering amplitudes for exchange interfere with a negative sign" is "exchange", it refers to the wavefunction of fermions being antisymmetric under exchange of the particles. It is not saying that in general you should substract fermionic amplitudes, just that, given some generic amplitude for two particles $\psi(x_1,x_2)$, you make it bosonic by $\psi(x_1,x_2) + \psi(x_2,x_1)$ and fermionic by $\psi(x_1,x_2)-\psi(x_2,x_1)$. this has nothing to do with substracting amplitudes in the double slit. $\endgroup$
    – ACuriousMind ♦
    Commented Apr 12, 2016 at 12:24

2 Answers 2

Reset to default
4
$\begingroup$

The double-slit experiment is a one-body experiment, meaning that one is only looking at interferences of one particle with itself. Thus the Bose or Fermi statistics does not play a role in that case.

What the OP has in mind in the Hong-Ou-Mandel effect, which for bosons implies that there is an increased probability that two identical bosons will be detected in the same detector, and an anti-correlation for identical fermions.

Improve this answer
$\endgroup$
0
$\begingroup$

There is an interesting detail about the intensity distribution behind edges from photons and electrons. The shadow from photons is smaller the "geometrical" shadow. The shadow behind an edge, built from electrons is always wider than geometrical shadow. This could be explained by the interaction between the electric field of the sharp edge (the surface electrons of this edge) and the particle beam.

In the case of photons the photon has an oscillating electric field and in dependence from the phase of the oscillation - (1) the positive pole or (2) the negative pole is nearer the edge, or (3) the magnetic field component is prevailing - the photon is deflected (1) towards the edge (2) away from the edge or (3) is undeflected. The first intensity maximum is exactly on the line of the geometrical shadow.

The deflection of electrons is different. Electrons have their electric field and get deflected away from the edge by the electric field of the surface electrons of this edge. The edge is wider the theoretical shadow. But the position and the width of the intensity distribution of electrons could be manipulated. Gottfried Möllensedt and Heinrich Düker have done such experiments (Beobachtungen und Messungen an Biprisma-lnterferenzen mit Elektronenwellen. In: Zeitschrift für Physik. Nr. 145, 1956, S. 377-397.) I'm sorry. It's in German. Here are two pictures from the original work:

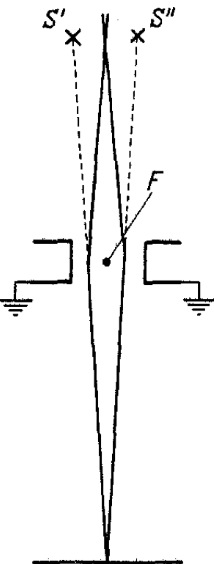
enter image description here

The biprisma.

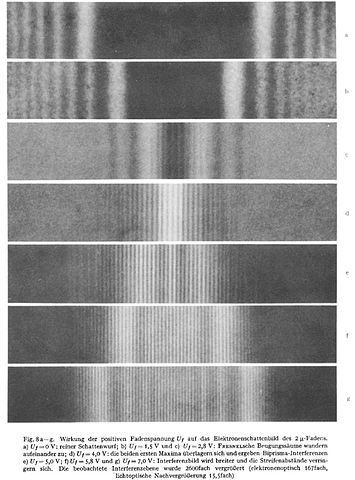
enter image description here

Different intensity distributions in dependence from the electric potential difference between the wire and the ground.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.