1
$\begingroup$

I'm studying the mechanics of a rigid solid of revolution on an incline. This is somewhat a variation of the double-cone "paradox", just slightly more general (hence, complicated).

Geometry

Here is the setup:

  • An incline, made of two rails, with arbitrary profile $(x,\gamma_2(x),\gamma_1(x))$ (see the images below, top and side views);
  • A solid of revolution, with base-profile $\gamma_3(t)$ (see the image below)

As for the double-cone paradox, there are some triplets of $\gamma$'s which will make the solid apparently roll up along the rails (whilst its center-of- mass falls). More precisely, the center-of-mass trajectory is $$\gamma_{cm}(x)=\gamma_1(x)+\gamma_3(\gamma_2(x))\sqrt\frac{1}{1+\{\frac{d\gamma_1(x)}{dx}\}^2}$$

The given solution holds until (and if) the solid reach the top of the rails. Then it becomes the opposite one. Hopefully, if frictions are just enough, the solid will continue to ramp up and down the top, like an upside-down pendulum.

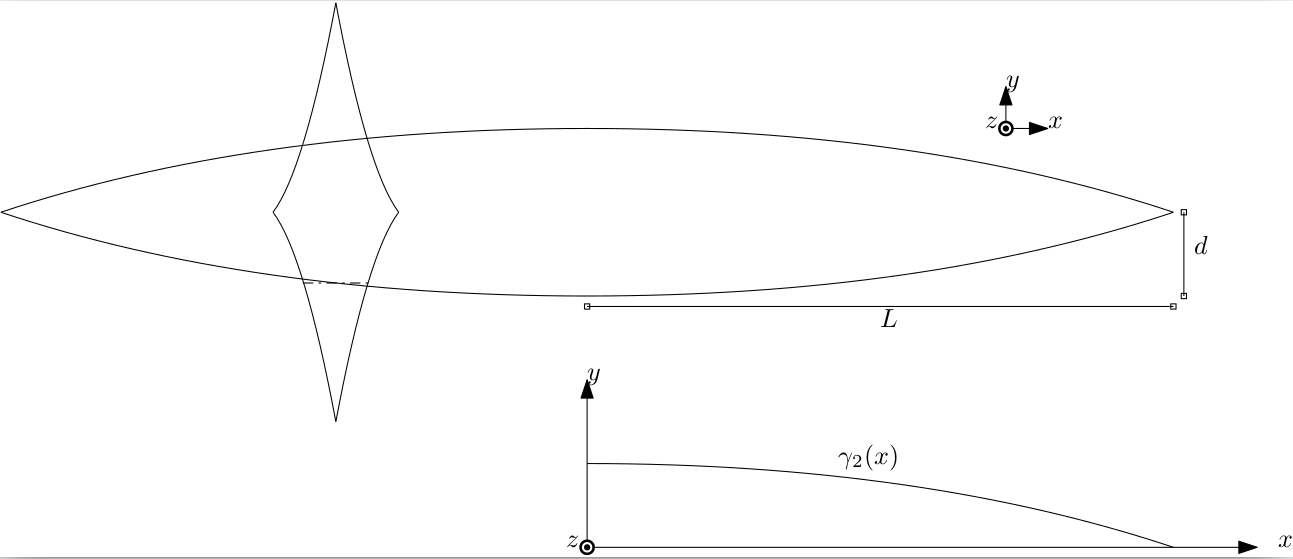
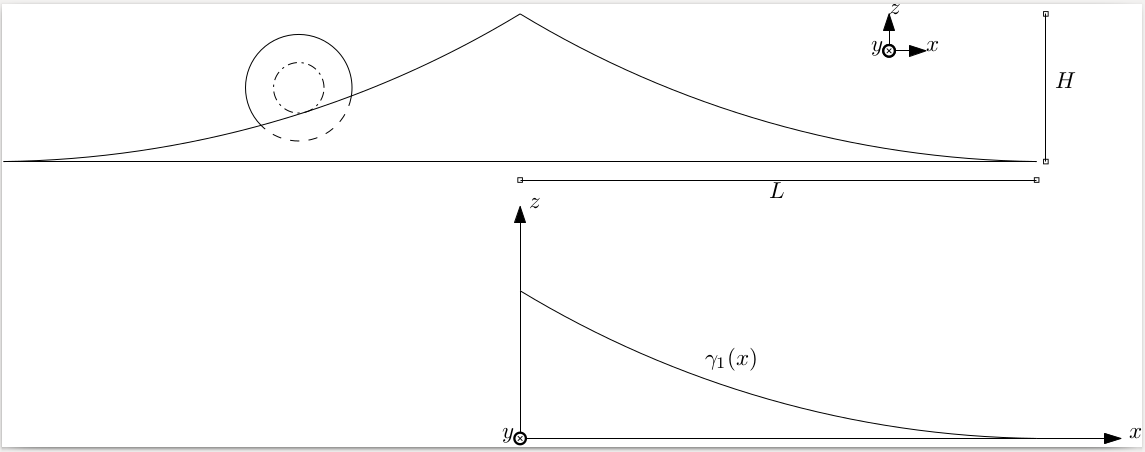
Top view Front view Cone

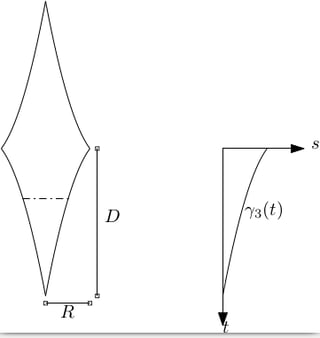
Mechanics

For demonstrative purposes I'd like to compute the center-of-mass acceleration, assuming no friction and rolling without slipping. This could be done equivalently using the generalized coordinate $l$ along $\gamma_1$, or $s$ along the center-of-mass trajectory.

In what follows I'm using the observations made here, for a similar problem:

Deflating wheel rolling without slipping

The system lagrangian should be, using $s$

$$L=T-V=\frac{1}{2}M\dot{s}^2+\frac{1}{2}I_{cm}\dot{\theta}^2-V(s)$$ with $V(s)=Mgh(s)$ the gravitational potential associated to an height $h(s)$ and $I_{cm}$ the moment of inertia around the symmetry axis.

Equivalently, using $l$ (and the Huygens-Steiner theorem) $$L=\frac{1}{2}M\dot{l}^2+\frac{1}{2}I_{l}\dot{\theta}^2-V(l)\\ =\frac{1}{2}M\dot{l}^2+\frac{1}{2}I_{cm}\dot{\theta}^2+\frac{1}{2}M[r(l)]^2\dot{\theta}^2-V(l)$$

Questions

  • First of all, am I right with the previous considerations?
  • Secondly, the rolling without slipping condition is easy to write using $l$ $$r\dot{\theta}=\dot{l}$$ (see the analysis in the other question). Than the angular variable can be removed, as for the simple cylinder rolling on an incline. Now, how to derive the acceleration of either the center-of-mass or the contact point?
Improve this question
$\endgroup$
5
  • $\begingroup$ Any comment would be helpful,including alternative ways to solve (i.e. force detailed analysis, hamiltonian formalism, etc.) $\endgroup$
    – Riccardo Buscicchio
    Commented May 3, 2017 at 17:23
  • $\begingroup$ I believe your center of mass trajectory is incorrect. I believe that gives you the height of the center of mass as a function of the $x$-position of the point of contact, which is not necessarily directly below the center of mass, so that height may not be over the given $x$-value. $\endgroup$
    – JoDraX
    Commented May 5, 2017 at 22:05
  • $\begingroup$ Yes. You're right. But I don't think It changes the solvability of the system. In both lagrangian $x$ disappears,in favour of_generalized_ $l$ and $s$. $\endgroup$
    – Riccardo Buscicchio
    Commented May 5, 2017 at 22:16
  • $\begingroup$ This looks really hard to solve in full generality. As a first step, have you tried to consider small oscillations around the stable point? The equation of motion should then be $\ddot{s} = -\omega^2 s$ with $\omega$ depending on a few parameters ($\gamma_{1,2}(0)$, $\ddot{\gamma}_{1,2}(0)$, $\gamma_3(\gamma_2(0))$ and maybe $\dot{\gamma}_3(\gamma_2(0))$) instead of three functions. Assume that $s$ is small and drop everything that is of order $s^3$ and higher in the Lagrangian. Then compute the equation of motion. $\endgroup$
    – Steven Mathey
    Commented May 8, 2017 at 10:19
  • $\begingroup$ Note that you will have to consider a smooth function for $\gamma_1(t)$ instead of the kink that you draw. Then you can then consider the limit $\ddot{\gamma}_1(0)\rightarrow -\infty$ to restore the kink. I would be interested in seeing how $\omega$ behaves in this limit. $\endgroup$
    – Steven Mathey
    Commented May 8, 2017 at 10:21

1 Answer 1

Reset to default
1
$\begingroup$

I think you missed a derivative in your lagrangian, when you split the linear velocity in its x and z component:

$\vec{v} = \dot{x} \vec{e}_x + \dot{z} \vec{e}_z = (\vec{e}_x + \underbrace{\dot{ \gamma}_1 + \dot{ \gamma}_3( \gamma _2(x)) \; \dot{ \gamma }_2 ( x)}_{=\alpha(x)} \vec{e}_z) \dot{x} $

The corresponding lagrangian would be:

$$ L = E - V = \frac{1}{2} \left(\frac{\bf{I}}{(\gamma_3(\gamma_2(x)))^2} + m \right) (1+\alpha^2) \dot{x}^2 - mg \left(\gamma_1 + \gamma_3( \gamma _2(x))\right) $$

You can derive the equations of motion for x from this via the Euler-Lagrange equation but I strongly doubt that it would be solvable without further assumptions.

Improve this answer
$\endgroup$
1
  • $\begingroup$ What is the point with such $z$ coordinate? Either you're missing $\sqrt{\frac{1}{1+(\frac{d\gamma_1}{dx})^2}}$ in $z_{cm}$, or you are adding incorrectly the time derivative of $\gamma_3(\gamma_2(x)$ if you're working with the contact point. In addition, for the second case, the tensor of inertia $I$ would have to be computed with respect to the contact point, $$I=I_ {cm}+\frac{1}{2}Mr(x)^2$$ thus adding a component depending on $r(x)$ (that is why I mentioned the Huygens-Steiner theorem). $\endgroup$
    – Riccardo Buscicchio
    Commented May 8, 2017 at 9:55

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.