Component of centripetal force in direction of time-average resultant [closed]

Question

I am not sure exactly how to derive the expression required in the final part (f).

I proceeded as follows:

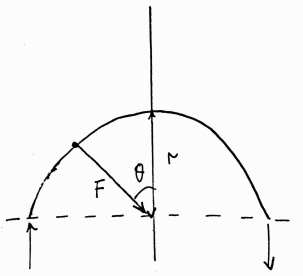
Given that the time-average of the resultant force over the turn is opposite to the initial direction of the vehicle (before the turn), we are seeking the vertical component of the centripetal force, $F_y$, at any point in terms of $t$. Now,

$\displaystyle F_y=F\cos\theta=\frac{v^2}{r}\cos\theta$.

All that is required is $\theta$ in terms of $t$. I presume that I must incoporate the angular displacement $\omega t$ somehow. I derived the equation $\theta = \pi/2 -\omega t$, from the assumption that $\omega t$ is zero at the beginning of the turn. Hence

$\displaystyle F_y=\frac{v^2}{r}\cos\left(\frac{\pi}{2}-\omega t\right)$.

Is this solution valid?

Answer 1

No.

Firstly:

$ F = ma, \frac{v^2}{r} = a,$ and therefore $centripetal force = ma = \frac{m(v^2)}{r} $

Secondly, the cosine term should just be $ sin(\omega t) $, ditch the phase shifting. The question specifies the angle relative to the Horizontal so just define it relative to the horizontal but take the angle such that at $t=0$, $\theta = 0.$ Otherwise, taking your definition of the angle and your formula [corrected to add in the m] you'd end up with $\frac{m(v^2)}{r}sin(\theta ')$ going from $\frac{-\pi}{2}$ to $\frac{\pi}{2}$ which would be wrong. [For instance, at the part where the function should reach it's maximum at the middle of the track, it's instead zero.]

The term should be:

$Fy = \frac{m(v^2)}{r}sin(\omega t)$