Is my understanding of the mechanics of skidding correct?

Question

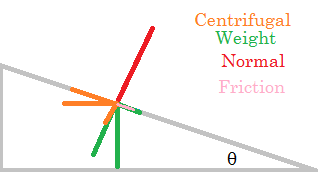
I'm trying to understand the mechanics which determine if a car making a turn will skid.

Are the following correct or incorrect:

1. A vehicle making a turn will skid unless the centripetal force is adequate to produce the centripetal acceleration. In a simple turn, where $r$ is constant, the force needed is $mr\omega^2$.

2. If the road is flat, this force can only come from friction created by turning the wheel. The maximum force is $\text{weight}\times\mu_\text{static}$. All of this force will be directed centripetally, and will therefore be available to prevent the skid.

3. If the road is banked by $\phi$, the friction force will be lessened by $\cos\phi$, but there will also be a centripetal component of the normal force, equal to $\sin\phi\cos\phi$.

Are these all correct? I believe they are but my results using them don't seem to work.

UPDATE: I believe my mistake was in the direction friction will be in. Determining the direction of friction in this case is tricky, because it has to both counteract the sliding due to normal force of the banked road, and also accelerate in the centripetal direction. It requires 3 dimensions. How do I determine the direction of friction, with respect to $r$ and $\theta$ vectors?

Answer 1

(1) is correct, but (2) and (3) are not. Consider a car turning counterclockwise around a track that is banked inwards. There are three forces acting on the vehicle: the weight of the car $\vec{W}$, the normal force $\vec{N}$, and the force of friction $\vec{f}$. Breaking these up into Cartesian coordinates gives:

$$\vec{W} = -mg\hat{y} \\ \vec{N} = N \left(\cos\phi \hat{y} - \sin\phi \hat{x}\right) \\ \vec{f} = f \left(-\sin\phi \hat{y} - \cos\phi \hat{x}\right)$$

Getting the direction correct for the friction is a bit subtle. We know from the $\phi \rightarrow 0$ limit that the friction has to point roughly in the direction of the turn, and also be perpendicular to the normal force, so for these coordinates, it must point down and to the left.

Since the car is moving in a circle in the horizontal plane, the forces in the $\hat{y}$ direction must cancel. Setting these equal to each other and considering the limiting case $f = \mu_s N$ gives an equation that can be solved for $N$. Then you can sum up the horizontal forces an insert this value to get the centripitel force.

Answer 2

As a side note skidding is not a yes or no state with tires. See this answer for more details.

The sum of the normal force and friction force that act on the car is the reaction force to the sum of the weight and centrifugal force of the car on the road.

We can equate them in the coordinate frame parallel and perpendicular to the road.

$$N=m\,g\, \cos(\theta) + m\,r\,\omega^2\,\sin(\theta)$$

In order for the car to not skid the maximum available frictional force must exceed the virtual centripetal force in the direction parallel to the road.

$$\mu_s\, N \gt |m\,r\,\omega^2\,\cos(\theta)-m\,g\, \sin(\theta)|$$

Combining yields:

$$\mu_s (m\,g\, \cos(\theta) + m\,r\,\omega^2\,\sin(\theta)) \gt |m\,r\,\omega^2\,\cos(\theta)-m\,g\, \sin(\theta)|$$

$$\mu_s \gt \frac{ |r\,\omega^2\,\cos(\theta)-g\, \sin(\theta)|}{g\, \cos(\theta) + r\,\omega^2\,\sin(\theta)}$$