Translation of Vectors

Question

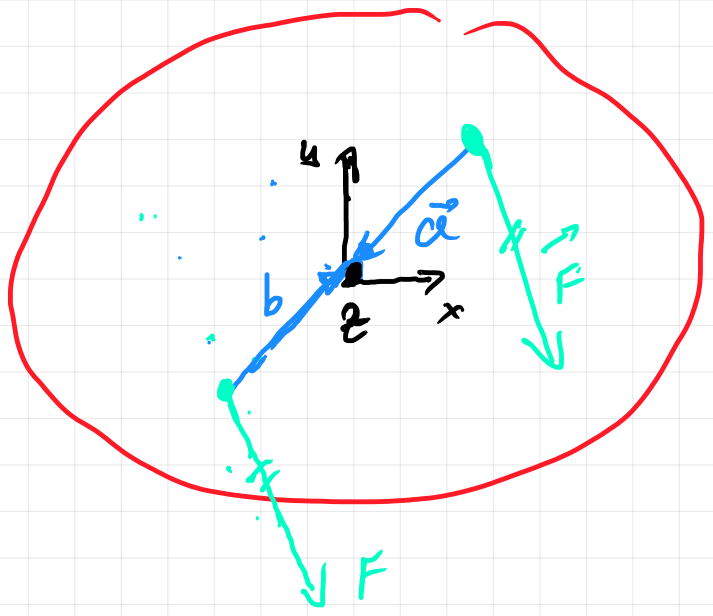
I am a bit confused about translation of vectors. In the first class in physics itself we are told that we can translate vectors as we like to the desired position to do whatever that we are trying to do. For example, if someone draws two random vectors then to get the sum, we translate them, make a parallelogram and draw the diagonal as the resultant.

However I have some doubts on this. In the following example, clearly we cannot translate the vectors. Consider this rigid body. We want to calculate the torque about origin of a force. Now if we translate the force vector, then we would obtain the following. 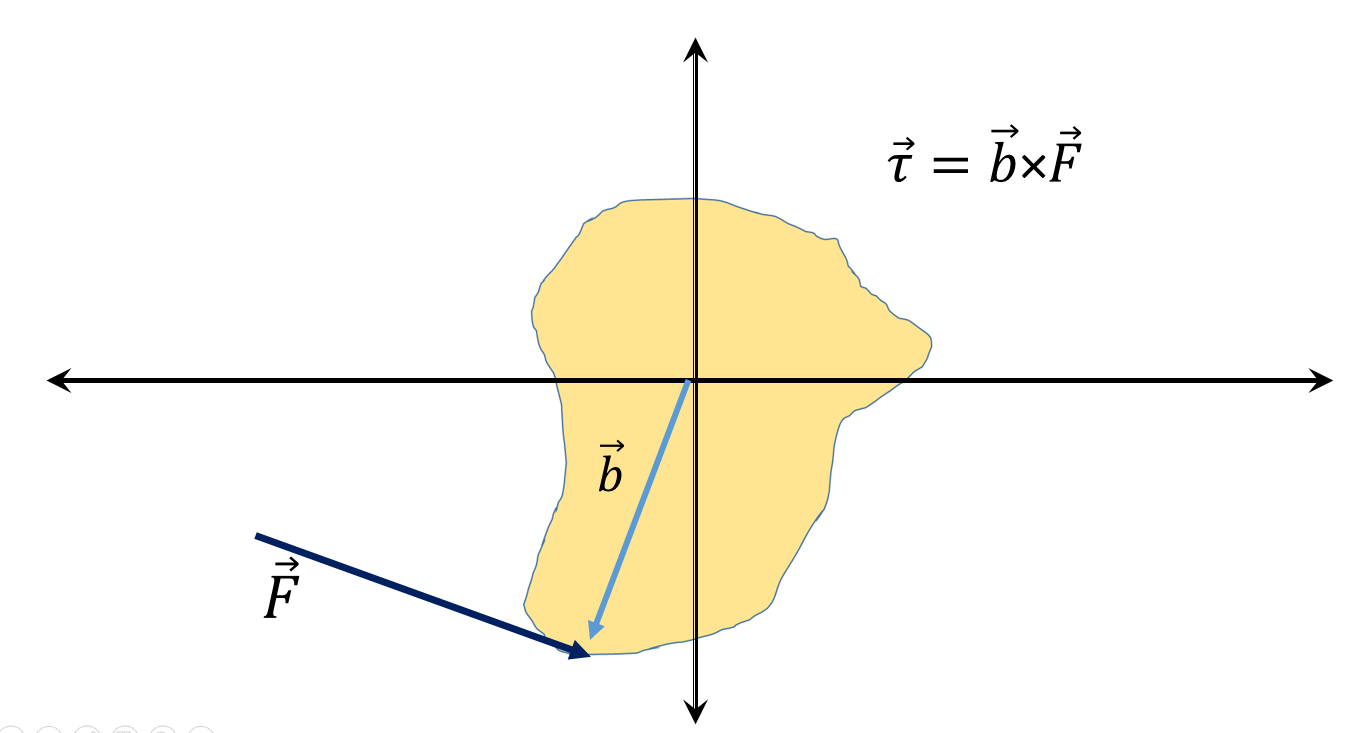Obviously the situation are very different and its not equivalent.
So are we really allowed to translate vectors?

Answer 1

Yes, we are allowed to translate vectors. To put it simply, think of coordinate geometry. When you shift the origin, it doesn't effect the orientation and length of a line segment. Similarly, in vectors, translation doesn't effect the vector as a vector is defined by its magnitude and direction. You can move it to anywhere in space and it will remain the same, provided these 2 properties don't change.

In the example you gave in the problem, although force vector doesn't change and is translated, the position vector of the point of application changes . b and a are completely different vectors in terms of magnitude and direction and hence their respective vector products with force vector are different and hence, the torque is different.

To summarize, translation of vectors means that the vector doesn't change if you move it to different points in space. However, the torque on a body also depends upon the position vector from centre of mass, which changes in this case. This doesn't contradict translation of vectors, since vector product depends upon both the vectors, not only force.

Update-Point of application matters only because position vector depends upon it and hence torque.

Answer 2

Vectors that cannot be translated are the ones that depend on the origin of coordinates. First and foremost in the list is the position vector $\vec r$ followed all the others with definitions that depend on the position vector. These would be torque, $\vec \tau = \vec r \times \vec F$; angular momentum $\vec L = \vec r \times \vec p$ and so on. That is why when you mention torque or angular momentum you have to specify the reference point about which you express these vectors in order to make sense.

Displacement, being the difference between two position vectors, can be translated. The vector from Boston to Philadelphia is the same regardless of whether you specify the positions of the two cities relative to New York or London. This means that vectors derived from the displacement vector, e.g. linear velocity, can also be translated.

A notable exception is the linear velocity in rotational motion, $\vec v = \vec \omega \times \vec r$ because, of course, the position vector of the rotating object figures in it. If you translate this velocity vector around the circle from the 12 o'clock to the 3 o'clock position you will have to change its direction by $90^{\text{o}}.$

Answer 3

What you are describing is a property of vectors. Vectors are not defined by their location in space. They are only defined by their magnitude and direction.

Intuitively speaking, a vector describes displacement from its start point to its end point. The displacement between these two points is only defined by how much space there is between them, and the direction the start point "faces" across this space. It doesn't matter where in a plane or space the vector is because the displacement it describes is the same. If I have a three-dimensional force vector with a magnitude of 10 N facing north (I use "north" very loosely), regardless of whether this vector is positioned here on Earth or on the Sun it is identical in quality.

As you say, when working with vectors algebraically their position is of no importance because the displacement they describe is not affected by their position. However, this is simply not true when describing forces in the real world. A force, as a vector, is still defined by just its magnitude and direction. The way a force interacts with the real world does not change its properties. Nevertheless, where we direct that force affects the translational, rotational, and vibrational motion of the object. The position vector is more of a mathematical tool than anything else. The way moment is defined, taking the cross product of the position vector and the force vector will give us the moment about the start point of the position vector. The position vector, similarly, is still defined only by its magnitude and direction, regardless of its application in real life.

Answer 4

The definition of a vector as having magnitude and direction is typically used in physics, so the exact location of the vector is not included in the definition. This is also called a "free" vector. As you say the effect of the vector depends on its location. For example, a force (vector) cannot act upon a point mass unless is acts "at" the location of the mass.

Physicists typically regard a vector as a free vector, recognizing the effect of the vector depends on its location. [Davis, Introduction to Vector Analysis] [Symon, Mechanics]

Engineering texts (and some older physics texts) sometimes distinguish among free, bound, and sliding vectors.

A good discussion of a free vector and its use in transforming between coordinate systems (for example, from an inertial system to a rotating and translating system) can be found in the textbook Mechanics by Symon.

Answer 5

In your given exercise, let force $\vec F$ be $\begin{bmatrix} 7 \\ -2 \\0\end{bmatrix},$ its application point $A$ be $(2,2,0),$ and $B$ be the point $(7,-2,0).$

1. As $\vec{OA}$ and $\vec{OB}$ are position vectors, they are “bound vectors”.
2. Now, the torque $\vec\tau$ about point $O$ due to force $\vec F$ applied at point $A$ is a function of (depends on) the distance between $O$ and $\vec F.$ So, computing $\vec\tau$ intrinsically forbids $\vec F$ from freely translating (this will change said distance from $\left|\vec{OA}\right|\sin\theta$ to something else) but allows $\vec F$ to freely slide along its line of action (this does not affect said distance); in other words, for the purpose of computing $\vec\tau,\;\vec F$ is a “sliding vector”.
3. Finally, let's geometrically determine the sum of $\vec F$ and $\vec{OA}.$ We do this by applying the Parallelogram Law of Vector Addition, whose algorithm demands that the two vectors be freely translated (but not rotated, for that would alter them). Here, clearly $\vec F$ and $\vec{OA}$ are “free vectors”.

Notice that even within a single physical setup, the vector $$\vec F=\begin{bmatrix} 7 \\ -2 \\0\end{bmatrix}=\vec{OB}$$ is variously “free”, “sliding” and “bound”, depending on the context; by “context”, I mean what we are doing with the vector, in other words, what definition or theorem we are invoking for the task at hand.

The moral is this: after the physical scenario has been mathematically modelled (abstracted), the classification system <free vector versus sliding vector versus bound vector> is unnecessary; instead, carefully respecting the phrasing and conditions of definitions and theorems ensures that steps performed are valid.

Answer 6

Yes, you can translate vectors, because they are defined by magnitude and direction, they are not defined by their location.

In the OP's picture, torque is a vector, and defined by the cross product of two others. If one of them changes and the other is the same, torque changes.

It is not so different from the concept of a scalar. Suppose a sphere where half of its volume has a mass $m$, and the other half mass $M$, ($M > m$). If the volume with M forms an inner sphere and m is a shell covering it, we have a symmetric object with an indiferent equilibrium. But if one hemisphere is M and the other m, it has different properties, like a preferred stable position. Depending on the relative position of the scalar quantity mass, the physical situation changes, but the masses themselves don't change.

Answer 7

to obtain the torque about the z-axis the tail of the vector a and the tail of vector F must be at the same point. the tip of the vector a and b point to $~(0,0,0)~$

from the fig the components of the vectors in $~(x,y,z)~$ coordinate system are

$$\vec F=\begin{bmatrix} F_x \\ -F_y \\ 0 \\ \end{bmatrix}\\ \vec a=\begin{bmatrix} -a_x \\ -a_y \\ 0 \\ \end{bmatrix}\\ \vec b=\begin{bmatrix} b_x \\ b_y \\ 0 \\ \end{bmatrix}$$

thus the torques

\begin{align*} &\vec{\tau}_a=\vec a\times \vec F=\begin{bmatrix} 0 \\ 0 \\ a_x\,F_y+a_y\,F_x \\ \end{bmatrix}\\ &\vec{\tau}_b=\vec b\times \vec F=\begin{bmatrix} 0 \\ 0 \\ -b_x\,F_y-b_y\,F_x \\ \end{bmatrix} \end{align*}

so $~\vec \tau_b=\vec\tau_a~$ if $~\vec b=-\vec a~$