What is the branching fraction of the $\Delta^0$?

Question

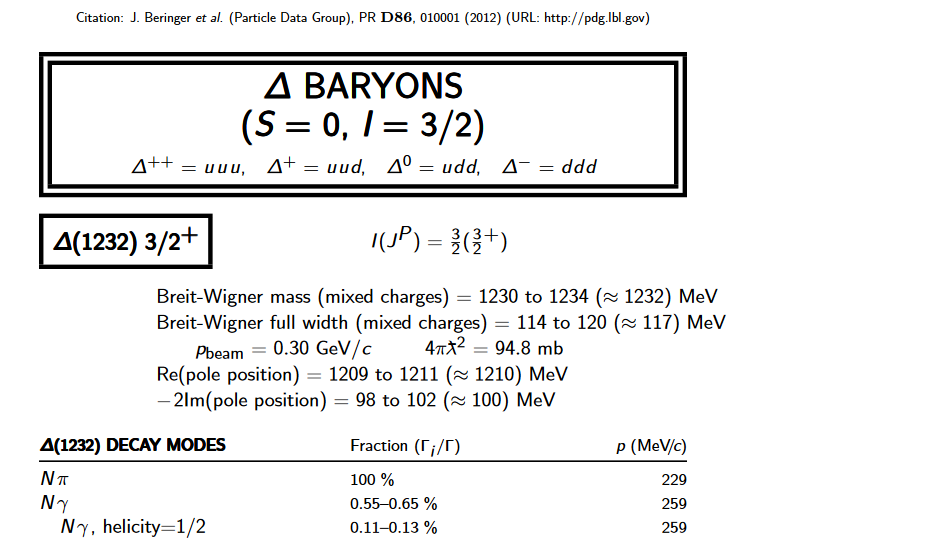
I've been looking for a literature value and can't find one, even from the PDG. Does anyone know where I could find the branching fractions for the $\Delta^0$ decay modes, specifically $\Delta^0 \rightarrow n + \pi^0$ $\Delta^0 \rightarrow p + \pi^-$?

Answer 1

Except for small electromagnetic decay branching ratios like $\Delta\to N\gamma$, essentially 100% of the branching ratio of the $\Delta$ is to $N\pi$ final states. This is just kinematics. The only other hadronic final state with baryon number one that is energetically allowed is $N\pi\pi$, which has an extremely small phase (it is just barely allowed).

The relative rates for $\Delta^0\to n+\pi^0$ and $\Delta^0\to p+\pi^-$ are determined by isospin. The relevant Clebsch-Gordon coefficients are $$ \langle 3/2,-1/2|1/2,-1/2;1,0\rangle = \sqrt{2/3}\\ \langle 3/2,-1/2|1/2,1/2;1,-1\rangle = \sqrt{1/3} $$ so the relative rates are 2/3 and 1/3.

Answer 2

The resonance has an isospin and as a strong interaction resonance, the decay channels are treated in the PDG as one "Nucleon pi" decay

The correct ratio is given in the answer by @Thomas, who gives the algebra of the SU(2) isospin case. 2:1