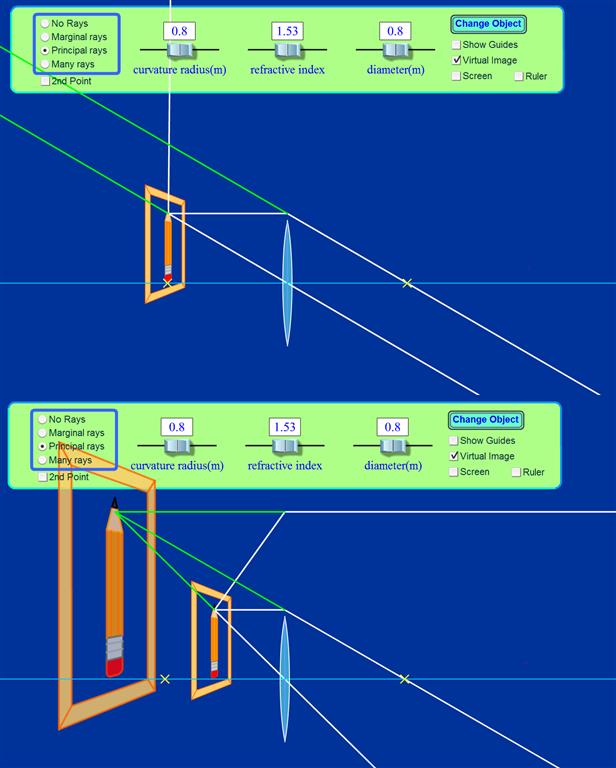
If an object is placed between focal length and optical center of a convex lens, a virtual, magnified and erect image is formed. But if we change the position of object within the focal length of lens, does the image still remains at 25 cm from eye? In other words is image always formed at least distance of distinct vision regardless of the position of object as long as it is within the focal length?
-
1$\begingroup$ I've seen several discussions in textbooks that lead to this confusion. There is nothing magical happening that forces the image to be at the near point, but you get the largest possible view (i.e. the best angular resolution) when that is the case. So part of the optimal use of a magnifying lens is to position the glass so that the image forms at your near point with your eye very near the lens and the book is assuming that you will have done this. $\endgroup$– dmckee --- ex-moderator kittenCommented Jan 23, 2017 at 9:47
3 Answers
If the object is in the focal plane of the lens then the upright, virtual image is seen at infinity - parallel rays of light enter the eye.
Bringing the object closer to the lens also brings the virtual image closer and the angular magnification also increases.
With the unaided eye the3 greatest magnification is obtained when the virtual image is as close as it can be whilst the optical surfaces within the eye still produce a focussed image on the retina.
That distance is called the least distance of distinct vision and usually is taken to be 25 cm.
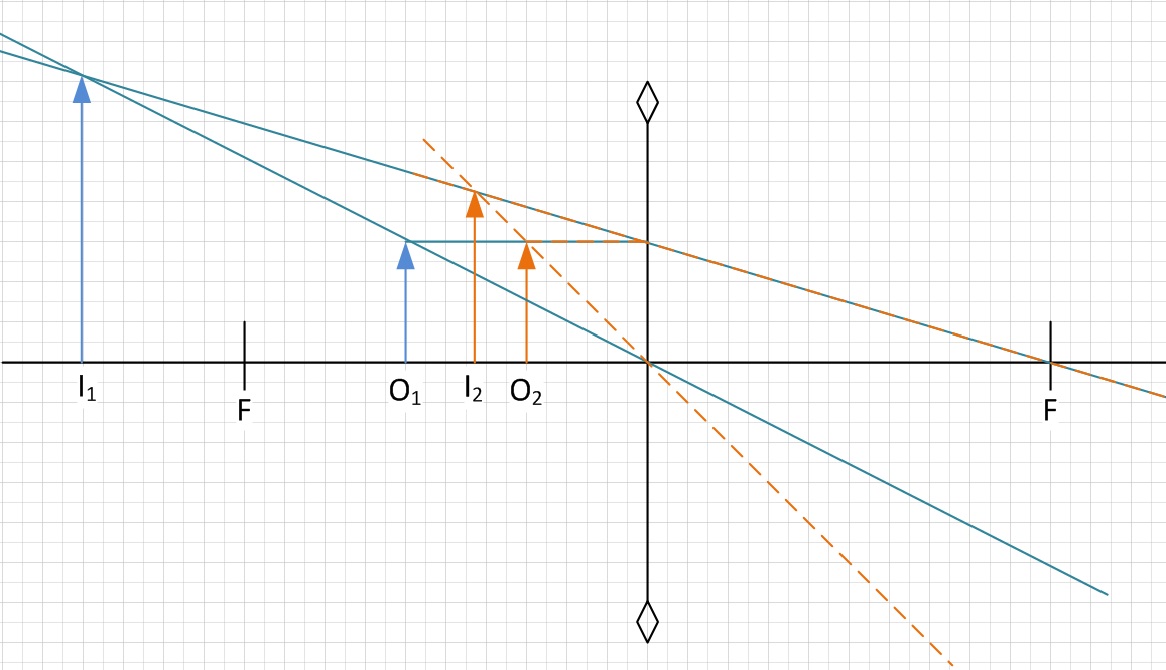
So the position of the virtual image as seen by the eye varies as the position of the object varies.
You might find using the Phet Geometrical Optics simulation informative?
Here are some screen shots:
No, it isn't, look at the ray diagram.
when the object is closer to the lens, the image is too..
If the image is too close the angle between the light rays is too great for a human eye to use to produce a retinal image. A near point of 25cm is assumed.
(Although for me it's more like 2.5 cm, but I am very short sighted.)
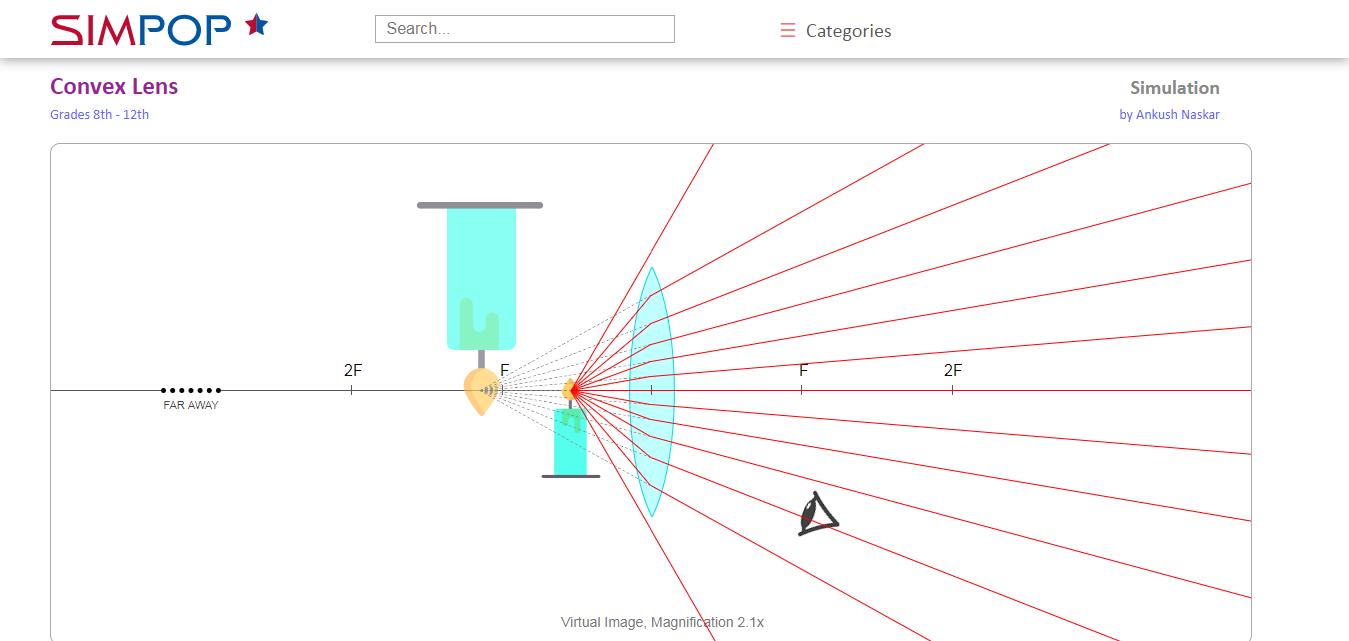
No, the distance from the virtual image and the magnification changes as the image is moved within the focal length. Try it yourself with my Convex Lens Simulation in html5.