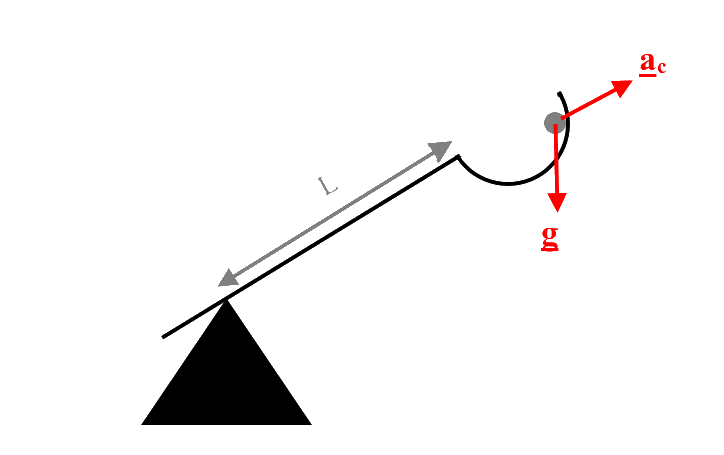
I am trying to study the motion of a point mass inside the bucket of a catapult.
The catapult is shooting downward (i.e. describing a rotation of 180° from the horizontal axis) and I would like to know whether the particle is leaving or not the bucket depending on L (the length of the arm) and the rotation speed of the catapult $\omega_c$.
Doing so I am trying to model the motion of a waterdrop inside a super-hydrophobic bucket. As a first model, I don't consider the friction (very low due to the coated surface), neither the rotational inertia of the waterdrop and I consider the norm of $a_c$ to be constant inside the bucket (because L is way bigger than the radius of the bucket). I only need an order of magnitude to design my experiment.
How would you do it? I was thinking about studying the bucket as fixed on the ground then computing different orientations of $a_c$ and $g$, like a "guided-surface" problem. However, I am afraid to skip the information of being in a rotational frame in this way.