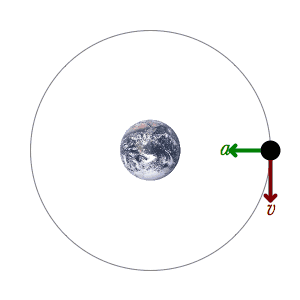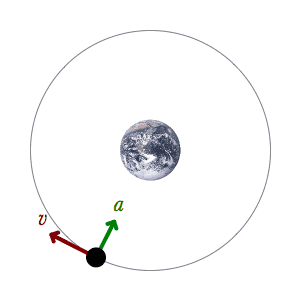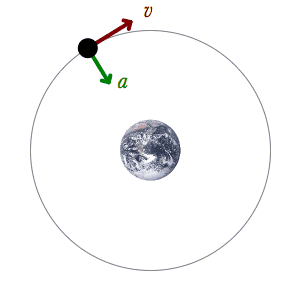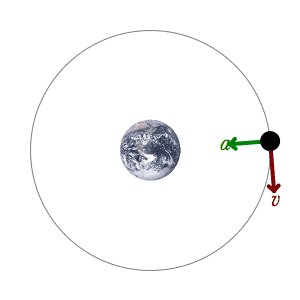
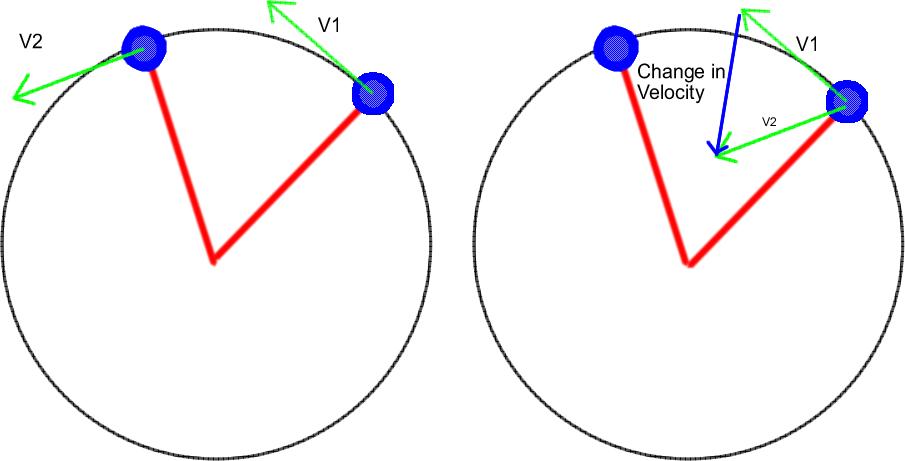
During uniform circular motion, the tangential acceleration is zero, but there is centripetal acceleration. Centripetal acceleration is a very necessary component of circular motion, but why does the object not move towards the center when the acceleration is towards center? Why does it move in a circle?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ Hi Danny, you need to read this about circular motion physicsclassroom.com/class/circles/Lesson-1/… $\endgroup$– user108787Commented Sep 12, 2016 at 16:55
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
Newton's first law states that an object that is in motion will remain in that motion going in a straight line unless acted by an unbalanced force.
So in case of a uniform circular motion we are essentially changing objects trajectory without changing its magnitude and that requires a force which is provided in this case by earths gravity. In the diagram below(Second picture) you can see that change in velocity(Blue vector) which is acceleration is pointing towards the center
Let's consider a different system: a ball moving in uniform circular motion by a string with a velocity $v$, When we observe the string it appears to be more stretched than it is when at rest, We also observe that faster the $v$ more stretched it is, This is because when an object has a larger velocity it is harder to change its direction of motion compared to when it has smaller velocity so because of this, string is stretched to provide more force through Tension. Mathematically we have to have force of tension equal to centripetal Force. Centripetal Force equation(proof of formula is explained very well in this question and also on khan academy):
$$F_c=m\frac{v^2}{r}$$
must equal to Force of Tension provided by string:
$$F=kx\Rightarrow$$
$$x=m\frac{v^2}{kr}$$
from equation we can see that more velocity and mass an object has more string has to stretch to keep it moving in a circular path.
why the object doesn't move towards the center
An objects centripetal acceleration is different when object has different $v$, More $v$ it has harder it is to change it's direction of motion, By saying above if you mean that "since there is an unbalanced force towards the center why doesn't it spiral down to center" It doesn't fall down to the center because centripetal force provided by Tension of a string is always the amount needed to only change the objects velocity direction, as a result it goes in a circular trajectory.
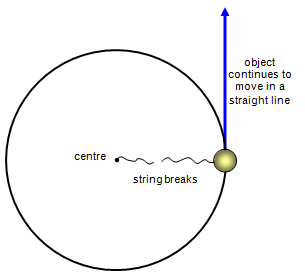
If a string broke there would no longer be this centripetal force to keep this object moving in a circular path so it will continue moving with that velocity $v$ in the direction that is tangential to the circle.
$\begingroup$
$\endgroup$
why the object doesn't move towards the center
IT DOES MOVE TOWARDS THE CENTRE. Every time the particle proceeds forward the centripetal force makes it fall by an equal amount towards it so essentially the particle is moving in a straight line but keeps falling towards the central force. The central force has no effect on the particles speed but instead it's the particle's speed that determines the amount of force needed to keep the particle in the circular orbit. This no effect causes the no change in tangential speed so the particle maintains constant velocity.
$\begingroup$
$\endgroup$
It is the magnitude by which the object needs to be curved, if centripetal acceleration is in fact also increasing due to some external factors(tightening of the string) but velocity is not increasing in proportion to the centripetal acceleration, i.e., at some instant, a > $mv^2/r$ It will spiral down the circular path as you picture in your head. when deriving the equations for circular motion, we treat radius(r) as a constant while differentiating, that is why centripetal acceleration for circular motion does not pull the object down wholly. While differentiating we tell Math that we need forces along tangential and radial directions that keep r constant.