My question is
Why voltage drops to zero after passing through a single resistance circuit?
Do charges slow down when passing through resistance and if they do then why isn't it effecting current?
How do charges carry on their motion even after voltage drops to zero?
The ideal circuit, as presented in introductory courses, simplifies the physics in order that the student not be distracted by too many details:
How do charges carry on their motion even after voltage drops to zero?
Resistance is present in the wires and connectors, as well as the internal resistance within the battery. Their is a slight, measurable, drop in voltage all along the path of the circuit. Thus the charges in the circuit will always be subject to a voltage.
Do charges slow down when passing through resistance and if they do then why isn't it effecting current?
Since the voltage varies from one point to the next, the potential energy has a spatial gradient; this is the origin of the electric field which is felt by the charges at each point along the circuit. Where the voltage drop is greater, the electric field is stronger.
Why voltage drops to zero after passing through a single resistance circuit?
In reality the voltage does not drop immediately to zero; however, in the ideal case the resistance of the wires is ignored - they aren't really there. In real circuits the wires and other connectors may play an important role in the design.
There is more to say about the physics of an electrical current: within any metal there exists typically one or two free electrons per atom, depending on the metal. These "free electrons" are shielded by the net positive charge of the atomic cores, and move "freely" throughout the metal. Drude called this the electron sea (1900), and this model is still studied in introductory condensed matter, though it has several defects which require quantum theory to repair.
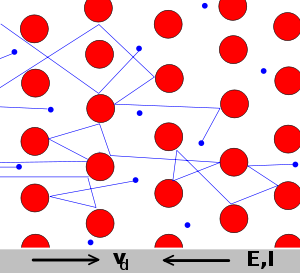
Drude's "electron sea" model
The "electron sea" thus consists of randomly moving electrons which are scattered off of defects and crystal boundaries. Drude assumed a statistical distribution of speeds similar to that which was so successful in the kinetic theory of gasses. The average thermal speed of a free electron in copper is about 1% of the speed of light; OTOH, the drift velocity of a typical current in the same wire is millimeters per second. See Hyperphysics-Microscopic Electric Current for calculations.
The physics tells us that though the current travels very slowly, the changes in the electric field travel at nearly the speed of light. This is usually covered in an upper level undergraduate course in electromagnetic field theory.
Why voltage drops to zero after passing through a single resistance circuit
Because one terminal of the resistance is connected to the battery's zero potential or ground.
Do charges slow down when passing through resistance and if they do then why isn't it effecting current?
Current depends on the no. of carriers passing through a cross-section. The number of charges in and out of the resistance remain the same. The resistance may slow the charges but doesn't absorb or alter the no. of charges.
How do charges carry on their motion even after voltage drops to zero
The battery emf is responsible for the uplift of charges from zero to battery potential $V$. The energy is provided by the chemical process in a normal battery cell.
Why voltage drops to zero after passing through a single resistance circuit?
The voltage is not the speed of these electrons. It is the push on them. For example, pushing something through water is much tougher than pushing something through air. You must push extra hard on the object in water if you want it to move just as fast.
The resistor resists electrons' motion. Like when you try to push water through a filter and must push harder to have more water moving through. The electrons are being resisted, so the push on them must be high for them to move through at some speed. Afterwards, there is no need for a push anymore; they can continue with the same speed they exited the resistor with without any more pushing.
Do charges slow down when passing through resistance and if they do then why isn't it effecting current?
No, they don't.
When the first electrons arrive they try to squeeze through but are resisted and slowed down. when the next arrive, they now must wait in line and must slow down to the same speed. The next arriving must do the same. Eventually, when you have a steady current, all charges move with same speed both before, throughout and after the resistor.
How do charges carry on their motion even after voltage drops to zero?
Remember that you do not need a force to move. You only need a force to change motion. If a spaceship just drifts at some speed, then it never stops. It continues effortless without any force acting on it.
Similarly, electrons must be pushed with a pushing force through the resistor to balance out the resisting force in the resistor. But afterwards, there is no resisting force on the electrons (assuming a perfectly conducting wire), so there is no more pushing force (voltage) needed to keep them moving.
