Consider a uniformly horizontally accelerated tube of water. I know that the fluid experiences a pseudo force in addition to its own weight, so that it reaches equilibrium in the below diagram.
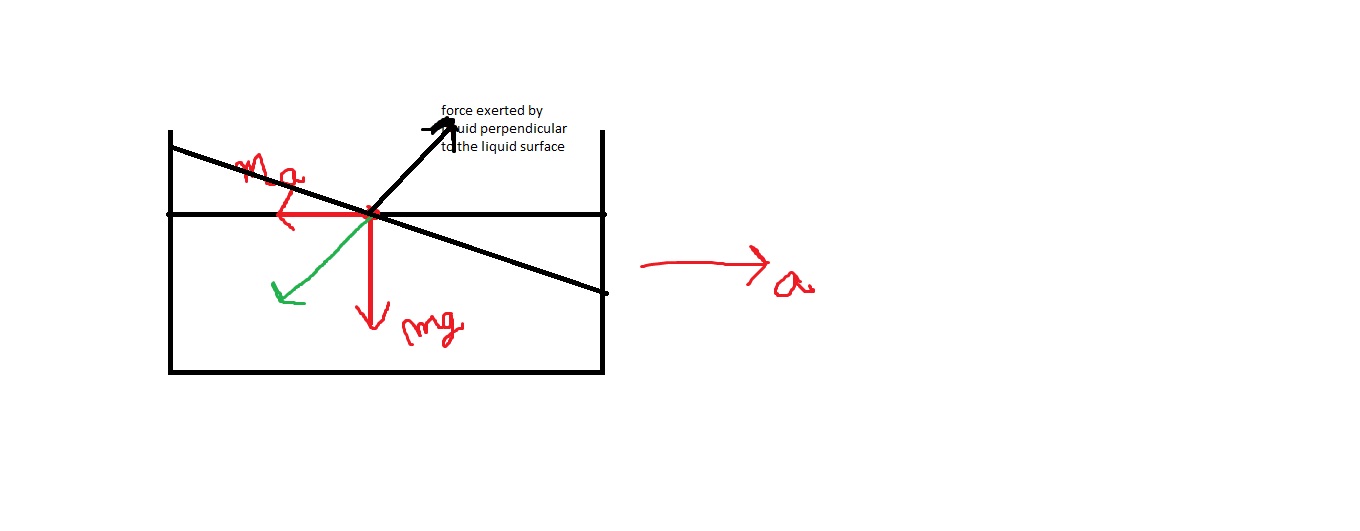But why can't the water also exert a force like this, so it can be in equilibrium horizontally?
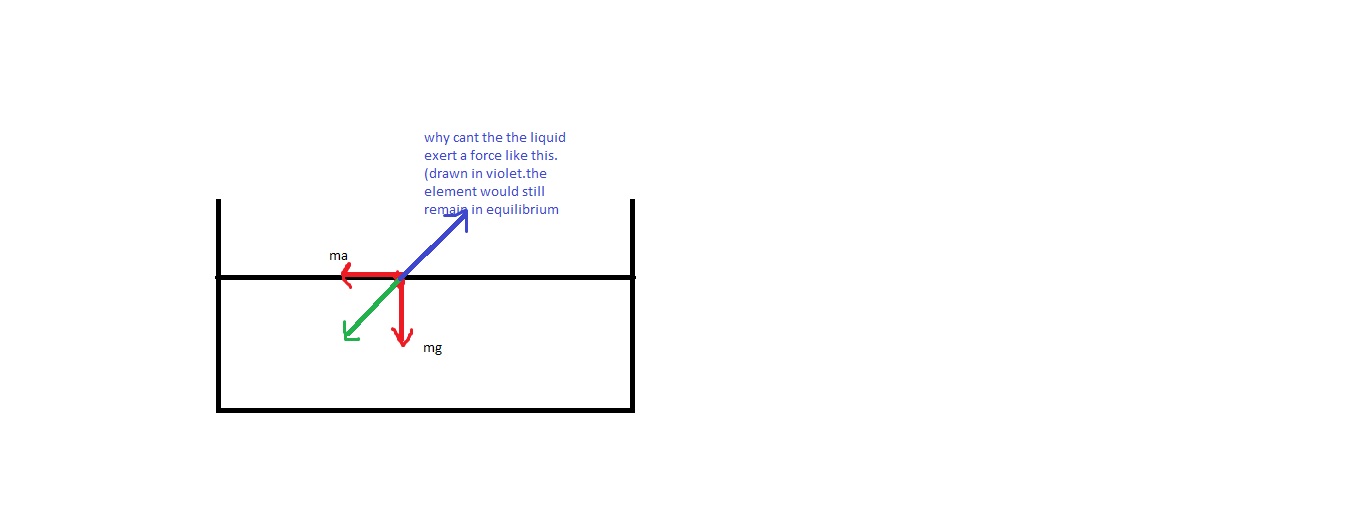
$\begingroup$
$\endgroup$
1
asked Aug 7, 2016 at 12:17
-
$\begingroup$ The surface of the liquid is going to be perpendicular to the total acceleration, including gravity. If you have a cup sitting on a table then the only acceleration is gravity, and the surface is perpendicular to it. When accelerating the cup from a different direction, the total acceleration vector is going to be slanted. The new "normal" vector represented by the surface will also be slanted. $\endgroup$– Sponge BobCommented Aug 8, 2016 at 18:58
Add a comment
|
5 Answers
$\begingroup$
$\endgroup$
The reason that the second diagram you drew cannot represent what is happening is that it will not satisfy Newton's 2nd law for all parcels of fluid in the tank. Imagine that you had a tank like the one shown in the diagrams and, rather than accelerating it, you just tilt it at an angle so that base is no longer horizontal. Basically, what you ave done is change the direction of gravity relative to the sides of the container. Would you expect the water surface to remain parallel to the base of the container, or would you expect it to be horizontal again (but tilted relative to the base). What you have done in the acceleration experiments is to add a pseudo-gravitational force component in the direction opposite to the acceleration. So now, the effective gravity is no longer pointing in the vertical direction. Thus, the surface of the fluid must readjust to again be perpendicular to the new effective gravitational direction (which is not vertical).
If you do a force balance on a small parcel of fluid within the system having sides dx, dy, and dz, the force balance in the y (vertical) direction reduces to:$$\frac{\partial p}{\partial y}=-\rho g$$The force balance in the x (horizontal) direction reduces to:$$\frac{\partial p}{\partial x}=-\rho a$$where a is the acceleration. The variation of pressure with position is given by: $$dp=\frac{\partial p}{\partial x}dx+\frac{\partial p}{\partial y}dy=-\rho adx-\rho g dy$$ It follows from this that the surfaces of constant pressure are given by:$$\frac{dy}{dx}=-\frac{a}{g}$$ The free surface is a contour of constant pressure.
answered Aug 8, 2016 at 2:46
$\begingroup$
$\endgroup$
For the sake of simplicity, let's assume it's a cubical container of water. The concept remains the same.
Look at the "free body diagram" of the water itself. As you noted, one side of the water is higher than the other. The surface is slanted.
The water is accelerating, so we know that there must be a net horizontal force acting on it. We also know that the only horizontal forces that are possible in our case are due to the pressure forces acting on the water via $P=\gamma*H$.
The only way the water can accelerate is if the force on one side is greater than the force on the other side. The only way this can happen is if the pressure on one side is greater than the pressure on the other side. The only way this can happen is if one side of the water is higher than the other. Again, $P=\gamma*H$.
The forces acting on the water in the $x-direction$ can be derived using calculus.
$\begingroup$
$\endgroup$
The reason your proposed force can't work is because water is a fluid.
Solids are locked into place, so they can both resist compression and resist shearing (the sideways force in your diagram). Fluids can only resist compression; they cannot resist a sideways shearing. This is precisely what makes fluids flow in the first place.
$\begingroup$
$\endgroup$
Slice the water in the latter case into layers that are perpendicular to the net force. All the layers in this case would be squeezed by the layers of water above them, so they would expand until they could no longer do so - in other words, when each layer extends from left wall of the container to the right wall (or the bottom, if the acceleration is high enough). This case would look like your first picture.
A more mathematical explanation would be that the slanted case is the configuration with the lowest potential energy. But proving that should involve some tedious steps, especially if you assume that the water could be in any shape in the end.
$\begingroup$
$\endgroup$
Imagine yourself inside a car at rest with 10 cm deep water inside it. Label the surface level as 0.
While stopped the water level is at rest, i.e. parallel to the Earth surface.
Next you accelerate the car motor:
The mass of the water is not rigid (is not ice) and it is not firmly linked to the car.
Move ahead the car a little - the water mass will lag a little because the motion could not be transmitted at once to it, without delay. Then:
The front of the car will present a water deficit (imagine a hole between the car and the water). Next moment - the water will fall into that hole and the surface level is negative.
In the rear of the car we have an accumulation of the initial water plus the water that is not present in the front of it - Then the water level will have to be positive; higher then the level 0.
The inertia law, aka the 1st Newton law, is at work in relation to the liquid mass and is often stated as - An object at rest stays at rest and an object in motion stays in motion with the same speed and in the same direction unless acted upon by an unbalanced force.
The water level will be balanced to 0 after the acceleration stopped, whatever the velocity.
