5
$\begingroup$

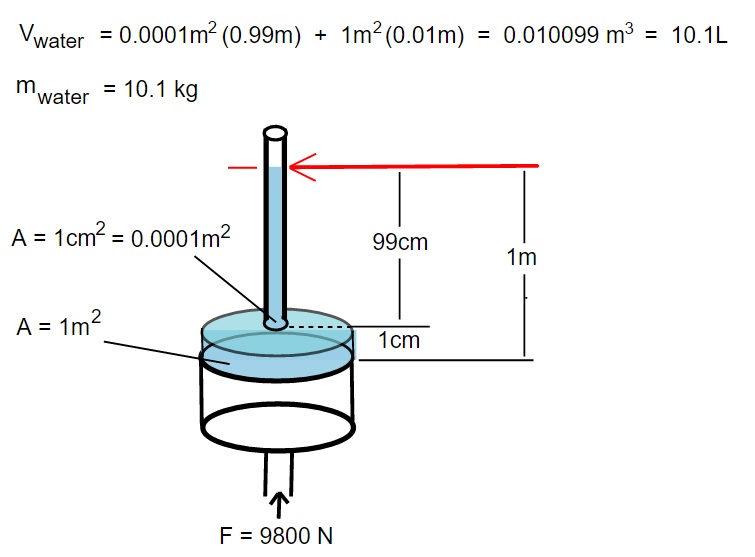
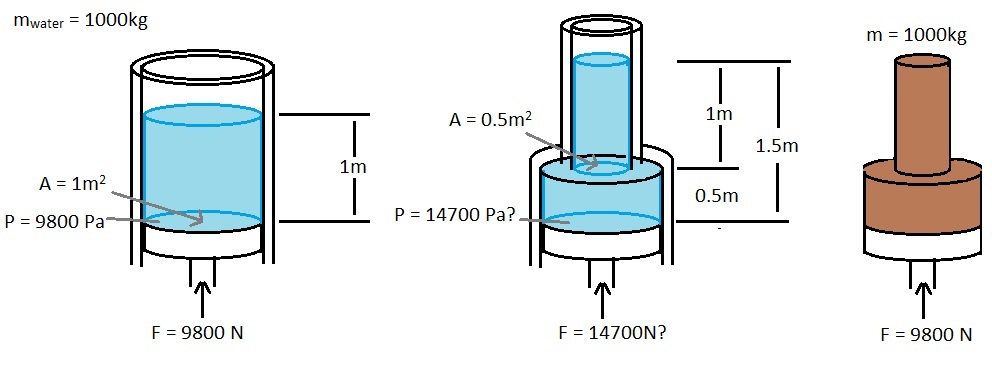
We know that the pressure in a fluid (like water) is dependent on the depth. Consider this example: enter image description here
For the first setup, solving the pressure at the bottom yields
$P_1 = \rho gh = 1000kg/m^3 \bullet 9.8m/s \bullet 1m = 9800Pa$
And from the definition of pressure, $P = F/A$, the fluid exerts a force on the piston equivalent to:
$F_1 = P_1A = 9800Pa\bullet 1m^2 = 9800N$
so the piston will exert a force of $9800N$ to maintain equilibrium.
Which should be reasonable since this is the actual weight of the water:
$W_{water}= 1000kg \bullet 9.8m/s^2 = 9800N$

For the second setup, solving the pressure at the bottom yields
$P_2 = \rho gh = 1000kg/m^3 \bullet 9.8m/s \bullet 1.5m = 14700Pa$
And
$F_2 = P_2A = 14700Pa\bullet 1m^2 = 14700N$
so the piston will exert a force of $14700N$ to maintain equilibrium.

Note that the water at each setup occupy the same amount of volume.

The question is: Is this assumption correct? If so, then how do the forces and pressures in the water arrange in such a way that it now requires more force to balance the water than its original weight? And how is setup 2 different from setup 3, where you have a solid mass of the same shape and weight?

Improve this question
$\endgroup$

4 Answers 4

Reset to default
9
$\begingroup$

Your analysis and intuition are correct. The force needed in the second setup is larger, even though the weight of the water is the same.

To understand why, consider the horizontal part of the container, $0.5\ \text{m}$ off the ground. This wall is above the water, so the water's pressure pushes up on it. Then in reaction, the wall pushes down on the water, providing the extra force you calculated.

In the third setup, there is no wall to provide an extra force, so the force on the piston is just the weight of the mass.

Improve this answer
$\endgroup$
1
$\begingroup$

Pascal's law:

Pascal's law or the principle of transmission of fluid-pressure (also Pascal's Principle) is a principle in fluid mechanics that states that pressure exerted anywhere in a confined incompressible fluid is transmitted equally in all directions throughout the fluid such that the pressure variations (initial differences) remain the same.

Due to transmission of pressure at the bottom of the middle piston the pressure ($P_2$) is indeed $14700\:\mathrm{Pa}$.

But left and right, the weight of the water is always $1000\:\mathrm{kg} \times 9.8\:\mathrm{m s^{-2}} = 9800\:\mathrm{N}$.

Improve this answer
$\endgroup$
6
  • $\begingroup$ does this mean that a balance cannot measure the weight of a liquid? $\endgroup$
    – user65081
    Commented Jun 17, 2016 at 22:15
  • $\begingroup$ @Wolphramjonny: there's difference between putting a 1000 kg in a container on one of the balance's platforms and using one of the balance's platforms as a frictionless piston in the OPs set ups. In the latter case the balance would act as a manometer. $\endgroup$
    – Gert
    Commented Jun 17, 2016 at 22:19
  • $\begingroup$ what I was thinking is to use a spring with a gauge instead of a force in the bottom of the piston, and also imagine cutting a small slice of piston at the top which will become the bottom of the container. What makes this modified setup different than a balance? $\endgroup$
    – user65081
    Commented Jun 17, 2016 at 22:22
  • $\begingroup$ Hmmm... I think a diagram is in order. If you think it might shed light on the question, why not formulate an answer? :-) $\endgroup$
    – Gert
    Commented Jun 17, 2016 at 22:27
  • 1
    $\begingroup$ That's correct. It's like when you immerse an object on a stick and held steady: the balance's reading increases by the buoyant force. Great trick for determining densities. $\endgroup$
    – Gert
    Commented Jun 17, 2016 at 22:33
1
$\begingroup$

In addition to @knzhou's fine answer, I'd like to present a more extreme example, which will hopefully provide some more insight!

enter image description here


Solving the pressure at the bottom yields:
$P = \rho gh = 1000kg/m^3 \bullet 9.8m/s \bullet 1m = 9800Pa$

And pressure, $P = F/A$, the fluid exerts a force on the piston equivalent to:
$F = P \bullet A = 9800Pa\bullet 1m^2 = 9800N$

And the piston must exert the equal, opposite force of $9800N$ in order to maintain the height of the water at the red $1m$ mark! Of course, this is equivalent to 2203 lb (to maintain that precise equilibrium)!

And the total weight of the water is only:
$W_{water}= 10.1kg \bullet 9.8m/s^2 = 99N$, which is 22.3 lb!

Improve this answer
$\endgroup$
-2
$\begingroup$

The quick answer is that a long as the surface area of the piston, and the volume/mass of the remain unchanged, the pressure stayed the same.

So the erreanous step is to assume that $ P_2 = \rho g h $. This invalid as the cross section area changes with height.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ $P_2=\rho g h$ is correct. The pressure at the bottom of a container is independent of the shape of the container. Only the depth of fluid and its density matter. $\endgroup$
    – Gert
    Commented Jun 17, 2016 at 22:07
  • 1
    $\begingroup$ Cool. If that's the case i definitely have to revisit my mechanics skills $\endgroup$
    – Mikael Fremling
    Commented Jun 18, 2016 at 7:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.