There seems to be something wrong with the answer.
My explanation is intuitive and quite long but worth it
Charges outside spherical conductor cannot induce electric field inside a electrically isolated conductor due to electrostatic shielding
Electrostatic Shielding-a region enclosed by meat of a conductor will not experience any electric field generated by charges placed outside the enclosure
So the field at the center is only due to the charges inside the shell and the inner surface.
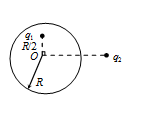
NOTE:HERE WE CONSIDER $q_{2}$ IS NOT PRESENT
To understand this better consider a spherical hollow shell with a charge $q_{1}$ kept anywhere in it.we know that that this charge $q_{1}$ will induce a $-q_{1}$ on the inner surface of the shell and a charge $q_{1}$ on the outer surface of the shell.
On the inner surface of the shell the region close to the charge will have more induced charge density and region farther away from the charge will have less induced charge density.
The distribution on the induced charge on the outer surface of the shell is going to be spherically symmetric because there is no force acting on it,there is no electric field inside the meat of and electrically isolated conductor and outer induced charges are inside the conductor and will arranged themselves is such a way that the have minimum potential energy among them (due to repulsion),that is in a spherically symmetric manner
To find electric field at the center of the shell
Due to the charge induced on the inner surface of the shell
region of high charge density are closer to $q_{1}$ but far away from center which balances the fraction $\frac{q}{r}\propto field$
region of low charge density are far away from $q_{1}$ but closer to center which balances the fraction $\frac{q}{r}\propto field$
So in net effect all the charges on the inner surface of the shell will produce (approx.)the same field at the center and will produce zero electric field.
Due to the charge induced on the outer surface of the shell
Outer charges are symmetrically distributed and will not produce any field inside the shell hence field due to outer charge zero.
- Due to $q_{1}$
$$\frac{1}{4\pi\epsilon_{0}}\frac{q}{({\frac{r}{2}})^{2}}$$
Net electric field =(electric field due to the charge induced on the outer surface of the shell) + (electric field due to the charge induced on the outer surface of the shell)+(due to q1)
=0+0+$\frac{1}{4\pi\epsilon_{0}}\frac{q}{({\frac{r}{2}})^{2}}$ = $\frac{1}{4\pi\epsilon_{0}}\frac{q}{({\frac{r}{2}})^{2}}$
Now comes the most beautiful part
Consider a charge $q_{2}$ to be placed outside the shell.
It's effect on the outer surface of shell.
- it messes up the charge distribution
It's effect on the inner surface of shell.
- None.As long as there is the meat of the conductor protecting it experiences no electric field by $q_{2}$ hence no force by $q_{2}$(electrostatic shielding)
It's effect on electric field at the center
None. Surrounded by meat of the conductor - electrostatic shielding.
effect of the messed up charged distribution on the outside surface of the conductor on the electric field inside the conductor
None.electrostatic shielding
So electric field at the center
$$\frac{1}{4\pi\epsilon_{0}}\frac{q}{({\frac{r}{2}})^{2}}$$
Correction to this answers are welcomed