Working out shaft torque in a stirred tank with a cylindrical cavern formation (using a non-Newtonian fluid)

Question

Here is some background to the problem (in a stirred tank):

"With yield stress non-Newtonian (viscoplastic) fluids, it is possible to generate an agitated volume around the impeller, defined as a cavern, surrounded by a stagnant region where the shear stress is insufficient to overcome the apparent yield stress of the fluid."

Sometimes you can get a cylindrical cavern around the impeller, see the below image.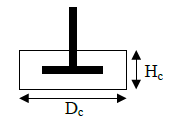

"By performing a force balance between the applied torque, Γ and the shear stress acting on the surface of a cylinder, we can define the boundary by setting the shear stress equal to the yield stress τ = τY. The total torque is given by: $$\Gamma = \frac{\pi}{2} \tau_{y}H_{C}D_{C}^2+\frac{\pi}{6}\tau_{y}D_{C}^3$$

I just can't get the second term. The first term I can get by doing: $$\Gamma_{1}=\tau_y \cdot Area_{Curved} \cdot \frac{D}{2} = \pi \cdot \frac{D^2}{2} \cdot H_{c} \cdot \tau_{y}$$

This gets me the first term...but the second term I just can't get, this is what I'm doing:

$$\Gamma_{2}=\tau_{y} \cdot Area_{Faces} \cdot \frac{D}{2} =\tau_{y} \cdot 2 \pi \cdot \frac{D^2}{4} \cdot \frac{D}{2} = \tau_{y} \cdot \pi \cdot \frac{D^3}{4} $$

Argh, so I'm getting D^2/4 instead of D^2/6 for the second term and I just can't work it out, if anyone can help I'd appreciate it. Thanks.

MIH.

Answer 1

The first term derives the torque for the curved surfaces and the second term derives the torque for the top and bottom of the cylindrical surface.

I don't really know why but apparently the cross sectional area at the top keep changing as $r\rightarrow R$ where $R=Dc/2$.

so $dA=\pi(r+dr)^2-\pi r^2$

$$dA=2\pi r dr+\pi.dr^2$$

I also don't understand why but apparently $dr^2$ is so small it can be assumed to be negligible. Anyways therefore $$dA=2\pi r dr$$

now $dΓ/r=τydA$,

$$dΓ=τy 2\pi r^2 dr$$

Integrating for $0$ to $Γ$ and $0$ to $R$ gives

$$Γ=\frac 23 τy\pi R^3$$

Since $R=Dc/2$

$$Γ=\frac 23 τy\pi Dc^3 \frac 18$$

$$Γ=\frac{1}{12}τy\pi Dc^3$$

This also applies for bottom and therefore adding these two gives

$$Γ=\frac 16τy\pi Dc^3$$

Sorry for the bad format but allow me XD