3
$\begingroup$

Problem

Definition

As shown in the figure below, an automobile is travelling horizontally in the rain with a constant velocity V. The windshield has a slope of θ. A raindrop with a mass of m, falling vertically with velocity v hits the windshield. Force is applied only perpendicularly to the windshield and the raindrop starts sliding on its surface. Any effect caused by air is ignored.

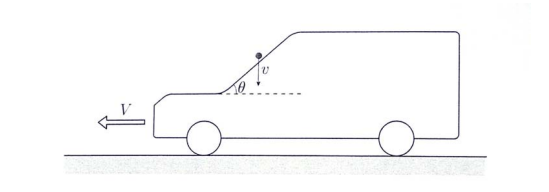
Figure

Question

What is the minimum ratio of V/v so that the raindrop starts sliding upwards? Provided possible answers are: sinθ; cosθ; tanθ; 1/sinθ; 1/cosθ; 1/tanθ.

EDIT 2: Greg Petersen and drvm's answers pretty much sum it all up. The trick is to treat the car as stationary and the rain drop as having a horizontal (V) and vertical (v) velocity. The vector formed when adding V and v should be at an angle smaller than 90 deg (from the windshield) which gives tanθ as the answer.

EDIT: Below is my view on how I thought things were supposed to happen, but that doesn't take into account the fact that air resistance (i.e. wind) is ignored by definition

If I have to be honest, I really have no idea what I'm supposed to do here. The way I see this, the raindrop moving upwards can only be caused by air resistance. Gravity can be broken down into two vectors, F_w which is parallel to the windshield and F_n which is perpendicular to it. This way F_n is cancelled out by N, which is the normal reaction force. This way, air resistance, which would also be parallel to the ground, would, if the car was moving fast enough, eventually cancel out F_w and the raindrop would start moving upwards.

This however cannot work, since the problem definition clearly states that anything air-related is ignored (which I presume includes air resistance as well). Another thing I can't really understand is the statement "Force is applied only perpendicularly to the windshield" - how is this possible if the raindrop is falling vertically downwards?

I'm not looking for an answer to this specific problem, but rather an explanation of what is going on and how I'm supposed to approach it. I've learned about motion (velocity and acceleration), forces and Newton's laws in school. but none of those seem to work here (or at least not the way I see it). Any help will be most highly appreciated!

Improve this question
$\endgroup$
5
  • $\begingroup$ Gravity applies a downward force and the wind applies an upward force based on the angle of the windshield. The question put simply is, which force is greater? Gravity pulling it down or the wind pushing it up? Once you know that, you can find the relationship between the two. $\endgroup$
    – Neil
    Commented Mar 22, 2016 at 15:46
  • $\begingroup$ @Neil The problem here states that effects caused by air are ignored, which I think includes wind as well. $\endgroup$
    – Stealthmate
    Commented Mar 22, 2016 at 16:20
  • $\begingroup$ Surface pressure of the water-glas interface simplified to friction is neglected? $\endgroup$
    – Stefan Bischof
    Commented Mar 22, 2016 at 16:25
  • $\begingroup$ @StefanBischof If I'm understanding that correctly, it should not be neglected, but how would you calculate pressure if you don't have any area anyway? $\endgroup$
    – Stealthmate
    Commented Mar 22, 2016 at 16:34
  • $\begingroup$ @Stealthmate Surface tension of water-glass surface determines contact angle to your windshield. Cleaning solvents will change this contact angle and area of contact. $\endgroup$
    – Stefan Bischof
    Commented Apr 19, 2016 at 13:51

2 Answers 2

Reset to default
2
$\begingroup$

The key phrase is "starts sliding upwards". I think they are asking what is the initial velocity of the raindrop (i.e. at t=0). If this is the case, you just need to balance the velocity of the drop due to falling vs the movement of the car. It may be helpful the consider the car at rest, and the drop moving to the right with the velocity of the car.

I would ask for clarification on the problem before submitting just to be safe though.

Improve this answer
$\endgroup$
8
  • $\begingroup$ I tried drawing a force diagram, but I can't find a connection between the two velocities and the forces acting on the rain drop. $\endgroup$
    – Stealthmate
    Commented Mar 22, 2016 at 16:22
  • $\begingroup$ Hmm you know after reading more closely, I think this is easier than I first thought. The key phrase is "starts sliding upwards". I think they are asking what is the initial velocity of the raindrop (i.e. at t=0). If this is the case, you just need to balance the velocity of the drop due to falling vs the movement of the car. It may be helpful the consider the car at rest, and the drop moving to the right with the velocity of the car. $\endgroup$
    – Greg Petersen
    Commented Mar 22, 2016 at 16:51
  • $\begingroup$ Is this the first part of a multi part question? $\endgroup$
    – Greg Petersen
    Commented Mar 22, 2016 at 16:52
  • $\begingroup$ Ok, I did that and found that V/v >= tanθ. Here is how it turned out. $\endgroup$
    – Stealthmate
    Commented Mar 22, 2016 at 17:21
  • $\begingroup$ Also yes, there is a second part to this question, which is to find the impulse of the rain drop acting on the windshield, but if this is the correct solution, then I pretty much know how it will turn out. $\endgroup$
    – Stealthmate
    Commented Mar 22, 2016 at 17:23
2
$\begingroup$

As its a homework exercise I can not do justice- but I can just point out that at certain velocity ratio the rain drops will start moving up the wind shield.

One can take the car as stationary and add a car's velocity vector V to rain drops vertically down velocity v- the resultant will be inclined to the vertical say at an angle $\theta$ and if it has some component sliding along the inclined wind shield it can happen(the droplets will slide up).

When we run on a rainy day(rain falling vertically downward) with an umbrella -the umbrella has to be tilted from the vertical to save us from rain drops and as we speed up this tilt angle has to be increased.

Improve this answer
$\endgroup$
4
  • $\begingroup$ We can post answers with capitalized "I"'s and the first word of sentences capitalized, can't we? This wouldn't be acceptable in a report to give to your boss and it isn't acceptable here either. $\endgroup$
    – Neil
    Commented Mar 23, 2016 at 7:38
  • $\begingroup$ sorry , i will edit it $\endgroup$
    – drvrm
    Commented Mar 23, 2016 at 11:04
  • $\begingroup$ Better. I'll remove the downvote. $\endgroup$
    – Neil
    Commented Mar 23, 2016 at 11:24
  • $\begingroup$ thanks for the gesture, i can add about the forces but again it is a homework and the hint should be sufficient. $\endgroup$
    – drvrm
    Commented Mar 23, 2016 at 11:29

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.